MCQ Chapter 2 Polynomials Class 10 Mathematics

Please refer to Polynomials MCQ Questions Class 10 Mathematics below. These MCQ questions for Class 10 Mathematics with answers have been designed as per the latest NCERT, CBSE books, and syllabus issued for the current academic year. These objective questions for Polynomials will help you to prepare for the exams and get more marks.

Polynomials MCQ Questions Class 10 Mathematics

Please see solved MCQ Questions for Polynomials in Class 10 Mathematics. All questions and answers have been prepared by expert faculty of standard 10 based on the latest examination guidelines.

MCQ Questions Class 10 Mathematics Polynomials

Question. If α,β are the zeros of the polynomial x² − px + q, then α²/β² +β²/α² is equal to −
(a) p⁴/q² +2 −4p²/q
(b) p⁴/q² −2 +4p²/q
(c) p⁴/q²+2q² −4p²/q
(d) None of these

Question. If a,b are the zeros of the polynomial x² − px + 36 and α² + β² = 9, then p =
(a) ± 6
(b) ± 3
(c) ± 8
(d) ± 9

Question. If α,β are zeros of ax² + bx + c, ac ≠ 0, then zeros of cx² + bx + a are −
(a) − α − β
(b) α,1/ β
(c) β,1/ α
(d) 1/α,1 /β

Question. If x − 3 is a factor of x³ + 3x² + 3x + p, then the value of p is
(a) 0
(b) −63
(c) 10
(d) None

Question. If 2 and 3 are the zeros of f(x) = 2x³ + mx² − 13x + n, then the values of m and n are respectively −
(a) −5, − 30
(b) −5, 30
(c) 5, 30
(d) 5, − 30

Question. If a,b are the zeros of the polynomial 6x² + 6px + p², then the polynomial whose zeros are (α + β)² and (α −β)² is −
(a) 3x² + 4p²x + p⁴
(b) 3x² + 4p²x − p⁴
(c) 3x² − 4p²x + p⁴
(d) None of these

Question. The value of ax² + bx + c when x = 0 is 6. The remainder when dividing by x + 1 is 6. The remainderwhen dividing by x + 2 is 8. Then the sum of a, b and c is
(a) 0
(b) −1
(c) 10
(d) None

Question. If the G.C.D. of the polynomials x³ − 3x² + px + 24 and x² −7x + q is (x − 2), then the value of (p + q) is:
(a) 0
(b) 20
(c) −20
(d) 40

Question. The remainder of x⁴ + x³ − x² + 2x + 3 when divided by x − 3 is
(a) 105
(b) 108
(c) 10
(d) None

Question. If the L.C.M. of two polynomials p(x) and q(x) is (x + 3)(x − 2)²(x − 6) and their H.C.F. is (x − 2).If p(x) = (x + 3)(x − 2)², then q(x) =________
(a) (x + 3)((x − 2) 
(b) x2 − 3x − 18
(c) x2 − 8x + 12
(d) none of these

Question. If α,β,γ are the zeros of the polynomial x³ + 4x + 1, then (α + β)⁻¹ + (β + γ)⁻¹ + (γ + α)⁻¹ =
(a) 2
(b) 3
(c) 4
(d) 5

Question. If α,β are the roots of ax² + bx + c and a + k, b + k are the roots of px² + qx + r, then k =
(a) −1/2[a/b−p/q]
(b) [a/b−p/q]
(c) 1/2[b/a−q/p]
(d) (ab − pq)

Question. The condition that x³ − ax² + bx − c = 0 may have two of the roots equal to each other but of opposite signs is :
(a) ab = c
(b) 2/3,a
(c) a²b = c
(d) None

Question. The remainder when x1999 is divided by x² − 1 is
(a) − x
(b) 3x
(c) x
(d) None

Question. For the expression f(x) = x³ + ax² + bx + c, if f(a) = f(b) = 0 and f(d) = f(0). The values of a, b & c are
(a) a = − 9, b = 20, c = − 12
(b) a = 9, b = 20, c = 12
(c) a = − 1, b = 2, c = − 3
(d) None of these

Question. If x² − ax + b = 0 and x² − px + q = 0 have a root in common and the second equation has equal roots, then
(a) b + q = 2ap
(b) b + q = ap /2
(c) b + q = ap
(d) None of these

Question. If f(x) = ax² + bx + c is divided by (bx + c), then the remainder is_____.
(a) c²/b²
(b) ac²/b² + 2c
(c) f(−c/b)
(d) ac² +2b²c/b²

Question. ax⁴ + bx³ + cx² + dx + e is exactly divisible by x² − 1, when:
(a) a + b + c + e = 0
(b) a + c + e = 0
(c) a + b = 0
(d) a + c + e = b + d = 1

Question. If x + 1 is a factor of ax⁴ + bx³ + cx² + dx + e = 0 then ____
(a) a + c + e = b + d
(b) a + b = c + d
(c) a + b + c + d + e = 0
(d) a + c + b = d + e

Question. If a³ −3a²b + 3ab² − b³ is divided by (a − b), then the remainder is
(a) a² − ab + b²
(b) a² + ab + b²
(c) 1
(d) 0

Question. xⁿ − yⁿ is divisible by x + y, when n is_______.
(a) An odd positive integer
(b) An even positive integer
(c) An integer
(d) None of these

Question. If α,β are the zeros of the quadratic polynomial 4x² − 4x + 1, then α³ + β³ is −
(a) 1/ 4
(b) 1/ 8
(c) 16
(d) 32

Question. If a + b = 4 and a³ + b³ = 44, then a,b are the zeros of the polynomial.
(a) 2x² − 7x + 6
(b) 3x² + 9x + 11
(c) 9x² − 27x + 20
(d) 3x² − 12x + 5

Question. If the sum of zeros of the polynomial p(x) = kx³ − 5x² − 11x − 3 is 2, then k is equal to
(a) k = − 5/ 2
(b) k = 2/ 5
(c) k = 10
(d) k = 5/ 2

Question. If y = f(x) = mx + c; then f(y) in terms of x is
(a) mx + m + c
(b) m + mc + c
(c) m²x + mc + c
(d) m²x + m²c

Question. If 7 + 3x is a factor of 3x³ + 7x, then the remainder is
(a) 490/ 9
(b) −490/ 9
(c) 470/ 9
(d) None

Question. The remainder when f(x) = 3x⁴ + 2x³ −x2/3 −x/9 + 2/27 is divided by g(x) = x + 2/ 3 is
(a) −1
(b) 1
(c) 0
(d) −2

Question. f(x) = 3x⁵ + 11×4 + 90x² − 19x + 53 is divided by x + 5 then the remainder is ______.
(a) 100 
(b) −100
(c) −102
(d) 102

Question. If f(x) = 4x³ − 6x² + 5x − 1 and α,β and γ are its zeros, then αβγ =
(a) 3/ 2
(b) 5/ 4
(c) −3/2
(d) 1/ 4

Question. Consider f(x) = 8x⁴ − 2x² + 6x − 5 and a,b,g,d are it’s zeros then α + β + γ + δ =
(a) 1/ 4
(b) − 1/ 4
(c) −3/2
(d) None

Question. If (x − 3), (x −3) are factors of x³ − 4x² − 3x + 18; then the other factor is
(a) x + 2
(b) x + 3
(c) x − 2
(d) x + 6

Question. The remainder when 1 + x + x² + x³ + ……….+ x2006 is divided by x− 1 is
(a) 2005
(b) 2006
(c) 2007
(d) 2008

Question. If (x − 1), (x + 1) and (x − 2) are factors of x⁴ + (p − 3)x³ − (3p − 5)x² + (2p − 9) x + 6 then the value of p is
(a) 1
(b) 2
(c) 3
(d) 4

Question. If f (−3/4)=0; then for f(x), which of the following is a factor?
(a) 3x − 4
(b) 4x + 3
(c) −3x + 4
(d) 4x − 3

Question. If the remainder when the polynomial f(x) is divided by x − 1, x + 1 are 6, 8 respectively then the remainder when f(x) is divided by (x − 1)(x + 1) is
(a) 7 − x
(b) 7 + x
(c) 8 − x
(d) 8 + x

Question. If a, b and g are the zeros of the polynomial f(x) = x³ + px² − pqrx + r, then 1/αβ + 1/βγ +1/γα =
(a) r/p
(b) p/ r
(c) − p/ r
(d) − r/ p

Question. The coefficient of x in x² + px + q was taken as 17 in place of 13 and it’s zeros were found to be − 2 and − 15. The zeros of the original polynomial are
(a) 3, 7
(b) − 3, 7
(c) − 3, − 7
(d) −3, − 10

Question. Let a, b be the zeros of the polynomial x² − px + r and ,α/2, 2β be the zeros of x²− qx + r. Then the value of r is −
(a) 2/9 (p−q)(2q−p) 
(b) 2/9 (q−p)(2p−q) 
(c) 2/9 (q −2p)(2q−p) 
(d) 2/9(2p−q)(2q−p)

Question. When x200 + 1 is divided by x² + 1, the remainder is equal to −
(a) x + 2
(b) 2x − 1
(c) 2
(d) − 1

Question. If a (p + q)² + 2bpq + c = 0 and also a(q + r)² + 2bqr + c = 0 then pr is equal to −
(a) p² + a/ c
(b) q² +c/a
(c) p² +q/b
(d) q² +a/c

Question. If α, β and γ are the zeros of the polynomial f(x) = ax³ + bx² + cx + d, then 1/α +1/β +1/γ =
(a) −b/ a
(b) c/ d
(c) −c/d
(d) −c / a

Question. If a, b and g are the zeros of the polynomial f(x) = ax³ − bx² + cx − d, then α² +β² + γ² =
(a) b² −ac/a²
(b) b² +2ac/b²
(c) b² −2ac/a
(d) b² −2ac/a²

Question. If a,b and c are not all equal and a and b be the zeros of the polynomial ax² + bx + c, then value of (1 + a + a²) (1 + b + b²) is :
(a) 0
(b) positive
(c) negative
(d) non-negative

Question. f(x) = 16x² + 51x + 35 then one of the factors of f(x) is
(a) x − 1
(b) x + 3
(c) x − 3
(d) x + 1

Question. Find the remainder obtained when x2007 is divisible by x² − 1.
(a) x²
(b) x
(c) x + 1
(d) −x

Question. If a polynomial 2x³ − 9x² + 15x + p, when divided by (x − 2), leaves −p as remainder, then p is equal to
(a) −16
(b) −5
(c) 20
(d) 10

Question. If a, b and g are the zeros of the polynomial 2x³ − 6x² − 4x + 30, then the value of (ab + bg + ga) is
(a) − 2
(b) 2
(c) 5
(d) − 30

Question. If ax³ + 9x² + 4x − 1 is divided by (x + 2), the remainder is −6; then the value of ‘a’ is
(a) −3
(b) −2
(c) 0
(d) 33 8

Question. If c, d are zeros of x² − 10ax − 11b and a, b are zeros of x² − 10cx − 11d, then value of a + b + c + d is
(a) 1210
(b) − 1
(c) 2530
(d) − 11

Question. If the ratio of the roots of polynomial x² + bx + c is the same as that of the ratio of the roots of x² + qx + r, then
(a) br² = qc²
(b) cq² = rb²
(c) q²c² = b²r²
(d) bq = rc

Question. The quadratic polynomial whose zeros are twice the zeros of 2x² − 5x + 2 = 0 is −
(a) 8x² − 10x + 2
(b) x² − 5x + 4
(c) 2x² − 5x + 2
(d) x² − 10x + 6

Question. If α,β,γ are the zeros of the polynomial x³ − 3x + 11, then the polynomial whose zeros are (α+β), (β+γ) and (γ+α) is −
(a) x³ + 3x + 11
(b) x³ − 3x + 11
(c) x³ + 3x − 11
(d) x³ − 3x − 11

Question. If α,β,γ are such that α + β + γ = 2, α² + β² + γ² = 6, α³ + β³ + γ³ = 8, then α4 + β⁴ + γ⁴ is equal to
(a) 10
(b) 12
(c) 18
(d) None

Question. If one zero of the polynomial ax² + bx + c is posi- tive and the other negative then (a,b,c ∈R, a ≠ 0)
(a) a and b are of opposite signs.
(b) a and c are of opposite signs.
(c) b and c are of opposite signs.
(d) a,b,c are all of the same sign.

Question. A real number is said to be algebraic if it satisfies a polynomial equation with integral coefficients. Which of the following numbers is not algebraic :
(a) 2/ 3
(b) 2
(c) 0
(d) π

Question. If two zeroes of p(x)= 2x⁴ + x³ – 14x² – 19x – 6 are –1 and –2, the other two zeroes are:       
(a) 1/ 2 and – 3
(b) −1/ 2 and – 3
(c) −1/ 2 and 3
(d) none of these

Question. The graph of y = – 3x² + 2x– 1 cuts the x-axis at : 
(a) 1/ 3 and 0
(b) 1/ 3 and 1/ 3
(c) do not cut
(d) none of these

Question. The two zeroes of f(x) = x⁴– 6x³ – 26x² + 138x – 35 are 2 ± √3 , the other two zeroes are :     
(a) 7 and –5
(b) –7 and 5
(c) –7 and – 5
(d) none of these

Question. The zeroes of y = x² + 7x + 12 are :   
(a) 4 and – 3
(b) –4 and –3
(c) –4 and 3
(d) 4 and 3

Question. If the zeroes of f(x) = x³ – 3x² + x + 1 are a – b, a, a + b, then value of a and b is :     
(a) a =1, b = −√ 2
(b) a =1, b = −√2
(c) a =1, b =√2
(d) none of these

Question. The zeroes of f (x) = 4 √3x² +5x − 2 √3 are :    
(a) 2/√3 and −√3/4
(b) −/√32 and −√3/4 
(c) −2/√3 and √3/4
(d) none of these

Question. The sum and product of zeroes of quadratic polynomial 3x² – 8x + 12 is :   
(a) −8/3 , 4 
(b) 8/3 , 4 
(c) −8/3 , −4 
(d) none of these

Question. The graph of y = f (x) is given in figure (ii). How many zeroes are there of f (x)? 

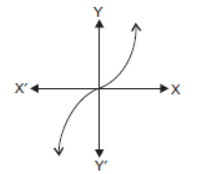
(a) 0
(b) 1
(c) 2
(d) many

Question. The number of polynomials having zeroes as –2 and 5 is   
(a) 1
(b) 2
(c) 10
(d) infinite

Question. The solution of x² + 6x + 9 = 0 is   
(a) –1
(b) 3
(c) –3
(d) 1

Question. The graph of y = f (x) is given in figure (i), for some polynomial f (x). The number of zeroes of f (x) is   

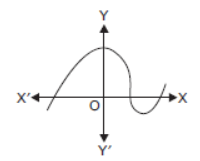
(a) 1
(b) 2
(c) 3
(d) many

Question. The value of k for which (–4) is a zero of the polynomial x² – x – (2k + 2) is   
(a) 2
(b) –6
(c) 9
(d) 8

Question. The graph of y = f (x) is given in figure (iii). How many zeroesare there of f (x)?    

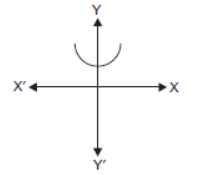
(a) 1
(b) 2
(c) 3
(d) None

Question. The zeroes of the quadratic equation 4s² – 4s + 1 are   
(a) 1/2 , 1/4
(b) 1/2 , 1/2
(c) 1/4 , 1/14
(d) 1/3 , 1/4

Question. The quadratic polynomial, sum of whose zeroes is 8 and their product is 12, is given by   
(a) x² – 8x + 12
(b) x² + 8x – 12
(c) x² – 5x + 7
(d) x² + 5x – 7

Question. A quadratic polynomial whose product and sum of zeroes are −13/ 5 and 3/5 , respectively.   
(a) k(x² + 12x + 5)
(b) k[x² – (8x) + (–9))
(c) k [x² − (1/2 x) + ( −7/5)]
(d) k [x² − (3/5 x) + (−13/5)] 

Question. A quadratic polynomial whose zeroes are 1 and –3 is   
(a) x² + 3x – 2
(b) x² + 5x – 5
(c) x² + 2x – 3
(d) None of these

Question. The sum and product of zeroes of the quadratic equation given in example 3 are respectively   
(a) 2, 3/4
(b) 0, 1/8
(c) 1, 1/4
(d) None of these

Question. The set of the zeroes of the polynomial x² – 25, their sum and product is   
(a) 4, 3; 7; 12
(b) –3, 3; 0; –9
(c) 5, –5; 0; –25
(d) None of these

Question. If the product of the zeroes of the polynomial ax² – 6x – 6 is 4, then value of a is   
(a) 1/8
(b) – 1/4
(c) 5/3
(d) –3/2 

Question. If a, b are the zeroes of the polynomial 2x² – 5x + 7, then a polynomial whose zeroes are 2α + 3β, 3α + 2β is     
(a) k(x² − 3/5 x + 21)
(b) k (x² − 25/2 x + 41)
(c) k (x² +9/2 x − 45 )
(d) None of these

Question. The value of k, if the sum of the zeroes of the polynomial x² – (k + 6) x + 2 (2k – 1) is half of their product is   
(a) 7
(b) 11
(c) 12
(d) None of these

Question. A quadratic equation x² – 2x – 8 is given. The zeroes of it are   
(a) –2 and 4
(b) 3 and 5
(c) 1 and 6
(d) None of these

Question. If the zeroes of the polynomial x² + px + q are double in value to the zeroes of 2x² – 5x – 3, the values of p and q are respectively   
(a) 5, 6
(b) 4, 7
(c) –5, –6
(d) –4, –7

Question. The sum and product of the zeroes of the quadratic equation given in example 1 are respectively   
(a) 2, 4
(b) 5, –8
(c) 6, 8
(d) 2, –8

Question. The remainder when f (x) = – 3x² + x⁴ + 4x + 5 is divided by –x² + 2 is   
(a) 6
(b) 8
(c) 12
(d) none of these

Very Short Answer and Question :

Question. Find the common zero of x² –1, x⁴ –1 and (x –1)² ?      

Question. Write a polynomial whose sum and product of zeroes are 2 and –9 respectively.   

Question. If α, β are the zeroes of quadratic polynomial x² – 3x + 2, form a quadratic polynomial whose zeroes are –α, and –β .  

Question. Find the zeroes of the polynomial 9x² – 25.   

Question. For what value of x, both the polynomials p(x) – x² – x – 6 and q(x) – x² – 2x –15 becomes zero. 

Question. How many maximum zeroes will the polynomial 3x³ + 6x² – 7 can have ?   

Question. What should be added to the polynomial p(x) = x² – 5x + 4, so that 2 is a zero of p(x)? 

Question. If α and β are the zeroes of the polynomial x2 – 5x + 6, find the value of 1/α +1/β . 

(a) If both Assertion and Reason are correct and Reason is the correct explanation of Assertion.
(b) If both Assertion and Reason are correct, but Reason is not the correct explanation of Assertion.
(c) If Assertion is correct but Reason is incorrect.
(d) If Assertion is incorrect but Reason is correct.

Question. Assertion : x³ + x has only one real zero.   
Reason : A polynomial of nth degree must have n real zeroes.

Question. Assertion : Degree of a zero polynomial is not defined.    
Reason: Degree of a non-zero constant polynomial is ‘0’.

Question. Assertion : Zeroes of f(x) = x² – 4x – 5 are 5, – 1.     
Reason : The polynomial whose zeroes are 2 + √3, 2 –√3 is x² – 4x + 7.

Question. Assertion : x² + 4x – 5 has two zeroes.   
Reason : A quadratic polynomial can have at the most two zeroes.

Question. Assertion : If one zero of polynomial p(x) = (k ² + 4) x² + 13x + 4k is reciprocal of other, then k = 2.   
Reason : If (x – α) is a factor of p(x), then p(α) = 0 i.e. α is a zero of p(x).