Worksheets For Class 9 Mathematics Euclids Geometry

Euclids Geometry Class 9 Worksheets have been designed as per the latest pattern for CBSE, NCERT and KVS for Grade 9. Students are always suggested to solve printable worksheets for Mathematics Euclids Geometry Grade 9 as they can be really helpful to clear their concepts and improve problem solving skills. We at worksheetsbag.com have provided here free PDF worksheets for students in standard 9 so that you can easily take print of these test sheets and use them daily for practice. All worksheets are easy to download and have been designed by teachers of Class 9 for benefit of students and is available for free download.

Mathematics Euclids Geometry Worksheets for Class 9

We have provided chapter-wise worksheets for class 9  Mathematics Euclids Geometry which the students can download in Pdf format for free. This is the best collection of  Mathematics Euclids Geometry standard 9th worksheets with important questions and answers for each grade 9th  Mathematics Euclids Geometry chapter so that the students are able to properly practice and gain more marks in Class 9  Mathematics Euclids Geometry class tests and exams.

Chapter-wise Class 9  Mathematics Euclids Geometry Worksheets Pdf Download

CBSE Class 9 Mathematics Introduction to Euclids Geometry Worksheet Set A

CBSE Class 9 Mathematics Introduction to Euclids Geometry Worksheet Set B

CBSE Class 9 Mathematics Introduction to Euclids Geometry Worksheet Set C

CBSE Class 9 Mathematics Euclids Geometry Worksheet Set A

CBSE Class 9 Mathematics Euclids Geometry Worksheet Set B

1. Euclid’s Definitions, Axioms and Postulates
2. Equivalent Versions of Euclid’s Fifth Postulate
The Greeks developed geometry is a systematic manner Euclid (300 B.C.) a greek mathematician, father of geometry introduced the method of proving mathematical results by using deductive logical reasoning and the previously proved result. The Geometry of plane figure is known as “Euclidean Geometry”. 
Axioms: The basic facts which are taken for granted without proof are called axioms some Euclid’s axioms are:
(i) Things which are equal to the same thing are equal to one another. i.e. a = b, b = c⇒a = c
(ii) If equals are added to equals, the wholes are equal i.e. a = b ⇒ a + c = b + c
(iii) If equals are subtracted from equals, the remainders are equal i.e. a = b⇒a – c = b – c
(iv) Things which coincide with one another are equal to one another.
(v) The whole is greater than the part.
Postulates: Axioms are the general statements, postulates are the axioms relating to a particular field.
Educlid’s five postulates are.
(i) A straight line may be drawn from any one point to any other point.
(ii) A terminated line can be produced indefinitely.
(iii) A circle can be drawn with any centre and any radius.
(iv) All right angles are equal to one another.
(v) If a straight line falling on two straight lines makes the interior angles on the same side of it taken together less than two right angles, then the two straight lines, if produced indefinitely meet on that side on which the angles are less than two right angles.
Statements: A sentence which is either true or false but not both, is called a statement.
eg. (i) 4+9=6 If is a false sentence, so it is a statement.
(ii) Sajnay is tall. This is not a statement because he may be tall for certain persons and may not be taller for others.
Theorems: A statement that requires a proof is called a theorem.
eg. (i) The sum of the angles of triangle is 180o .
(ii) The angles opposite to equal sides of a triangles are equal.
Corollary – Result deduced from a theorem is called its corollary.

Question. The three steps from solids to points are:
(a) Solids – surfaces – lines – points
(b) Solids – lines – surfaces – points
(c) Lines – points – surfaces – solids
(d) Lines – surfaces – points – solids

Question. The number of dimension, a point has:
(a) 0
(b) 1
(c) 2
(d) 3

Question. The total number of propositions in the Elements are:
(a) 465
(b) 460
(c) 13
(d) 55

Question. The number of dimensions, a solid has:
(a) 1
(b) 2
(c) 3
(d) 0

Question. Boundaries of solids are:
(a) surfaces
(b) curves
(c) lines
(d) points

Question. Boundaries of surfaces are:
(a) surfaces
(b) curves
(c) lines
(d) points

Question. In ancient India, the shapes of altars used for house hold rituals were:
(a) Squares and circles
(b) Triangles and rectangles
(c) Trapeziums and pyramids
(d) Rectangles and squares

Question. A pyramid is a solid figure, the base of which is
(a) only a triangle
(b) only a square
(c) only a rectangle
(d) any polygon

Question. The side faces of a pyramid are:
(a) Triangles
(b) Squares
(c) Polygons
(d) Trapeziums

Question. It is known that if x + y = 10 then x + y + z = 10 + z. The Euclid’s axiom that illustrates this statement is:
(a) First Axiom
(b) Second Axiom
(c) Third Axiom
(d) Fourth Axiom

Question. In Indus Valley Civilisation (about 3000 B.C.), the bricks used for construction work
were having dimensions in the ratio
(a) 1 : 3 : 4
(b) 4 : 2 : 1
(c) 4 : 4 : 1
(d) 4 : 3 : 2

Question. Euclid divided his famous treatise “The Elements” into:
(a) 13 chapters
(b) 12 chapters
(c) 11 chapters
(d) 9 chapters

Question. The number of interwoven isosceles triangles in Sriyantra (in the Atharva Veda) is:
(a) Seven
(b) Eight
(c) Nine
(d) Eleven

Question. In Ancient India, Altars with combination of shapes like rectangles, triangles and trapeziums were used for:
(a) Public worship
(b) Household rituals
(c) Both A and B
(d) None of A, B, C

Question. Euclid stated that all right angles are equal to each other in the form of
(a) an axiom
(b) a definition
(c) a postulate
(d) a proof

Question. Euclid belongs to the country:
(a) Babylonia
(b) Egypt
(c) Greece
(d) India

Question. Greek’s emphasised on:
(a) Inductive reasoning
(b) Deductive reasoning
(c) Both A and B
(d) Practical use of geometry

Question. Thales belongs to the country:
(a) Babylonia
(b) Egypt
(c) Greece
(d) Rome

Question. The number of dimensions, a surface has:
(a) 1
(b) 2
(c) 3
(d) 0

Question. Which of the following needs a proof?
(a) Theorem
(b) Axiom
(c) Definition
(d) Postulate

Question. ‘Lines are parallel if they do not intersect’ is stated in the form of
(a) an axiom
(b) a definition
(c) a postulate
(d) a proof

Question. Pythagoras was a student of:
(a) Thales
(b) Euclid
(c) Both A and B
(d) Archimedes

Question. The boundaries of the solids are curves.
Answer. The given statement is false because boundaries of solids are surfaces.

Question. The statements that are proved are called axioms.
Answer. The given statement is false because the statement that are proved are called theorems.

Question. The things which are double of the same thing are equal to one another.
Answer. True Since, it is one of the Euclid’s axioms. Some of Euclid’s axiom:
(1) Things which are equal to the same thing are equal to one another.
(2) If equals are added to equals, the wholes are equal.
(3) If equals are subtracted from equals, the remainder are equal.
(4) Things which coincide with one another are equal to one another.
(5) The whole is greater than the part.
(6) Things which are double of the same things are equal to one another.
(7) Thing which are halves of the same things are equal to one another.

Question. Euclidean geometry is valid only for curved surfaces.
Answer. The given statement is false because Euclidean geometry is valid only for the figures in the plane.

Question. Attempts to prove Euclid’s fifth postulate using the other postulates and axioms led to the discovery of several other geometries.
Answer. The given statement is true because these geometries are different from Euclidean geometry called non-Euclidean geometry.

Question. If a quantity B is a part of another quantity A, then A can be written as the sum of B and some third quantity C.
Answer. The given statement is true because it is one of Euclid’s axiom.

Question. “For every line l and for every point P not lying on a given line l, there exists a unique line m passing through P and parallel to l” is known as Play fair’s axiom.
Answer. The given statement is true, because it is an equivalent version of Euclid’s fifth postulate.

Question. The edges of a surface are curves.
Answer. The given statement is false because the edges of surfaces are line.

Question. Two distinct intersecting lines cannot be parallel to the same line.
Answer. The given statement is true, because it is an equivalent version of Euclid’s fifth postulate.

Question. Two salesmen make equal sales during the month of August. In September, each salesman doubles his sale of the month of August. Compare their sales in September.
Answer. Let the sales of two salesmen in the month of August be x and y. As, they make equal sale during the month of August, x = y. In September, each salesman double his sale of the month of August, So 2x = 2y.
Now, by Euclid’s axiom, thing which are double of the same things are equal to one another.
Hence, we can say that in the month of September also, two salesmen make equal sales.

Question. Look at the figure, and show that
Length AH > sum of lengths of AB + BC + CD.

Answer. We see the AB, BC and CD are parts of line.
Now, AB + BC + CD = AD                                                         …(1)
By Euclid’s axiom 5, the whole is greater than the part, so AH > AD
i.e., Length AH> sum of length of AB + BC + CD                [Using (1)]

Question. In the Fig.5.6, we have
BX = 1/2 AB
BY = 1/2 BC and AB = BY
Show that BX = BY.

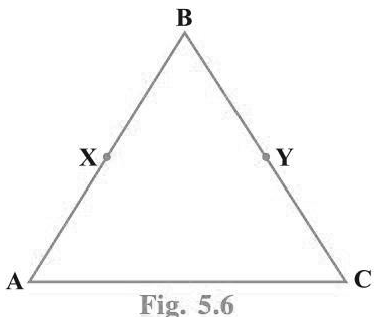
Answer. We have AB = BC [Given]
Now, by Euclid’s axiom 7, we have things which are halves of the same things are equal to one another.
∴ 1/2AB = 1/2BC
Hence, BX = BY. [ ∵ BX = 1/2AB and BY = 1/2 BC (Given)]

Question. In the Fig.5.4, we have
AB = BC, BX = BY. Show that AX = CY.

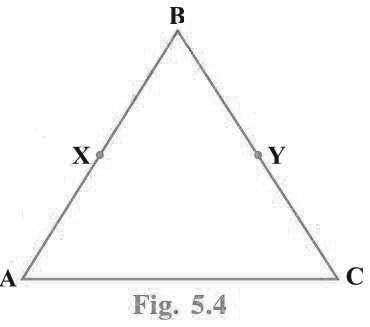
Answer. We have AB = BC                …(1) [Given]
And BX = BY                              …(2) [Given]
Subtracting (2) from (1), we get
Ab – BX = BC – BY
Now, By Euclid’s axiom 3, we have
If equals are subtracted from equals, the remainder are equal.
Hence, AX = CY.

Question. It is known that x + y = 10 and that x = z. Show that z + y= 10?
Answer. It is known that x + y = 10 and that x = z.
∴ x + y = z + y                [∵By Euclid’s axiom 2, if equals of are added to equals, the wholes are equal]
⇒ 10 = y + z                   [Using (1), x + y = 10]
Hence, z + y = 10.

Question. In the Fig.5.5, we have X and Y are the mid-points of AC and BC and AX = CY. Show that AC = BC.

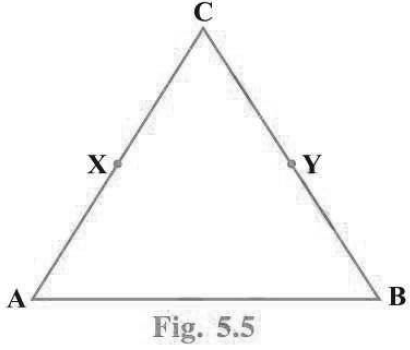
Answer. We have AX = CY [Given]
Now, by Euclid’s axiom 6, we have things which are double of the same thing are equal to
one another, so 2AX = 2CY
Hence, AC = BC.                 [∵X and Y are the mid- points of AC and BC]

Question. In the given figure, we have ∠ABC = ∠ACB,∠4 = ∠3. Show that ∠1 = ∠2.

Answer. We have
⇒ ∠ABC =∠ACB                …(1) [(Given)]
And ∠4 = ∠3                     …(2) [(Given)]
Now, subtracting (2) from (1), we get
Now, by Euclid’s axiom 3, if equals are subtracted from equals, the remainders are equal.
∠ABC − ∠4 = ∠ACB − ∠3
Hence, ∠1 = ∠2.

Question. In the given figure, we have
∠1= ∠2,Ð∠2 = ∠3. Show that ∠1 = ∠3.

Answer. We have
∠1 = ∠2 [Given]
∠2 = ∠3 [Given]
Now, by Euclid’s axiom 1, things which are equal to the same thing are equal to one other.
Hence, ∠1 = ∠3.

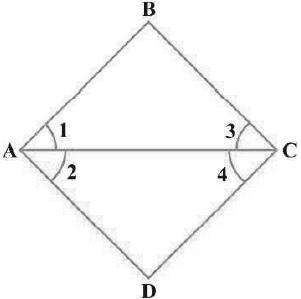
Question. In the given figure, we have ∠1 = ∠3 and ∠2 = ∠4. Show that ∠A = ∠C.

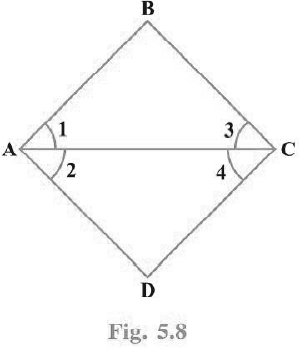
Answer. We have ∠1 = ∠3        …(1) [Given]
And ∠2 = ∠4                       …(2) [Given]
Now, by Euclid’s axiom 2, we have if equal are added to equals, the whole are equal.
Adding (1) and (2), we get
∠1+ ∠2 = ∠3 +∠4
Hence, ∠A = ∠C.

Question. In the Fig. 5.10, we have AC = DC, CB = CE. Show that AB = DE.

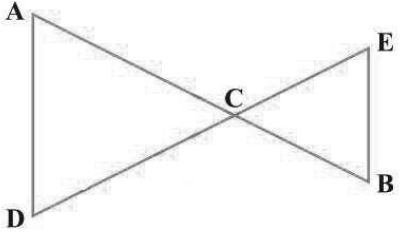
Answer. We have AC = DC               …(1) [Given]
And CB = CE                              …(2) [Given]
Now, by axiom 2, if equals are added to equals, the wholes are equal.
Adding (1) and (2), we get
        AC + CB = DC + CE
Hence, AB = DE.

Question. In the given figure, if Ox = 1/2 XY,PY 1/2 = XZ and OX = PX, show that XY = XZ.

Answer. We have OX = PX [Given]
Now
∴ Ox = 1/2 XY (Given) and PX = 1/2 XZ [Given]
∴ 1/2 XY = 1/2 XZ
                                    [ ∵Things which are halves of the same thing are equal to one another (Euclid’s axiom 7)]
 XY= XZ                       [∵ Things which are double of the same thing are equal to one another (Euclid’s axiom 6)]

Question. In the Fig.5.12:
(i) AB = BC, M is the mid-point of AB and N is the mid- point of BC. Show that AM = NC.
(ii) BM = BN, M is the mid-point of AB and N is the mid-point of BC. Show that AB = BC.

Answer. (i) We have AB = BC …(1) [Given]
Now, A, M, B are the three points on a line, and M lies between A and B, then
AM + MB = AB …(2)
Similarly, BN + NC = BC
So, we get AM + MB = BN + NC
From (1), (2), (3) and Euclid’s axiom 1]
Since M is the mid-point of AB and N is the mid-point of BC, therefore
             2AM = 2NC
Using axiom 6, things which are double of the same thing are equal to one another.
Hence, AM = NC.
(ii) We have BM = BN …(1)
As M is the mid-point of AB, so
            BM = AM …(2)
And N is the mid-point of BC,
            BN = NC …(3)
From (1), (2) and (3) and Euclid’s axiom 1, get
            AM = NC …(4)
Adding (4) and (1), we get
            AM + BM = NC + BN
Hence, AB = BC
[By axiom 2 if equals are added to equals, the wholes are equal]

Question. Study the following statement:
“Two intersecting lines cannot be perpendicular to the same line”. Check whether it is an equivalent version to the Euclid’s fifth postulate. [Hint: Identify the two
intersecting lines l and m and the line n in the above statement.]
Answer. Two intersecting lines cannot be both perpendicular to the same line because if two lines l and m are perpendicular to the same line n, then l and m must be parallel. The given statement is not an equivalent version of Euclid’s fifth postulate. 

Question. Read the following two statements which are taken as axioms:
(i) If two lines intersect each other, then the vertically opposite angles are not equal.
(ii) If a ray stands on a line, then the sum of two adjacent angles so formed is equal to 180°. Is this system of axioms consistent? Justify your answer.
Answer. The given system of axioms is not consistent because if a ray stands on a line and the sum of two adjacent angles so formed is equal to 180o, then for two lines which intersect each other, the vertically opposite angles becomes equal.

Question. Read the following statement:
An equilateral triangle is a polygon made up of three line segments out of which two line segments are equal to the third one and all its angles are 60° each. Define the terms used in this definition which you feel necessary. Are there any undefined terms in this? Can you justify that all sides and all angles are equal in a equilateral triangle?
Answer. The term need to be defined are:
Polygon: A simple closed figure made up of three or more line segments.
Line segment: Part of a line with two end points.
Line: Undefined term.
Point: Undefined term.
Angle: A figure formed by two rays with a common initial point.
Acute angle: Angle whose measure is between 0o and 90o.
Undefined terms used are: Line, part Two line segments are equal to third line segment Therefore, all three sides of an equilateral triangle are equal All its angle are 60o each. Therefore, all angles are equal (by Euclid’s first axiom, things which are equal to same things are equal to one another.)
Hence, we can say that all sides and all angles are equal in an equilateral triangle.

Question. Read the following axioms:
(i) Things which are equal to the same thing are equal to one another.
(ii) If equals are added to equals, the wholes are equal.
(iii) Things which are double of the same thing are equal to one another.
Check whether the given system of axioms is consistent or inconsistent.
Answer. The given system of axioms is consistent because (i), (ii) and (iii) are Euclid’s axiom.

Question. Read the following statements which are taken as axioms:
(i) If a transversal intersects two parallel lines, then corresponding angles are not necessarily equal.
(ii) If a transversal intersects two parallel lines, then alternate interior angles are equal. Is this system of axioms consistent? Justify your answer.
Answer. No, this system of axioms is not consistent because if a transversal intersects two parallel lines and if corresponding angles are not equal, then alternate interior angles cannot be equal.

Mathematics Euclids Geometry Worksheets for Class 9 as per CBSE NCERT pattern

Parents and students are welcome to download as many worksheets as they want as we have provided all free. As you can see we have covered all topics which are there in your Class 9 Mathematics Euclids Geometry book designed as per CBSE, NCERT and KVS syllabus and examination pattern. These test papers have been used in various schools and have helped students to practice and improve their grades in school and have also helped them to appear in other school level exams. You can take printout of these chapter wise test sheets having questions relating to each topic and practice them daily so that you can thoroughly understand each concept and get better marks. As Mathematics Euclids Geometry for Class 9 is a very scoring subject, if you download and do these questions and answers on daily basis, this will help you to become master in this subject.

Benefits of Free Worksheets for CBSE Class 9 Mathematics Euclids Geometry

1. You can improve understanding of your concepts if you solve NCERT Class 9 Mathematics Euclids Geometry Worksheet,
2. These CBSE Class 9 Mathematics Euclids Geometry worksheets can help you to understand the pattern of questions expected in Mathematics Euclids Geometry exams.
3. All worksheets for Mathematics Euclids Geometry Class 9 for NCERT have been organized in a manner to allow easy download in PDF format
4. Parents will be easily able to understand the worksheets and give them to kids to solve
5. Will help you to quickly revise all chapters of Class 9 Mathematics Euclids Geometry textbook
6. CBSE Class 9 Mathematics Euclids Geometry Workbook will surely help to improve knowledge of this subject

These Printable practice worksheets are available for free download for Class 9 Mathematics Euclids Geometry. As the teachers have done extensive research for all topics and have then made these worksheets for you so that you can use them for your benefit and have also provided to you for each chapter in your ebook. The Chapter wise question bank and revision worksheets can be accessed free and anywhere. Go ahead and click on the links above to download free CBSE Class 9 Mathematics Euclids Geometry Worksheets PDF.