Worksheets For Class 9 Mathematics Areas of Parallelogram and Triangle

Areas of Parallelograms and Triangles Class 9 PDF Worksheets have been designed as per the latest pattern for CBSE, NCERT and KVS for Grade 9. Students are always suggested to solve printable worksheets for Mathematics Areas of Parallelogram and Triangle Grade 9 as they can be really helpful to clear their concepts and improve problem solving skills. We at worksheetsbag.com have provided here free PDF worksheets for students in standard 9 so that you can easily take print of these test sheets and use them daily for practice. All worksheets are easy to download and have been designed by teachers of Class 9 for benefit of students and is available for free download.

Mathematics Areas Of Parallelogram And Triangle Worksheets for Class 9

We have provided chapter-wise worksheets for class 9 Mathematics Areas Of Parallelogram And Triangle which the students can download in Pdf format for free. This is the best collection of Mathematics Areas Of Parallelogram And Triangle standard 9th worksheets with important questions and answers for each grade 9th Mathematics Areas Of Parallelogram And Triangle chapter so that the students are able to properly practice and gain more marks in Class 9 Mathematics Areas Of Parallelogram And Triangle class tests and exams.

Chapter-wise Class 9 Mathematics Areas Of Parallelogram And Triangle Worksheets Pdf Download

1. Figures on the same Base and Between the same Parallels
2. Parallelograms on the same Base and between the same Parallels
3. Triangles on the same Base and between the same Parallels
• Area of a figure is a number (in square unit) associated with the part of the plane enclosed by that figure.
• Two congruent figures have equal areas but the converse is not true.
• Area of a parallelogram = (base X height )
• Area of a triangle = 1/2 X ´base X height
• Parallelogram on the same base and between the same parallels are equal in area.
• A parallelogram and a rectangle on the same base and between the same parallels are equal in area.
• Triangles on the same base and between the same parallels are equal in area.
• If a triangle and parallelogram are on the same base and between the same parallels, then. ( Area of a triangle = 1/2 X ´base X height)
• A diagonal of parallelogram divides it into two triangles of equal areas. In parallelogram ABCD, we have Area of ΔABD = area of ΔACD

• The diagonals of a parallelogram divide it into four triangles of equal areas therefore ar (ΔAOB)=ar (ΔCOD)=ar (ΔAOD)=ar (ΔBOC)

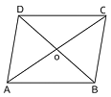
• If a parallelogram and a triangle are on the same base and between the same parallel, then area of the triangle is equal to one half area of the parallelogram.
• A median AD of a ΔABC divides it into two triangles of equal areas. Therefore ar (ΔABD)=ar (ΔCD)
• If the medians of a intersect at G, then ar (ΔAGB) = ar (ΔAGC) = ar (BGC) = 1/3 ar ( ΔABC )

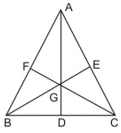
• Triangles with equal bases and equal areas have equal corresponding altitude.

CBSE Class 9 Mathematics Areas Of Parallelogram And Triangle Worksheet Set A

CBSE Class 9 Mathematics Areas Of Parallelogram And Triangle Worksheet Set B

CBSE Class 9 Mathematics Areas Of Parallelogram And Triangle Worksheet Set C

Question. The median of a triangle divides it into two
(a) triangles of equal area
(b) congruent triangles
(c) right triangles
(d) isosceles triangles

Question. In which of the following figures (Fig. 9.3), you find two polygons on the same base and between the same parallels?

Question. The figure obtained by joining the mid-points of the adjacent sides of a rectangle of sides 8 cm and 6 cm is:

(a) a rectangle of area 24 cm²
(b) a square of area 25 cm²
(c) a trapezium of area 24 cm²
(d) a rhombus of area 24 cm²

Question. In Fig. 9.4, the area of parallelogram ABCD is:

(a) AB × BM
(b) BC × BN
(c) DC × DL
(d) AD × DL

Question. In Fig. 9.5, if parallelogram ABCD and rectangle ABEM are of equal area, then:

(a) Perimeter of ABCD = Perimeter of ABEM
(b) Perimeter of ABCD < Perimeter of ABEM
(c) Perimeter of ABCD > Perimeter of ABEM
(d) Perimeter of ABCD = 1/2 = (Perimeter of ABEM)
Answer a

Question. The mid-point of the sides of a triangle along with any of the vertices as the fourth point make a parallelogram of area equal to
(a) 1/2 ar ( ΔABC)
(b) 1/3 ar (ΔABC)
(c) 1/4 ar (ΔABC)
(d) ar(ΔABC)

Question. Two parallelograms are on equal bases and between the same parallels. The ratio
of their areas is
(a) 1 : 2
(b) 1 : 1
(c) 2 : 1
(d) 3 : 1

Question. ABCD is a quadrilateral whose diagonal AC divides it in two parts, equal in area, then ABCD
(a) is a rectangle
(b) is always a rhombus
(c) is a parallelogram
(d) need not be any of (a), (b) or (c)

Question. If a triangle and a parallelogram are on the same base and between same parallels, then the ratio of the area of the triangle to the area of parallelogram is
(a) 1: 3
(b) 1: 2
(c) 3: 1
(d) 1: 4

Question. ABCD is a trapezium with parallel sides AB = a cm and DC = b cm (Fig. 9.6). E and F are the mid-points of the non-parallel sides. The ratio of ar (ABFE) and ar (EFCD) is

(a) a : b
(b) (3 a+ b) : (a + 3b)
(c) (a + 3b) : (3a + b)
(d) (2a + b) : (3a + b)

Question. ABCD is a parallelogram and X is the mid-point of AB. If ar (AXCD) = 24 cm², then ar (ΔABC) = 24cm².
Answer. We have ABCD is a parallelogram and X is the mid – point of AB.
Now, ar (ABCD) = ar (AXCD) + ar (ΔXBC)                            …(1)
∵ Diagonal AC of a parallelogram divides it into two triangles of equal area.
∴ ar (ABCD) = 2ar (ΔABC)                            …(2)
Again, X is the mid-point of AB, So
ar (ΔCXB) = 1/2 ar (ΔABC)                            …(3)
[∵ Median divides the triangle in two triangles of equal area]
∴ 2ar (ΔABC) = 24 + 1/2 ar (ΔABC) [Using (1), (2) and (3)]
∴ 2ar (ΔABC) − 1/2 ar (ΔABC) = 24
⇒ 3/2 ar (ΔABC) = 24
⇒ ar (ΔABC) = 2 X 24 / 3 = 16cm²
Hence, the given statement is false.

Question. PQRS is a rectangle inscribed in a quadrant of a circle of radius 13 cm. A is any point on PQ. If PS = 5 cm, then ar (PAS) = 30 cm².
Answer. It is given that A is any point on PQ, therefore, PA < PQ.
It is given that A is any point on PQ, therefore PA < PQ.

Now, ar (ΔPQR) = 1/2 × base × height
Now, ar (ΔPQR) = 1/2 × PQ × QR = 1/2 x 12 x 5 = 30cm²
[∵ PQRS is a rectangle ∴ RQ = SP = 5 cm]
As PA < PQ (= 12 cm)
So ar (ΔPAS) < ar (ΔPQR)
Or ar (ΔPAS) < 30cm² [ar (ΔPQR) = 30cm²]
Hence, the given statement is false.

Question. PQRS is a parallelogram whose area is 180cm² and A is any point on the diagonal QS. The area of ΔASR = 90cm².
Answer. PQRS is a parallelogram. We know that diagonal (QS) of a parallelogram divides parallelogram into two triangles of equal area, so
∴ ar (ΔQRS) = 1/2 ar ( ||gm PQRS)
= 1/2 x 180 = 90cm²
∵ A is any point on SQ
∴ ar (ΔASR) < ar (ΔQRS)
Hence, 2 ar (ΔASR) < 90cm²
Hence, the given statement is false.

Question. ABC and BDE are two equilateral triangles such that D is the mid-point of BC. Then ar (ΔBDE).= 1/4 = 1/4 ar (ΔABC)
Answer. ΔABC and ΔBDE are two equilateral triangles.
Let each sides of triangle ABC be x.
Again, D is the mid-point of BC, so each side of triangle BDE is x/2

Question. In the given figure, ABCD and EFGD are two parallelogram and G is the mid-point of CD. Then ar (ΔDPC) = 1/2 ar (|| gmEFGD)

Answer. As DDPC and ||gm ABCD are on the same base DC and between the same parallels AB and DC, So
ar (ΔDPC) = 1/2 ar (||gm ABCD)
= ar (||gm EFGD)                          [∵ G is the point of DC]
Hence, the given statement is false.

Question. In Fig.9.11, PSDA is a parallelogram. Points Q and R are taken on PS such that PQ = QR = RS and PA||QB||RC. Prove that ar (PQE) = ar (CFD).

Answer. PSDA is a parallelogram. Points Q and R are taken on Ps such that PQ = RS = RS and PA||QB||RC.
We have to prove that ar (ΔPQE) = ar (ΔCFD).
Now, PS = AD [Opp. Sides of a ||gm]
∴ 1/3 PS =1/2 AD ⇒ PQ = CD                 …(1)
Again, PS||AD and QB cut them,
∴ ∠PQE = ∠CBE [Alt. ∠s ]                        …(2)
Now, QB||RS and AD cut them
∴ ∠QBD = ∠RCD [Corres. ∠s ]                …(3)
So, ∠PQE = ∠FCD                                   …(4)
[From (2) and (3), ∠CBE and ∠QBD are same and ∠RCD and ∠FCD are same]
Now, in ΔPQE and ΔCFD
∠PQE = ∠CDF                           [Alt. ∠s ]
PQ = CD                                    [From (1)]
And ∠PQE = ∠FCD                    [From (4)]
∴ ΔPQE ≅ ΔCFD                        [By ASA congruence rule]
Hence, ar (ΔPQE) = ar (ΔCFD)  [Congruence Ds are equal in area]

Question. X and Y are points on the side LN of the triangle LMN such that LX = XY= YN. Through X, a line is drawn parallel to LM to meet MN at Z (See Fig. 9.12). Prove that ar (LZY) = ar (MZYX).

Answer. We have to prove that ar (ΔLZY) = ar (MZYX )
Since ΔLZY and ΔXMZ are on the same base and between the same parallels LM and XZ, we have
ar (ΔLXZ) = ar (ΔXMZ) ..(1)
Adding ar (ΔXYZ) to both sides of (1), we get
ar (ΔLXZ) + ar (ΔXYZ) = ar (ΔXMZ) + ar (ΔXYZ)
⇒ ar (ΔLZY) = ar (MZYX )

Question. The area of the parallelogram ABCD is 90 cm² (see fig). Find
(i) ar (ABEF)
(ii) ar (ABD)
(iii) ar (BEF)

Answer. (i) Since parallelograms on the same base and between the same parallels are equal in area, so we have
ar (||gm ABEF) = ar (||gm ABCD)
Hence, ar (||gm ABEF) = ar (||gm ABCD) = 90 cm²
(ii) ar (ΔABD) = 1/2 ar (||gmABCD)
[∵ A diagonal of a parallelogram divides the parallelogram in two triangles of equal area]
= 1/2 × 90cm² = 45cm²
ar (ΔBEF) = 1/2 ar (||gmABEF) = 1/2 x 90cm² = 45cm²

Question. In Δ ABC, D is the mid-point of AB and P is any point on BC. If CQ || PD meets AB in Q (Fig. 9.14), then prove that ar (ΔBPQ) = ar (ΔABC)

Answer. D is the mid-point of AB and P is any point on BC of Δ ABC. CQ||PD meets AB in Q, we have to prove that ar (ΔBPQ) = 1/2 ar (ΔABC) Join CD. Since median of a triangle divides it into two triangles of equal area, so we have
ar (ΔBCD) = 1/2 ar (ΔABC)                                         …(1)
Since, triangles on the same base and between the same parallels are equal in area, so we have
ar (ΔDPQ) = ar (ΔDPC)                                               …(2)
                                  [∵ Triangle DPQ and DPC are on the same base DP and
between the same parallels DP and CQ]
ar (ΔDPQ) + ar (ΔDPB) = ar (ΔDPC) + ar (ΔDPB)
Hence, ar (ΔBPQ) = ar (ΔBCD) = 1/2 ar (ΔABC)         [Using (1)]

Question. ABCD is a square. E and F are respectively the midpoints of BC and CD. If R is the mid-point of EF (Fig. 9.15), prove that ar (ΔAER) = ar (ΔAFR).
Answer. ABCD is a square. E and F are respectively the mid-points of BC and CD. If R is the midpoint of EF, we have to prove that ar (ΔAER) = ar (ΔAFR). In ΔABE and ΔADF , we have

AB = AD [Sides of a square are equal]
∠ABE = ∠ADF [Each 90^o]
BE = DF [∵E is the mid-point of BC and F is the mid-point of
                      CD. Also 1/2 BC = 1/2 CD ]
ar (ΔABE) = ar (ΔADF)                                   [By SAS Congruence rule]
∴ AE = AF [CPCT]                                             …(1)
Now, in ΔAER and ΔAFR, we have
AE = AF                                                      [From (1)]
ER = RF                                                        [∵R is mid-point of EF]
And AR = AR                                               [Common side]
∴ ar (ΔAER) = ar (ΔAFR)                              [By SSS rule of congruence]
Hence, as ar (ΔAER) = ar (ΔAFR) [∵Congruent triangles are equal in area]

Question. O is any point on the diagonal PR of a parallelogram PQRS (Fig. 9.16). Prove that ar (PSO) = ar (PQO).

Answer. Join SQ, bisect the diagonal PM at M. Since diagonals of a parallelogram bisect each other,
so SM = MQ.
Therefore, PM is a median of ΔPQS
ar (ΔPSM) = ar (ΔPQM)                     …(1)
[∵ Median divides a triangle into two triangles of equal area]

Again, as Om is the median of triangle ΔOSQ , so
ar (ΔOSM) = ar (ΔOQM)                      …(2)
Adding (1) and (2), we get
ar (ΔPSM) + ar (ΔOSM) = ar (ΔPQM) + ar (ΔOQM)
⇒ ar (ΔPSO) = ar (ΔPQO)
Hence, proved.

Question. ABCD is a parallelogram in which BC is produced to E such that CE = BC (Fig. 9.17). AE intersects CD at F. If ar (DFB) = 3 cm², find the area of the parallelogram ABCD.

Answer. In DADF and DEFC , we have

∠DAF = ∠CEF                                  [Alt. interior ∠s ]
AD = CE                                         [∵ AD = BC = CE [Given]]
∠ADF = ∠FCE                                [Alt interior ∠s ]
∴ ADF ≅ ECF                                  [By SAS rule of congruence]
∴ DF = CF                                      [CPCT]
As BF is the median of ΔBCD ,
∴ ar (ΔBDF = 1/2 ar (ΔBCD)                               …(1)
[∵ Median divides a triangle into two triangles of equal area]
Now, if a triangle and parallelogram are on the same base and between the same parallels, then the area of the triangles is equal to half the area of the parallelogram.
∴ ar (ΔBCD) = 1/2 ar (||gmABCD)                       …(2)
∴ By (1), we have ar (ΔBDF) 1/2 {1/2 ar (||gmABCD)}
⇒ 3cm² = 1/4 ar (||gmABCD)
⇒ ar (||gmABCD) = 12cm²
Hence, the area of the parallelogram ABCD is 12 cm².

Question. In trapezium ABCD, AB || DC and L is the mid-point of BC.
Through L, a line PQ || AD has been drawn which meets AB in P and DC produced in Q (Fig. 9.18). Prove that ar (ABCD) = ar (APQD)

Answer. AS AB||DC, so AB||DQ
In ΔCLQ and ΔBLP , we have
∴ ∠QCL = ∠LBP                     [Alt. ∠s ]
CL = LB                                  [∵L is the mid-point of BC]
∠CLQ = ∠BLP                        [Vertically opposite ∠s ]
∴ DQCL ≅ ∠BLP                   [By ASA congruence rule]
⇒ ar (ΔCLQ) = ar (ΔBLP)          …(1) [Congruence Ds are equal in area]
Adding ar (APLCD) to both sides of (1), we get
ar (ΔCLQ) + ar (APLCD) = ar (ΔBLP) + ar (APLCD)
⇒ ar (APQD) = ar (ABCD)
Hence,              ar (ABCD) = ar (APQD)

Question. A point E is taken on the side BC of a parallelogram ABCD. AE and DC are produced to meet at F. Prove that ar (ΔADF) = ar (ΔABFC)
Answer. Given: ABCD is a parallelogram. A point E is taken on the Side BC. AE and DC are produced to meet at F.
Proof: Since ABCD is a parallelogram and diagonal AC divides it into two triangles of equal area, we have

ar (ΔADC) = ar (ΔABC)                          …(1)
As DC||AB, So CF||AB
Since triangles on the same base and between the same parallels are equal in area, so we have
ar (ΔACF) = ar (ΔBCF)                          …(2)
Adding (1) and (2), we get
ar (ΔADC) + ar (ΔACF) = ar (ΔABC) + ar (ΔBCF)
⇒ ar (ΔADF) = ar (ABFC)
Hence, proved.

Question. If the mid-points of the sides of a quadrilateral are joined in order, prove that the area of the parallelogram so formed will be half of the area of the given quadrilateral (Fig. 9.19). [Hint: Join BD and draw perpendicular from A on BD.]

Answer.

Given: A quadrilateral ABCD in which the mid-point of the sides of it are joined in order of form parallelogram PQRS.
To prove: ar (|| gmPQRS) = ar (ABCD)
Construction: Join BD and draw perpendicular from A and BD which intersect SR and BD at X and Y respectively.
Proof: In ΔABD, S and R are the mid-points of sides AB and AD respectively.
∴ SR || BD
⇒ SX || BY
⇒ X is the mid-point of AY              [Converse of mid-point theorem]
⇒ AX = XY             …(1)                   [∵ S is the mid-point of AB and SX||BY]

Question. The diagonals of a parallelogram ABCD intersect at a point O. Through O, a line is drawn to intersect AD at P and BC at Q. Show that PQ divides the parallelogram into two parts of equal area.
Answer. ∵ AC is a diagonal of the ||gm ABCD

∴ ar ΔACD = 1/2 ar (||gmABCD)          ..(1)
Now, in ΔAOP and ΔCOQ
AO = CO [∵ Diagonals of a   ||gm bisects each other]
∠AOP = ∠COQ                     [Vert. opp. ∠s ]
∠OAP = ∠OCQ                     [Alt. ∠s; AB||CD]
∴ DAOP ≅ DCOQ                 [By ASA cong. Rule
Hence, ar(ΔAOP) = ar(ΔCOQ) [Cong. Area axiom]         ..(2)
Adding ar(quad. AOQD) to both sides of (2), we get
ar(quad.AOQD) + ar(ΔAOP) = ar(quad.AOQD) + ar(ΔCOQ)
⇒ ar(quad. APQD) = ar(ΔACD)

Question. The medians BE and CF of a triangle ABC intersect at G. Prove that the area of ΔGBC = area of the quadrilateral AFGE.
Answer. BE and CF are medians of a triangle ABC intersect at G. We have to prove that the
ar(ΔGBC) = area of the quadrilateral AFGF.
Since, median (CF) divides a triangle into two triangles of equal area, so we have
ar(DBCF) = ar(DACF)
⇒ ar(DGBF) + ar(DGBC) = ar(AFGE) + ar(DGCE)               ..(1)

  Since, median (BE) divides a triangle into two triangle of equal area, so we have
⇒ ar(ΔGBF) + ar(AFGE) = ar(ΔGCE) + ar(ΔGBE) …(2)
Subtracting (2) and (1), we get
ar(ΔGBC) − ar(AFGE) = ar(AFGE) − ar(ΔGBC)
⇒ ar(ΔGBC) + ar(ΔGBC) = ar(AFGE) + ar(AFGE)
⇒ 2ar(ΔGBC) = 2ar(AFGE)
Hence, ar(ΔGBC) = ar(AFGE)

Question. In Δ ABC, if L and M are the points on AB and AC, respectively such that LM || BC. Prove that ar (ΔLOB) = ar (ΔMOC).

Answer. Since triangles on the same base and between the same parallels are equal in area, So we have
∴ ar(ΔLBM) = ar(ΔLCM)
[ ΔLBM and ΔLCM are on the same base LM and between the same parallels LM and BC]
∴ ar(ΔLBM) = ar(ΔLCM)
⇒ ar(ΔLOM) + ar(ΔLOB) = ar(ΔLOM) + ar(ΔMOC)
Hence, ar(ΔLOB) = ar(ΔMOC)                    [Cancelling (ΔLOM) from both sides]

Question. In Fig. 9.24, CD||AE and CY||BA. Prove that ar (ΔCBX) = ar ( ΔAXY)

Answer. CD||AE and CY||BA. We have to prove that ar(DCBX ) = ar(DAXY).
Since triangle on the same base and between the same parallels are equal in area, so we have
ar(ΔABC) = ar(ΔABY)
⇒ ar(ΔCBX ) + ar(ΔABX ) = ar(ΔABX ) + ar(ΔAXY)
Hence, ar(ΔCBX ) = ar(ΔAXY)                         [Cancelling ar(ΔABX ) from both sides]

Question. ABCD is a trapezium in which AB||DC, DC = 30 cm and AB = 50 cm. If X and Y are, respectively the mid-points of AD and BC, prove that
Answer. ar (DCYX) = 7/9 ar(XYBA)
Answer. In ΔMBY and ΔDCY , we have
∠1 = ∠2               [Vertically opposite ∠s ]
∠3 = ∠4               [∵AB||DC and alt. ∠s are equal]

BY = CY                               [∵ Y is the mid-point of BC]
∴ ΔMBY ≅ ΔDCY                [By ASA Cong. Rule]
So, MB = DC = 30 cm       [CPCT]
Now, AM = AB + BM = 50 cm + 30 cm = 80 cm

In ΔADM,we have XY = 1/2 AM = 1/2 x 80cm = 40cm
As AB||XY||DC and X and Y are the mid-points of AD and BC, so height of trapezium DCXY and XYBA are equal. Let the equal height be h cm.

Question. In Fig. 9.25, ABCDE is any pentagon. BP drawn parallel to AC meets DC produced at P and EQ drawn parallel to AD meets CD produced at Q. Prove that ar (ABCDE) = ar( ΔAPQ)

Answer. BP||AC and AD||EQ.
Since, triangles on the same base and between the same parallels are equal in area
ar(ΔABC) = ar(ΔAPC)                                     …(1)
And ar(ΔADE) = ar(ΔADQ)                            …(2)
Adding (1) and (2), we get
ar(ΔABC) + ar(ΔADE) = ar(ΔAPC) + ar(ΔADQ)
Adding ar(DACD) to both sides, we get
ar(ΔABC) + ar(ΔADE) + ar(ΔACD) = ar(ΔAPC) + ar(ΔADQ) + ar(ΔACD)
Hence, ar(ABCDE) = ar(ΔAPQ)

Question In Fig. 9.27, ABCD and AEFD are two parallelograms. Prove that ar ( DPEA) = ar (DQFD) [Hint: Join PD].
Answer.

Answer. ABCD and AEFD are two parallelograms.
We have to prove that ar(ΔPEA) = ar(ΔQFD). Join PD.
In ΔPEA and ΔQFD, we have
∠APE = ∠DQF                               [∵Corresp. ∠s are equal as AB||CD]
∠AEP = ∠DFQ                               [∵Corresp. ∠s are equal as AE||DF]
AE = DF                                         [∵opposite sides of a ||gm are equal]
∴ ΔPEA ≅ ΔQFD [By AAS cong. Rule]
Hence, ar(ΔPEA) = ar(ΔQED)

Question. If the medians of a Δ ABC intersect at G, show that ar (AGB) = ar (AGC) = ar (BGC)= 1/2 ar ABC 
Answer. Given: Medians AE, BF and CD of Δ ABC intersect at G.

To prove: ar(ΔAGB) = ar(ΔAGC) = ar(ΔBGC)
= 1/3 ar (ΔABC)
Construction: Draw BP ⊥ EG.
Proof: AG = 2/3 AE                 [∵Centroid divides the median in the ration 2:1]
Now ,ar(ΔAGB) = 1/2 × AG× BP

Similarly, ar (ΔABC) = ar (ΔBGC) = 1/3 ar (ΔABC)
∴ ar (ΔAGB) = ar(ΔAGC) = ar (ΔBGC) 1/3 = ar( ΔABC) Hence, proved.

Question. In Fig. 9.26, X and Y are the mid-points of AC and AB respectively, QP||BC and CYQ and BXP are straight lines. Prove that ar (ΔABP) = ar (ΔACQ).

Answer. In triangle ABC, X and Y are the mid-points of AB and AC.
∴ XY||BC [BY mid-point theorem]
Since triangles on the same base (BC) and between the same parallels (XY||BC) are equal in area
∴ ar(ΔBYC) = ar(ΔBXC)                                          …(1)
Subtracting ar(ΔBOC) from both sides, we get
ar(ΔBYC)− ar(ΔBOC) = ar(ΔBXC) − ar(ΔBOC)
a) Flash and thunder are produced simultaneously. But thunder is heard a few seconds after the flash is seen.
⇒ ar(ΔBOY) = ar(ΔCOX )                  …(2)
Adding ar(ΔXOY ) to both sides of (2), we get
ar(ΔBOY )+ ar(ΔXOY) = ar(ΔCOX ) + ar(ΔXOY)              …(3)
Now, quad. XYAP and XYQA are on the same base XY and between the same parallels XY
and PQ.
∴ ar(XYAP) = ar(XYQA)                …(4)
Adding (3) and (4), we get
ar(ΔBXY )+ ar(XYAP) = ar(ΔCXY) + ar(XYAQ)
Hence, ar(ΔABP) = ar(ΔACQ)

Mathematics Areas Of Parallelogram And Triangle Worksheets for Class 9 as per CBSE NCERT pattern

Parents and students are welcome to download as many worksheets as they want as we have provided all free. As you can see we have covered all topics which are there in your Class 9 Mathematics Areas of Parallelogram and Triangle book designed as per CBSE, NCERT and KVS syllabus and examination pattern. These test papers have been used in various schools and have helped students to practice and improve their grades in school and have also helped them to appear in other school level exams. You can take printout of these chapter wise test sheets having questions relating to each topic and practice them daily so that you can thoroughly understand each concept and get better marks. As Mathematics Areas of Parallelogram and Triangle for Class 9 is a very scoring subject, if you download and do these questions and answers on daily basis, this will help you to become master in this subject.

Benefits of Free Areas of Parallelograms and Triangles Class 9 PDF Worksheets

1. You can improve understanding of your concepts if you solve NCERT Class 9 Mathematics Areas of Parallelogram and Triangle Worksheet,
2. These CBSE Class 9 Mathematics Areas of Parallelogram and Triangle worksheets can help you to understand the pattern of questions expected in Mathematics Areas of Parallelogram and Triangle exams.
3. All worksheets for Mathematics Areas of Parallelogram and Triangle Class 9 for NCERT have been organized in a manner to allow easy download in PDF format
4. Parents will be easily able to understand the worksheets and give them to kids to solve
5. Will help you to quickly revise all chapters of Class 9 Mathematics Areas of Parallelogram and Triangle textbook
6. CBSE Class 9 Mathematics Areas of Parallelogram and Triangle Workbook will surely help to improve knowledge of this subject

These Printable practice worksheets are available for free download for Class 9 Mathematics Areas of Parallelogram and Triangle. As the teachers have done extensive research for all topics and have then made these worksheets for you so that you can use them for your benefit and have also provided to you for each chapter in your ebook. The Chapter wise question bank and revision worksheets can be accessed free and anywhere. Go ahead and click on the links above to download free CBSE Class 9 Mathematics Areas of Parallelogram and Triangle Worksheets PDF.