Please refer to Trigonometric Functions MCQ Questions Class 11 Mathematics below. These MCQ questions for Class 11 Mathematics with answers have been designed as per the latest NCERT, CBSE books, and syllabus issued for the current academic year. These objective questions for Trigonometric Functions will help you to prepare for the exams and get more marks.
Trigonometric Functions MCQ Questions Class 11 Mathematics
Please see solved MCQ Questions for Trigonometric Functions in Class 11 Mathematics. All questions and answers have been prepared by expert faculty of standard 11 based on the latest examination guidelines.
MCQ Questions Class 11 Mathematics Trigonometric Functions
Question: If tan α =sinθ- cosθ/sin θcos θ, then sin a α is equal to
(a) ± sin θ+cos θ /√2
(b) ± sin θ-cosθ/√2
(c) ± – (sin θcos θ) q q
(d) None of these
Answer
B
Question: If cosec θ – sin θ =a3 and sec θ – cos θ = b3,then is a2b2(a2+b2) is equal to
(a) 2
(b) sin2/θ
(c) cos2/θ
(d) 1
Answer
D
Question: If cosec θ – = cot θ = p, then the value of cosec θ is
(a) 1/2(p+1/p)
(b) 1/2(1-1/p)
(c) (1+1/p)
(d) (1-1/p)
Answer
B
Question: The value of the expression tanθ/1-cot +cot/1-tan θ -sec θ cosec θ is equal to
(a) -1
(b) 0
(c) 1
(d) None of these
Answer
C
Question: if cosec A + = cot A =11/2 then tanA is equal to
(a) 21/22
(b) 15/16
(c) 117/43
(d) 44/117
Answer
D
Question: The angle subtended at the centre of a circle of diameter 50 cm by an arc of length 11 cm is
(a) 25° 27′
(b) 23° 12′
(c) 25° 12′
(d) None of these
Answer
C
Question: If cos2 B-1=tan2 A, then cosA cosB is equal to
(a) ±1/2
(b) ±1/3
(c) ±1/√2
(d) None of these
Answer
C
Question: If 3 sin θ cos θ = 5, then the value of 5θ -3 cos θ is
(a) ± 9
(b) ± 3
(c) ± 4
(d) ± 5
Answer
B
Question: If sin3 θ+y cos3 θ = cos θ sin θ and x sin θ = y cos θ, then
(a) x2+y2=2
(b) x2+y2 = 4
(c) x2+ y2 3
(d) x2+y2=1
Answer
D
Question: If sec A= a +(1/4a), then sec A + tan is equal to
(a) 2a or 1/2a
(b) a or 1/a
(c) 2a or 1/a
(d) a or 1/2a
Answer
A
Question: cot π/20 cot 3π/20 cot 5π/20 cot 7π/20 cot 9π/20 is equal to
(a) 0
(b) -1
(c) 1
(d) None of these
Answer
C
Question:
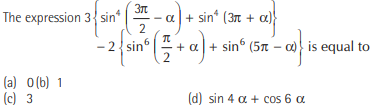
Answer
B
Question: If cos θ = – √3/2 and sin α= -3/5, where θ does not lie in the third quadrant and α lies in the third quadrant, then 2 tan α+√3 tan θ /cot2 θ +cos α is equal to
(a) 5/22
(b) 7/22
(c) 9/22
(d) 22/5
Answer
A
Question: If√1-sin α/√1+sin α = sec α- tan α, then the quadrants in which α lies are
(a) II and III
(b) I and IV
(c) I and II
(d) III or IV
Answer
B
Question: The expression (sin 3x+ sinx ) sinx+ (cos 3x- cosx ) cosx is equal to
(a) 0
(b) 1
(c) –1
(d) 1
Answer
A
Question: The value of cos4 π/8 + cos4 3π/8 + cos4 3π/8 +cos4 5π/8 + cos4 7π/8 is equal to
(a) 2
(b) 1/2
(c)3/2
(d) None of these
Answer
C
Question:
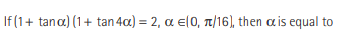
(a) π/20
(b) π/30
(c) π/40
(d) π/60
Answer
A
Question: If sinB=1/5 sin(2A+B),then tan(A+B)/tan A is equal to
(a) 2/3
(b) 3/2
(c) 3/4
(d) None of these
Answer
B
Question:

(a) 3
(b) 2
(c) 1
(d) 0
Answer
D
Question:
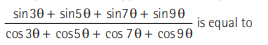
(a) tan3θ
(b) cot3θ
(c) tan6 θ
(d) cot6 θ
Answer
C
Question: The minimum value of 9 tan2 θ+4 cot2θ is
(a) 13
(b) 9
(c) 6
(d) 12
Answer
D
Question: The greatest value of 4 sin θ+3 cosθ + 10 is
(a) 13
(b) 15
(c) –15
(d) –13
Answer
B
Question:

Answer
C
Question: The minimum value of 2 sinx+2cos x is
(a) 1
(b) 2
(c) 2-1/√2
(d) 21-1/√2
Answer
D
Question: If 1-tan x/1+tan x = tan y and x-y = π/6, then x and y are respectively
(a) 5π/24, π/24
(b) -7π/24,-11π/24
(c) -115π/24,-119π/24
(d) All of these
Answer
D
Question:
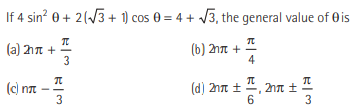
Answer
D
Question: The equation cos 4x-(a+2) cos2 x-(a+3)=0 possesses a solution, if
(a) a > – 3
(b) a < – 2
(c) -3 ≤ a ≤-2
(d) a is any positive integer
Answer
C
Question:

Answer
A
Question:
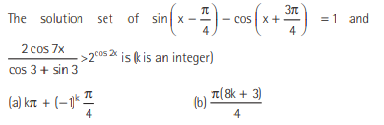
Answer
B
Question: If 1+sin θ + sin2 θ +…∞ = 4+2√3,0<θ <π,θ ≠π/2, , then
(a) θ =π/6
(b) θ=π/3
(c) θ =π/3 or π/6
(d) θ= π/3 or 2π/3
Answer
D
Question:
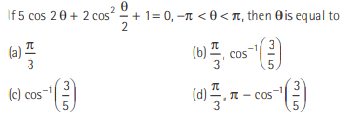
Answer
D
Question:
(a) -171/221
(b) -21/221
(c) 21/221
(d) 171/221
Answer
D
Question: If cos A =-m cos B and cot A+B/2 ∈(θ-β) b, then sin2 (α- β) +-2ab cos (α- β) is equal to
(a) a2 +b2
(b) a2 – b2
(c) b2-a2
(d) – a2– b2
Answer
A
Question: If cos A= m cos B and cot A+B/2 =λ tan B-A/2, tan ,lthen λ is
(a) m/m – 1
(b) m+1/m
(c) m+1/m-1
(d) None of these
Answer
C
Question: The value of sin(45°+θ)- cos (45°-θ) is
(a) 2 cos θ
(b) 2 sin θ
(c) 1
(d) 0
Answer
D
Question: If tan α =(1+2-x, tan β =(1+2 x+1)-1, then α + β equals
(a) π/6
(b) π/4
(c) π/3
(d) π/2
Answer
B
Question: The value of cos 12°+ cos 84°+ cos 156°+ cos 132° is
(a) 1/2
(b) 1
(c) -1/2
(d) 1/8
Answer
C
Question: cos 2θ cos 2Φ +sin2 (θ-Φ) -sin2 (θ+Φ) is equal to
(a) sin 2(θ+Φ)
(b) cos 2(θ+Φ)
(c) sin 2(θ-Φ)
(d) cos 2(θ-Φ)
Answer
B
Question: If cosα+ cosβ=0= sinα+ sinβ , cos2α+ cos2β equal to
(a) 2cos (α+β )
(b) -cos (α+β )
(c) 3 cos(α+β )
(d) None of these
Answer
B
Question:

Answer
C
Question: The value of sin20° sin 40° sin60° sin80° is equal to
(a) -3/16
(b) 5/16
(c) 3/16
(d) -5/16
Answer
C
Question: The value of cos2 48° – sin2 12° sin is
(a) √5+ 1/8
(b) √5- 1/8
(c) √5+ 1/5
(d) √5+ 1/2√2
Answer
A
Question: The value of cos π/5 cos2π/5 cos 4π/5 cos 8π/5 is equal to
(a) 1/16
(b) 0
(c) -1/8
(d) -1/16
Answer
D
Question: If cos(θ-α) = sin (θ-β) = b, then cos 2(α-β ) +2absin (α-β) is equal to
(a) 4a2b2
(b) a2-b2
(c) a2 +b2
(d) – a2 b2
Answer
C
Question: If angle θ be divided into two parts such that the tangent of one part is k times the tangent of the other and f is their difference, then sin θ is equal to
(a) k+1/k-1sin θ
(b) k-1/k+1 sinθ
(c) 2k -1/2k+1 sin θ
(d) None of these
Answer
A
Question:
Answer
A
Question: If A+ B+ C + = π and cosA= cosB cosC, then tan B tan C is equal to
(a) 1/2
(b) 2
(c) 1
(d) -1/2
Answer
B
Question: The value of sin 12° sin 48° sin 54° is equal to
(a) 1/16
(b) 1/32
(c) 1/8
(d) 1/4
Answer
C
Question: If cos (θ+Φ) =m cos (θ-Φ), then 1-m/1+m cot Φ is equal to
(a) tan q
(b) – tan q
(c) 2 tan q
(d) None of these
Answer
A
Question:

Answer
B
Question: If angle θ is divided into two parts such that the tangent of one part is K times the tangent to other and Φ is their difference, then sin θ is equal to
(a) K+1/K–1sinθ/2
(b) K+1/K–1sinΦ/2
(c) K+1/K–1sinΦ
(d) K–1/K+1sinΦ
Answer
C
Question: If tan θ + sec θ = p, then what is the value of sec θ ?
(a) p2+1/p2
(b) p2+1/√p
(c) p2+1/2p
(d) p+1/2p
Answer
C
Question: General solution of tan 5θ = cot 2θ is
(a) θ = nπ/7 + π/14
(b) θ = nπ/7 + π/5
(c) θ = nπ/7 + π/2
(d) θ = nπ/7 + π/3
Answer
A
Question: If cos x + cos y + cos α = 0 and sin x + sin y + sin α = 0, then cot( x + y/2) =
(a) sin α
(b) cos α
(c) cot α
(d) sin ( x + y/2)
Answer
C
Question: Let sinx = 3/5 , x lies in second quadrant.

Codes:
A B C D E
(a) 3 4 2 5 1
(b) 3 4 1 5 2
(c) 3 2 4 5 1
(d) 1 2 5 4 3
Answer
A
Question: The number of values of x in the interval [0, 3π] satisfying the equation 2 sin2 x + 5 sin x – 3 = 0 is
(a) 4
(b) 6
(c) 1
(d) 2
Answer
A
Question: If tan(A – B) = 1, sec(A + B) = 2√3 , the smallest positive value of B is
(a) 25π/24
(b) 19π/24
(c) 13π/24
(d) 7π/24
Answer
D
Question: Value of sin 10° + sin 20° + sin 30° +……+sin360° is
(a) 1
(b) 0
(c) 2
(d) 1/2
Answer
B
Question: The value of expression sin θ + cos θ lies between
(a) –2 and 2 both inclusive
(b) 0 and √2 both inclusive
(c) – √2 and √2 both inclusive
(d) 0 and 2 both inclusive
Answer
C
Question: Value of 2 sin2 π/6 + cosec2 7π/6 · cos2 π/3 is m/m–1 .The value of ‘m’ is
(a) 3
(b) 2
(c) 4
(d) None of these
Answer
A
Question: The value of tan 19π/3 is √n . Value of ‘n’ is
(a) 1
(b) 2
(c) 3
(d) 5
Answer
C
Question: If √3 tan 2θ + √3 tan 3θ + tan 2θ tan 3θ = 1, then the general value of θ is
(a) nπ + π/5
(b) (n + 1/6)π/5
(c) (2n ± 1/6 )π/5
(d) (n + 1/3)π/5
Answer
B
Question: The most general value of θ satisfying the equations sin θ = sin α and cos θ = cos α is
(a) 2nπ + α
(b) 2nπ – α
(c) nπ + α
(d) nπ – α
Answer
A
Question: Find the distance from the eye at which a coin of a diameter 1 cm be placed so as to hide the full moon, it is being given that the diameter of the moon subtends an angle of 31′ at the eye of the observer.
(a) 110 cm
(b) 108 cm
(c) 110.9 cm
(d) 112 cm
Answer
C
Question: cot2π/6 + cosec 5π/6 + 3 tan2π/6 is equal to
(a) 1
(b) 5
(c) 3
(d) 6
Answer
D
Question: The minute hand of a watch is 1.5 cm long. How far does its tip move in 40 minutes? (Use π = 3.14)
(a) 2.68 cm
(b) 6.28 cm
(c) 6.82 cm
(d) 7.42 cm
Answer
B
Question: Solution of the equation 3 tan(θ – 15) = tan(θ + 15) is
(a) θ = nπ/2 + (–1)n π/4
(b) θ = nπ + (–1)n π/3
(c) θ = nπ – π/3
(d) θ = nπ – π/4
Answer
A
Question:Match the following in column-I with the given in column-II.
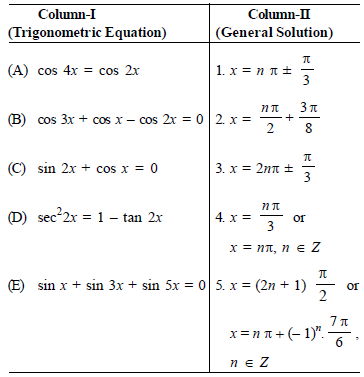
Codes:
A B C D E
(a) 4 3 5 1 2
(b) 4 3 5 2 1
(c) 3 4 5 1 2
(d) 1 3 5 2 4
Answer
B
Question: If sec2 θ = 4/3, then the general value of θ is
(a) 2nπ ± π/6
(b) nπ ± π/6
(c) 2nπ ± π/3
(d) nπ ± π/3
Answer
B
Question: Find x from the equation:
cosec (90° + θ) + x cos θ cot (90° + θ) = sin (90° + θ).
(a) cot θ
(b) tan θ
(c) –tan θ
(d) –cot θ
Answer
B

