Worksheets For Class 10 Mathematics Triangles

Class 10 Triangles Worksheet have been designed as per the latest pattern for CBSE, NCERT and KVS for Grade 10. Students are always suggested to solve printable worksheets for Mathematics Triangles Grade 10 as they can be really helpful to clear their concepts and improve problem solving skills. We at worksheetsbag.com have provided here free PDF worksheets for students in standard 10 so that you can easily take print of these test sheets and use them daily for practice. All worksheets are easy to download and have been designed by teachers of Class 10 for benefit of students and is available for free download.

Mathematics Triangles Worksheets for Class 10

We have provided chapter-wise worksheets for class 10 Mathematics Triangles which the students can download in Pdf format for free. This is the best collection of Mathematics Triangles standard 10th worksheets with important questions and answers for each grade 10th Mathematics Triangles chapter so that the students are able to properly practice and gain more marks in Class 10 Mathematics Triangles class tests and exams.

Chapter-wise Class 10 Mathematics Triangles Worksheets Pdf Download

Question. Find x and if DE || BC.   

(A) 1
(B) 2
(C) 3
(D) 4

Question. In a triangle ABC, D is the mid-point of AB, E is the mid-point of DB and F is the midpoint of BC. If the area of ΔABCis 96, then the area of ΔAEF is ………..   
(A) 16
(B) 24
(C) 32
(D) 36

Question. Find y if DE || BC.   

(A) 250
(B) 300
(C) 40
(D) 45

In fig., a rectangle is divided into four triangles x, y, z, w. The ratio of area x to area y is 2 : 3 and the ratio of area y to area z is 2 : 3. If area of w is 168cm2. Find the area of the rectangle.     

(A) 324cm2
(B) 624cm2
(C) 430cm2
(D) None of these

Question. In the given figure, AB = AC and CD || BA. The value of x is   

(A) 52⁰
(B) 76⁰
(C) 156⁰
(D) 104⁰

Question. If the two polygons are similar then find the value of x.   

(A) 6
(B) 16/3
(C) 17/3
(D) 8

Question. A kite got stuck on top of a 20 feet wall. A ladder is used by person to get the kite. It should be placed in such a manner that the top of ladder should rest on top of the wall and bottom of the ladder should be 15 feet away from the bottom of wall. Height of the ladder is:     
(A) 22 feet
(B) 20 feet
(C) 25 feet
(D)14 feet

Question. If AP and BP are the bisectors of the angle A and angle B of a parallelogram ABCD, then value of the angle APB is:   

(A) 30o
(B) 45o
(C) 60o
(D) 90 

Question. If ΔABC has ∠B = 90⁰ and D and E are points on BC where when connected to A, AD and AE trisects the angle A. Then     
(A) AE² = 3AC² /8 + 5AD²/8
(B) AC² = 3AE² /8 + 5AD²/8
(C) AC² = AE² = AD²
(D) none of these

Question. In the figure, PQ = QR = RS = SP = SQ = 6 cm and PT = RT = 14 cm. The length ST is: 

(A) 4√10 cm
(B) (7 √3 – 2) cm
(C) 10 cm
(D) 11 cm

Question. If ΔABCis a isosceles triangle where AB = BC and DE || BC, so if AD = 1.8 cm and CE = 5. 2 cm then AC is : 
(A) 5.2 cm
(B) 6 cm
(C) 8 cm
(D)7cm

Question. ABCD is a square of area of 4 square units which is divided into 4 non overlapping triangles as shown in figure, then sum of perimeters of the triangles so formed is     

(A) 8(2 + √2)
(B) 8(1 + √2)
(C) 4(2 + √2)
(D) 4(1 + √2)

Question. A ship started from the base of a light house. Height of the ship is 25 ft. The ship is travelling with a speed of 10 feet/sec. So after 5 seconds what is the length if the ship’s shadow on water if height of the light house is 50 ft.   
(A) 60 ft
(B) 50 ft
(C) 25 ft
(D) 100ft

Question. If DE || BC then   

(A) AB/AC = BC/BE
(B) AB/BC = DE/BE
(C) AC/BE = AB/AD
(D) none of these

Question. In the figure ∠D = 90⁰ AB =16 cm BC =12 cm and CA = 6cm then CD is 

(A) 13/6 cm
(B) 17/6 cm
(C) 19/6 cm
(D) 18/5cm

Question. ABCD is a quadrilateral in which zy = y/x = xw = k and k ϵ z. also {w, x} < 900 and {y, z}> 900, then the difference between the greatest angle and the smallest angle is:   
(A) 1280
(B) 1680
(C) 1010
(D) 990

Question. In a ΔABC,DE intersects AB and AC at point D and E respectively. If AB = 9, AD = 6, AE = 4, AC = 6 then line DE and BC are   
(A) same
(B) parallel
(C) perpendicular
(D) none of these

Question. Here BG || CD and FG || DE when which of the following are correct?     

(A) AC/BG = AE/DE
(B) AB/AC = AF/AE
(C) AD/AG = AC/AE
(D) none of these

Question. ABC is a triangle in which D, E and F are the mid-point of the sides of AC, BC and AB respectively . what is the ratio of the area of the shaded to the unshaded region in the triangle?

(A) 3 : 2
(B) 1 : 1
(C) 3 : 7
(D) None of these

Question. Here QN || LM and QO || LN, so which of the following is correct?   

(A) POxPN  = NMxON
(B) POxMN = PNxON
(C) ONxNL = OQ
(D) none of these

Question. In the given figure, ABCD is trapezium in which AB CD and its diagonals intersect at O. If AO= (3x –1) cm, OC = (5x –3) cm, BO = (2x+1) cm andOD(6x –5) cm, find the value of x   

(A) 1/2
(B) 3
(C) 4
(D) 2

Question. Let A by any point inside the rectangle KLMN. Then   
(A) KL² + LM = NM² + MA
(B) KA² + LM = KN² + AL
(C) AK² + AM = AL² + AN
(D) none of these

Question. If DG is the bisector of the angle ∠D in the ΔDEF and DE = 6, DF = 8, EF = 10, then EG =   
(A) 15/7
(B) 34/7
(C) 40/7
(D) 30/7

Question. There are two squares s1 and s2 with areas 8 and 9 square units, respectively s1 is inscribed within s2, with one comes of s1 on each side of s2. The corners of the smaller square divides the sides of the bigger square into two segments, one of the length a and the other of length b, where b>a. a possible value of b/a is. 
(A) ≥ 14 and < 17
(B) >17
(C) ≥ 11 and < 14
(D) None of these

Question. If two lines DE and LM bisects each other and if DM = 8 cm then EL is:   
(A) 8/3cm
(B) 4 cm
(C) 6 cm
(D) 8cm

Question. Here DR || HI || GF, HJ = 6 cm, JF = 9 cm, GI = 18 cm and GF = 12 cm then HI is:   

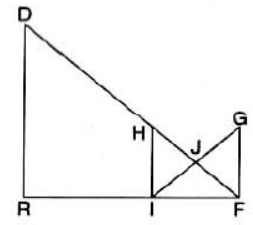
(A) 9 cm
(B) 8 cm
(C) 6 cm
(D) 10 cm

Question. If ΔABC ≅ ΔDEF. If ∠A = 3x – 60and ∠D = x + 20 then ∠A is:   
(A) 60
(B) 90
(C) 100
(D)

Question. Find x:   

(A) 11.4
(B) 10.9
(C) 11.6
(D)12.2

Question. If AB || CD then ΔABE and ΔDCEwill be   

(A) similar
(B) concreate
(C) equilateral
(D) cannot say

CBSE Class 10 Mathematics Triangles Worksheet Set A

CBSE Class 10 Mathematics Triangles Worksheet Set B

CBSE Class 10 Mathematics Triangles Worksheet Set C

CBSE Class 10 Mathematics Triangles Worksheet Set D

CBSE Class 10 Mathematics Triangles Worksheet Set E

CBSE Class 10 Mathematics Triangles Worksheet Set F

CBSE Class 10 Mathematics Triangles Worksheet Set G

CBSE Class 10 Mathematics Triangles Worksheet Set H

CBSE Class 10 Mathematics Triangles Worksheet Set I

CBSE Class 10 Mathematics Triangles Worksheets Set A

CBSE Class 10 Mathematics Triangles Worksheets Set C

CBSE Class 10 Mathematics Triangles Worksheets Set D

CBSE Class 10 Mathematics Triangles Worksheets Set E

Solved Problems on Class 10 Triangles

Question. In Fig.4.5, if LM||CB and LN||CD, prove that AM/AB=AN/AD

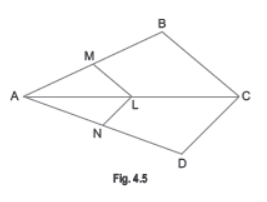
Sol. Firstly, in ΔABC, we have
LM||CB                                                          (Given)
Therefore, by Basic Proportionality Theorem, we haveAM/AB=AL/AC         …(i)
Again, in ΔACD, we have
LN||CD                                                           (Given)
∴ By Basic Proportionality Theorem, we have AN/AD=AL/AC                      …(ii)
Now, from (i) and (ii), we have AM/AB=AN/AD.

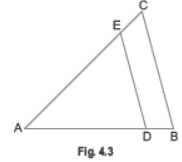
Question. In Fig.4.3, DE||BC. If AD = x , DB = x – 2, AE = x + 2 and EC = x -1, find the value of x.
Sol. In DABC, we have
DE||BC,
∴ AD/DB=AE/EC          [By Basic Proportionality Theorem]
⇒ x/x-2=x+2/x-1     ⇒   x(x-1)=(x_2)
⇒  x (x -1) = (x – 2) (x + 2)
⇒  x 2 – x = x² – 4   ⇒  x = 4

Question. If ABC and DEF are similar triangles such that ∠A = 47° and ÐE = 63°, then the measures of ∠C = 70°. Is it true? Give reason.
Sol. Since ΔABC ~ΔDEF
∴ ∠A= ÐD = 47°, ∠B= ÐE = 63°
∴ ∠C =180° -(∠A + ∠B) = 180 – (47 + 63) = 70°
∴ Given statement is true

Question. In Fig.4.6, DE||OQ and DF||OR, Show that EF||QR. 

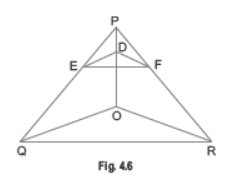
Sol. In ΔPOQ, we have
DE||OQ (Given)
∴ By Basic Proportionality Theorem, we have PE/EQ=PD/DO          …(i)
Again, in DPOR, we have
DF||OR (Given)
∴ By Basic Proportionality Theorem, we have PD/DO=PF/FR           …(ii)
Now, from (i) and (ii), we have
PE/EQ=PF/FR  ⇒ EF||QR
[Applying the converse of Basic Proportionality Theorem in DPQR]

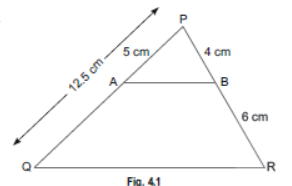
Question. A and B are respectively the points on the sides PQ and PR of a ΔPQR such that PQ =12 5 . cm, PA = 5 cm, BR= 6 cm and PB= 4 cm. Is AB||QR? Give reason.
Sol. PA/AQ =5/12.5-5 = 5/ 5 = 2/3
        PB/BR= 4/6=2/3
Since PA/AQ=PB/BR=2/3
∴ AB||QR

Question. In triangle PQR and MST, ∠P = 55°, ∠Q = 25°, ∠M =100° and ∠S = 25°. Is ΔQPR~ΔTSM? Why?
Sol. Since, ∠R =180°-(∠P + ∠Q) = 180° – (55° + 25°) = 100° = ÐM
∠Q = ∠S = 25°
ΔQPR ~ΔSTM
i.e., ΔQPR is not similar to ΔTSM.

Question. Prove that, if a line is drawn parallel to one side of a triangle to intersect the other two sides in distinct points, the other two sides are divided in the same ratio.
Using the above result, do the following:  In Fig. 4.2 DE||BC and BD = CE. Prove that DABC is an isosceles triangle.

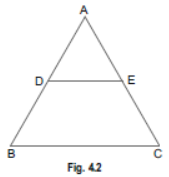
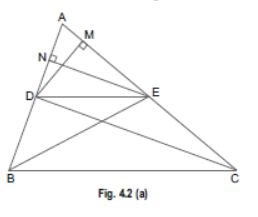
Sol. Given: A triangle ABC in which a line parallel to side BC intersects other two sides AB and AC at D and E respectively.
To Prove: AD/DB=AE/EC.
Construction: Join BE and CD and then draw DM^AC and EN^ AB.
Proof: Area of ΔADE = 1/2(basexhight)
So, ar (ADE) = 12/ AD x EN

Question.. E and F are points on the sides PQ and PR respectively of a ΔPQR. For each of the following cases, state whether EF||QR.
(i) PE = 4 cm, QE = 4.5 cm, PF = 8 cm and RF = 9 cm
(ii) PQ= 1.28 cm, PR= 2.56 cm, PE = 0.18 cm and PF = 0.36 cm

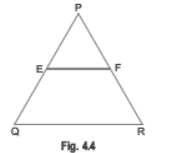
Sol. (i) We have, PE = 4 cm, QE = 4.5 cm
PF = 8 cm, RF = 9 cmNow, PE/QE= 4/4.5=8/9
And PF/RF= 8/9
Thus, PE/QE=PF/RF,                               Therefore, EF||QR. [By the converse of Basic Proportionality Theorem]
(ii) We have,
PQ =1.28 cm, PR = 2.56 cm
PE = 0.18 cm, PF = 0.36 cm
Now, QE = PQ – PE =1.28 – 0.18 =1.10 cm
and FR = PR – PF = 2.56 – 0.36 = 2.20 cm
Now, PE/QE=0.18/1.10 =18/.10=9/55.
and, PF/FR= 0.36/220 =36/220=9/55.            ∴ PE/QE=PF/FR
Therefore, EF||QR [By the converse of Basic Proportionality Theorem]

Question.. In Fig.4.7, A, B and C are points on OP, OQ and OR respectively such that AB||PQ and AC||PR. Show that BC||QR. 

Sol. In ΔOPQ, we have
AB||PQ                                                 (Given)
∴ By Basic Proportionality Theorem, we have
OA/AP=OB/BQ                                                   …(i)
Now, in DOPR, we have
AC||PR                                                (Given)
∴ By Basic Proportionality Theorem, we have
OA/AP=OC/CR                                                    …(ii)
From (i) and (ii), we have
OB/BQ=OC/CR
Therefore, BC||QR (Applying the converse of Basic Proportionality Theorem in ΔOQR)

Question. Using Basic Proportionality Theorem, prove that a line drawn through the mid-point of one side of a triangle parallel to another side bisects the third side. 

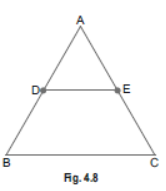
Sol. Given: A ΔABC in which D is the mid-point of AB and DE is drawn parallel to BC, which meets AC at E.
To prove: AE = EC
Proof: In ΔABC, DE||BC
∴ By Basic Proportionality Theorem, we have
AD/DB=AE/EC                                   …(i)
Now, since D is the mid-point of AB
⇒ AD = BD                                        …(ii)
From (i) and (ii), we have
BD/BD = AE/EC       ⇒      1 = AE/EC
⇒   AE = EC
Hence, E is the mid-point of AC.

Question. Using converse of Basic Proportionality Theorem, prove that the line joining the mid-points of any two sides of a triangle is parallel to the third side.

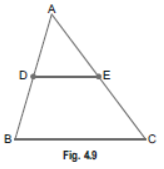
Sol. Given: DABC in which D and E are the mid-points of sides AB and AC respectively.
To prove: DE||BC
Proof: Since, D and E are the mid-points of AB and AC respectively
∴ AD = DB                         and             AE=EC
⇒ AD/DB=1                       and         AE/EC=1
⇒ AD/DB=AE/EC
Therefore, DE||BC (By the converse of Basic Proportionality Theorem)

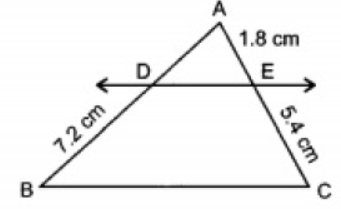
Question. In the given figure, DE ll BC. Find the length of side AD, given that AE = 1.8 cm, BD = 7.2 cm and CE = 5.4 cm.

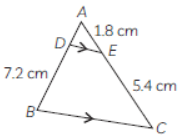
Solution. Given: A Δ ABC, in which DE || BC
AE = 1.8 cm, CE = 5.4 cm
and BD = 7.2 cm
To Find: Length of side AD.
Proof: As DE || BC, then by thales theorem
AD/BD = AE/QE
⇒AD/72 =1.8/5.4
⇒ AD = 7.2 x 1.8 / 5.4 = 2.4 cm
Hence, the value of AD is 2.4 cm.

Question. It is given that ΔDEF ∼ ΔRPQ. Is it true to say that ∠D = ∠R and ∠F = ∠P ? Why?
Solution. No.
Explanation:
We know that when ΔDEF ∼ ΔRPQ, each angle of triangle ΔDEF is equal to the corresponding angle of ΔRPQ. So,
∠D = ∠R: ∠E = ∠P. ∠ F = ∠Q
⇒ ∠D = ∠R is true but ÐF ≠ ÐP.
Hence, it is not true that ∠D = ∠R and ∠F = ∠P.

Question. In the given figure, DABC is an isosceles triangle right angled at C with AC = 4 cm. Find the length of AB.

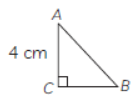
Solution. Given: A Δ ABC, right angled at C and AC = BC.
To Find: Length of AB.

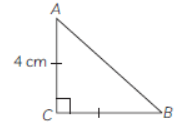
Proof: Δ ABC is an isosceles triangle
: AC = BC
= 4 cm
By applying the pythagoras theorem, we have
AB² = AC² + BC²
= 42 + 42
= 32
∴ AB = √32 = 4 √2 cm
Hence, the length of AB is 4√2 cm.

Question. The ratio of the corresponding altitudes of two similar triangles is 3/5. Is it correct to say that the ratio of their areas is 6/5? Why?
Solution. No.
It is given that the ratio of altitudes of similar triangles is 3/5.
Using the property of area of two similar triangles, we have
Area 1/Area 2 = Altitude 1²/Altitude 2² = (Altitude1 /Altitude2)²
Area 1/Area 2 = (3/5)2
Area 1 / Area 2 = 9/25
9/25 ≠ 6/5
Hence, the given statement is not correct because it does not satisfy the criteria.

Question. BC and BDE are two equilateral triangles such that D is the mid-point of BC. Find the ratio of the areas of triangle ABC and BDE.
Solution. D is the mid-point of BC,

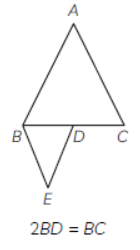
As ΔABC ∼ ΔBDE, because each triangle is an
equilateral triangle,
or (ΔABC) : or (ΔBDE) = BC² : BD²
= (2BD)² : BD² [by (i)]
= 4 : 1

Question. For a rhombus ABCD, prove the following: 4AB2 = AC² + BD² 
Solution. In a rhombus ABCD,

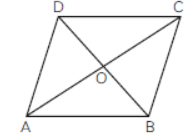
AO = CO = 1/2 AC BO = DO = 1/2 BD.
and ∠AOB = 90° [By Pythagoras theourm]
So, AB² = AO² + BO²
= (1/2 AC)² + (1/2 BD)²
⇒ 4 AB² = AC² + BD²

Question. A and B are respectively the points on the sides PQ and PR of a ΔPQR such that PQ = 12.5 cm, PA = 5 cm, BR = 6 cm and PB = 4 cm. Is AB ll QR? Give reasons for your answer.
Solution. Yes. 36
According to the question,
PQ = 12.5 cm PA = 5 cm
BR = 6 cm PA = 4 cm
⇒ QA = QP – PA = 12.5 – 5 = 7.5 cm
⇒ PA/AQ = 5/7.5 = 50/75 = 2/3
⇒ PB/BR = 4/6 = 2/3
From eqn. (i) and (ii), we get
PA/AQ = PB / BR
By the converse of basic proportionality theorem, if a line divides any two sides of a triangle in the same ratio, then the line is parallel to the third side.
Hence, AB ll QR.

Question. The perimeters of two similar triangles are 30 cm and 20 cm, respectively. If one side of the first triangle is 9 cm long, find the length of the corresponding side of the second triangle.   
Solution. Let the triangles be DABC and ΔPQR.  
Now, ΔABC ∼ ΔPQR

Question. Let Δ ABC ∼ Δ DEF and their areas be 64 cm2 and 121 cm² respectively. If EF = 15.4 cm, find BC.
Solution. Given,
ΔABC ~ ΔDEF
and ar (ΔABC) = 64 cm2
ar (ΔDEF) = 121 cm2
EF = 15.4 cm
ar(ΔABC)/ar(ΔDEF) = BC2/EF²
[Since, the ratio of areas of similar triangles is equal to the square of the corresponding sides] 37

Question. In the given figure DE ll BC, AD = 1 cm and BD = 2 cm. What is the ratio of the area (Δ ABC) to the area (Δ ADE)?   

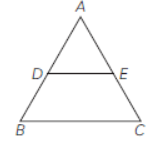
Solution.

Question. Is the triangle with sides 25 cm, 5 cm and 24 cm a right triangle? Give reasons for your answer.
Solution. No.
According to the question,
a = 25 cm b = 5 cm c = 24 cm
By Pythagoras theorem, we have,
a² = b² + c²
= 52 + 242
= 25 + 576 = 601
But a² = 625
625 ≠ 601
Since the sides do not satisfy the property of Pythagoras theorem, we can say that a triangle with sides 25cm, 5cm and 24cm is not a right triangle.

Question. The area of two similar triangles are 25 sq. m and 121 sq. cm. Find the ratio of their corresponding sides.
Solution. Given, Area (Δ1) = 25 sq. m, Area (Δ2) = 121 sq,

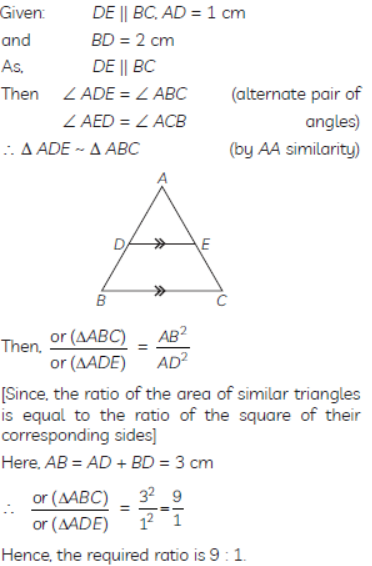
Question. In the given figure, if ∠D = ∠C, then it is true that ΔADE ∼ ACB? Why?

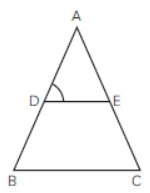
Solution. Yes,
In ΔADE and ΔACB,
∠D = ΔC
∠A = ∠A [Common angle]
⇒ ΔADE ∼ ΔACB [By AA similarity criterion]

Question. Is the following statement true? Why?
“Two quadrilaterals are similar, if their corresponding angles are equal”. 
Solution. No. Two quadrilaterals will be similar, if their corresponding angles are equal and ratio of corresponding sides must also be proportional.
eq. : rectangle, square

Question. In the figure, if ∠ACB = ∠CDA, AC = 6 cm and AD = 3 cm, then find the length of AB

Solution. ΔACB∼ΔADC (AA criterion)
⇒ AC/AD = AB /AC

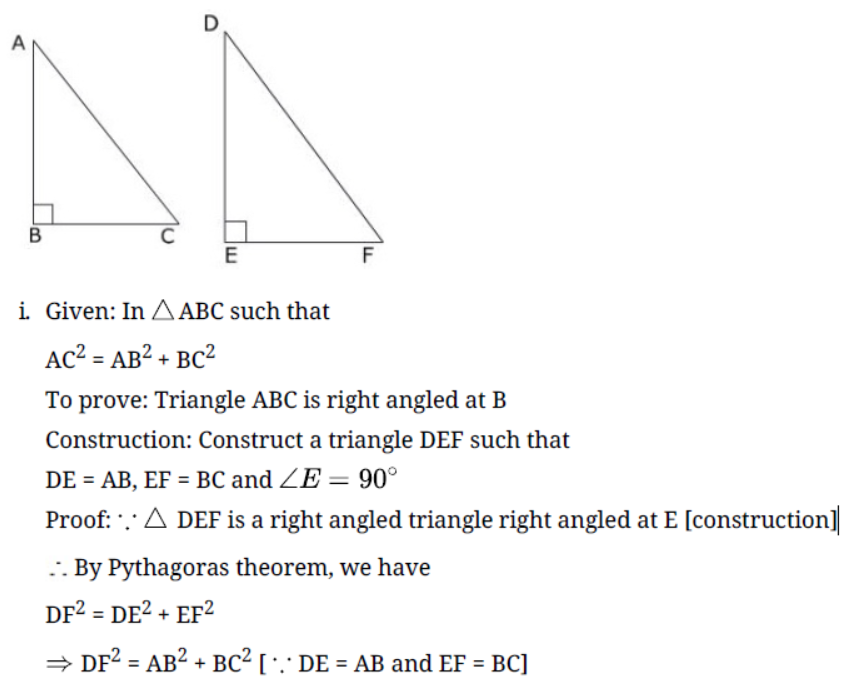
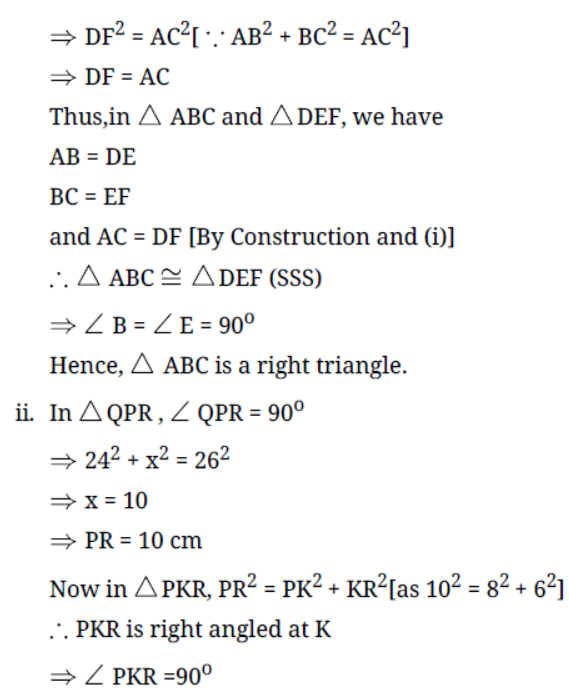
Question. In a triangle, if the square of one side is equal to the sum of the squares on the other two sides. Prove that the angle opposite to the first side is a right angle. Use the above theorem to find the measure of ∠ PKR in the figure given below.

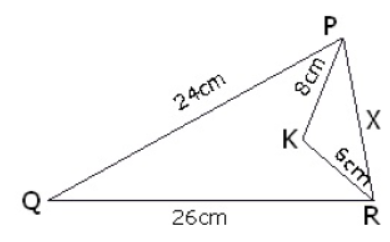
Solution.

Question. A ladder is placed in such a way that its foot is at a distance of 5 m from a wall and its tip reaches a window 12 m above the ground. Determine the length of the ladder.
Solution. Let AC be the ladder, AB be the wall and BC be the distance of ladder from the foot of the wall.
In right Δ ABC,

⇒ AC² = AB² + BC²{ using Pythagoras theorm for right-angled triangle}
⇒ AC² = (12)² + 52
⇒ AC² = 144 + 25
⇒ AC = 13 m

Question. InΔ ABC, X is any point on AC. If Y, Z, U and V are the middle points on AX, XC, AB and BC respectively, then prove that UY || VZ and UV || YZ.

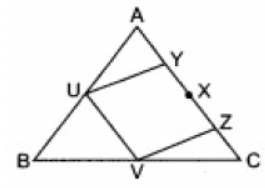
Solution. Join BX
In ABX, U is midpoint of AB and Y is mid-point AX (given)
∴ UY ll BX (using mid-point theorem) …..(i)

In BCX, v is mid-point of BC and z is mid-point of XC
VZ ll BX ..(ii)
from (i) and (ii)
UY ll VZ
In ABC, U is mid-point of AB and V is mid-point of BE.
∴ UV ll AC
⇒ UV ll YZ Hence proved.

Question. If the angles of one triangle are respectively equal to the angles of another triangle, Prove that the ratio of their corresponding sides is the same as the ratio of their corresponding angle bisectors.
Solution.

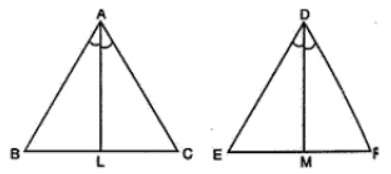
Given: Two triangles ABC and DEF in which ∠A = ∠D, ∠B = ∠E and ∠C = ∠F, AL and
DM are angle bisectors of A
and ∠D respectively

Question. In aΔ ABC, D and E are points on the sides AB and AC respectively such that DE || BC. If AD = x, DB = x-2, AE = x + 2 and EC = x – 1, find the value of x.
Solution. We have,

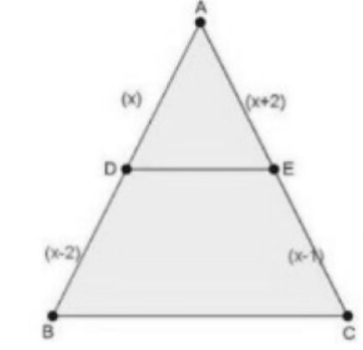
DE || BC
Therefore, by basic proportionality theorem,
We have,

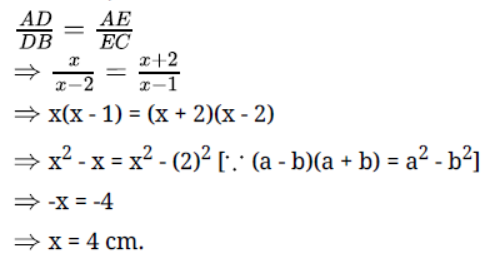
Question. In the given figure, ST || RQ, PS = 3 cm and SR = 4 cm. Find the ratio of Δ the area of PST to the area of Δ PRQ.

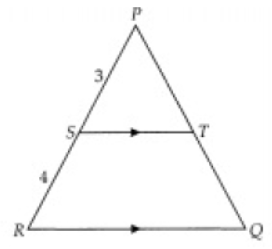
Solution. PS = 3 cm, SR = 4 cm and ST || RQ.

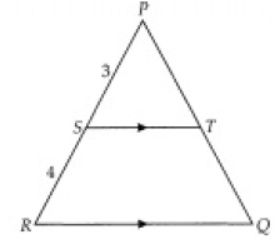
PR = PS + SR
= 3 + 4 = 7 cm

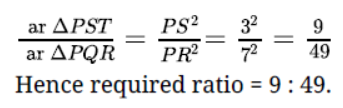
Question. If D and E are points on the sides AB and AC respectively of ΔABC such that AB = 5.6 cm, AD = 1.4 cm, AC = 7.2 cm and AE = 1.8 cm, show that DE ||BC.

Solution. Given: AB = 5.6 cm, AD = 1.4 cm, AC = 7.2 cm and AE = 1.8 cm

Hence, by the converse of Thales’ theorem, DE ll BC .

Question. In the given figure A, B and C are points on OP, OQ and OR respectively such that AB ll PQ and AC PR. Prove that BC QR.

Solution.

Question. Triangles ABC and DEF are similar. If AC = 19 cm and DF = 8 cm, find the ratio of the area of two triangles.
Solution. We have,
ΔABC ~ ΔDEF
AC = 19 cm and DF = 8 cm
By area of similar triangle theorem

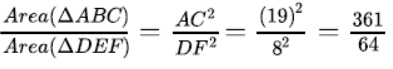
Question. In aΔ ABC, D and E are points on the sides AB and AC respectively such that DE ll BC. If AD = 8x – 7, DB = 5x – 3, AE = 4x – 3 and EC = (3x -1), find the value of x.
Solution. We have,

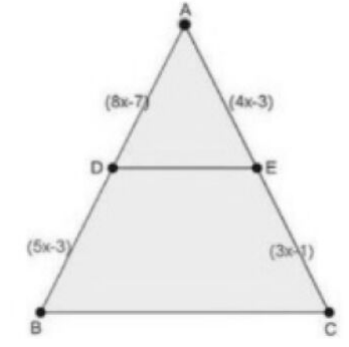
We are given that, DE || BC
Therefore, by thales theorem,
We have,

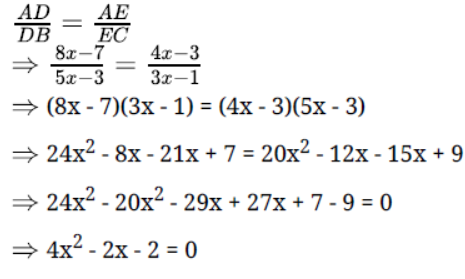
Question. In Fig. find F.

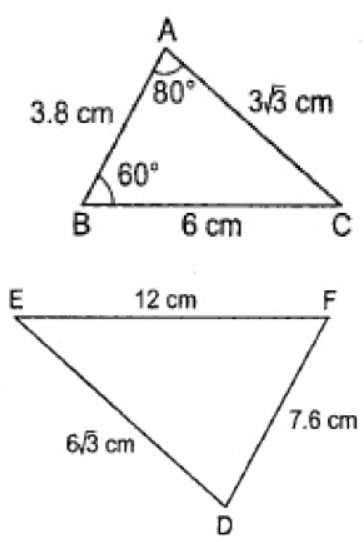
Solution. In triangles ABC and DEF, we have

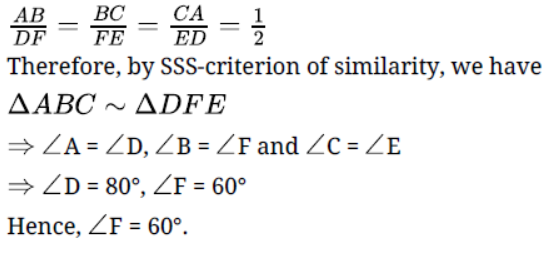
Question. In the given figure, DE BC. Find AD.

Solution.

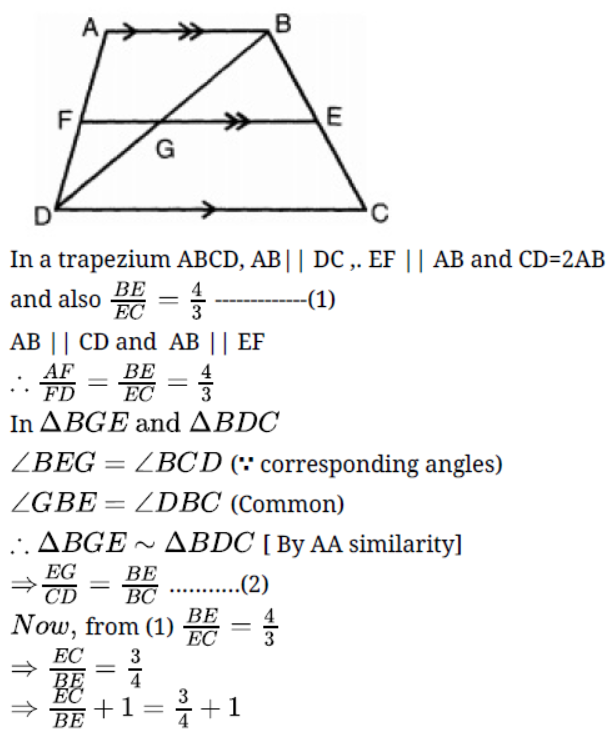
Question. In a trapezium ABCD, AB | | DC and DC = 2AB. EF | | AB, where E and F lie on BC and AD respectively such that BE/EC = 4/3. Diagonal DB intersects EF at G. Prove that, 7EF =11AB.
Solution.

Question. A man goes 10m due south and then 24m due west. How far is he from the starting point?
Solution. Starting from O, let the man goes from O to A and then A to B as shown in the figure.
Then,
OA = 10m, AB = 24m and OAB = 90^o

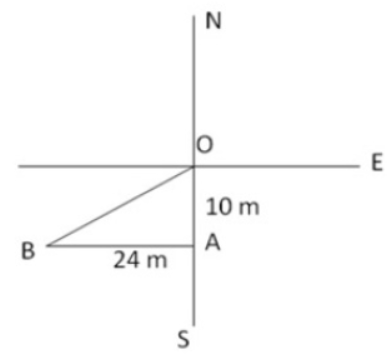
Using Pythagoras theorem:
⇒ OB² = OA² + AB²
⇒ OB² = 102 + 242
⇒ OB² = 100 + 576
⇒ OB² = 676
⇒ OB = √676 = 26m
Hence, the man is 26m south-west from the starting position.

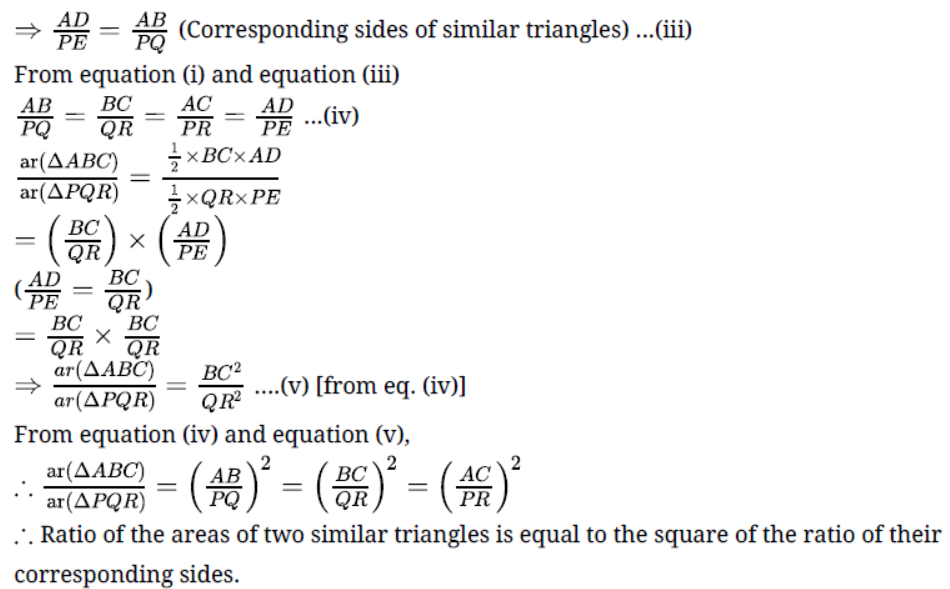
Question. Prove that ratio of the areas of two similar triangles is equal to the square of the ratio of their corresponding sides.
Solution.

Question. In the figure of Δ ABC, the points D and E are on the sides CA, CB respectively such that DE AB, AD = 2x, DC = x + 3, BE = 2 x – 1 and CE = x. Then, find x.

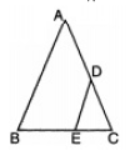
Solution. DE ll AB
AD = 2x, DC = x + 3, BE = 2 x – 1 and CE = x
By Basic proportionality theorem
CD/AD = CE/BE
x + 3 /2x = x / 2x – 1
(x + 3) (2x – 1) = x(2x)
2x² – x + 6x – 3 = 2x²
2x² + 5x – 3 = 2x²
5x – 3 = 0
or, 5x = 3
x = 3/5

Question. In figure, D and E are points on AB and AC respectively, such that DE BC. If AD = 1/3 BD, AE = 4.5 cm, find AC.

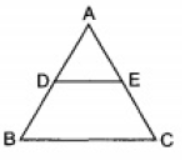
Solution. According to question it is given that D and E are the points on sides AB and AC respectively
Also AD 1/3 = BD,
AE = 4.5 cm, DE ll BC

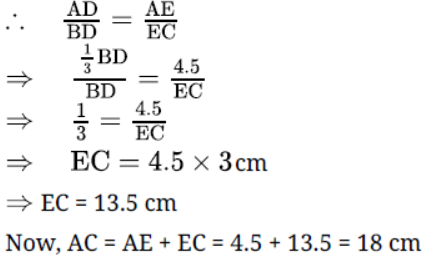
Question. InΔABC shown below, DE || BC If BC = 8 cm , DE = 6 cm and area of ΔADE = 45 cm² , What is the area of ΔABC ?

Solution.

Question. In three line segments OA, OB, and OC, points L, M, N respectively are so chosen that LM ll AB and MN ll BC but neither of L, M, N nor of A, B, C are collinear. Show that LN ll AC .
Solution. We have,

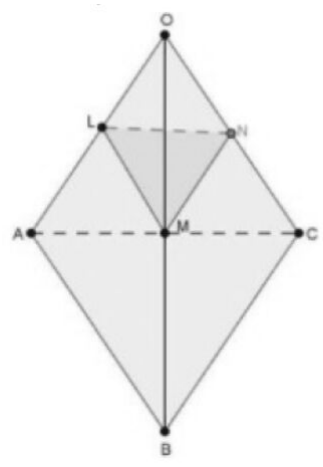
LM || AB and MN || BC
Therefore, by basic proportionality theorem,
We have,

Thus, LN divides sides OA and OC Δ OAC in the same ratio.
Therefore, by the converse of basic proportionality theorem, we have,
LN||AC.

Question. In the given figure , AP = 3cm , AR = 4.5 cm, AQ = 6 cm ,AB = 5 cm and AC = 10 cm , then find AD and the ratio of areas of ΔAQR and ΔADC .

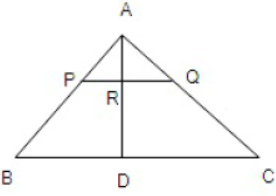
Solution. Given , AP = 3cm, AR = 4.5 cm, AQ = 6 cm, AB = 5 cm and AC = 10 cm

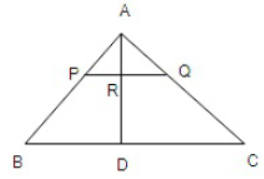
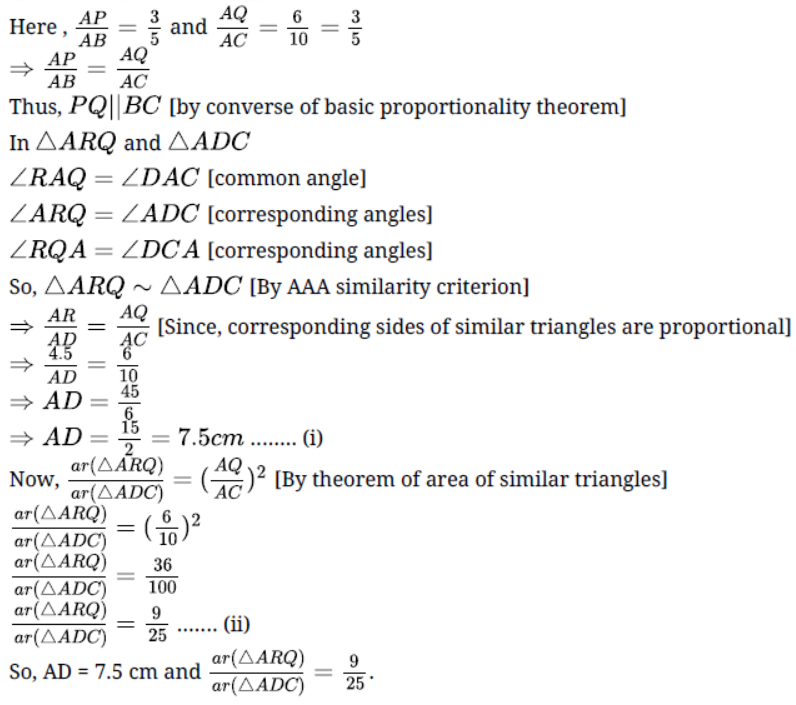
Question. In Δ ABC, if X and Y are points on AB and AC respectively such that AX/XB = 3/4 , AY = 5 and YC = 9, then state whether XY and BC are parallel or not. 
Solution.

Question. In Fig. M = N = 46°. Express x in terms of a, b and c where a, b, c are lengths of LM, MN and NK respectively.

Solution. In ΔKPN and ΔKLM, we have
∠KNP = KML = 46° [Given]
∠NKP = MKL [Common]
Thus, ΔKPN ~ ΔKLM [by AA similarity criterion of triangles]
KN/KM = NP/ML [because we know that corresponding sides of similar triangles are proportional]

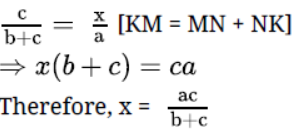
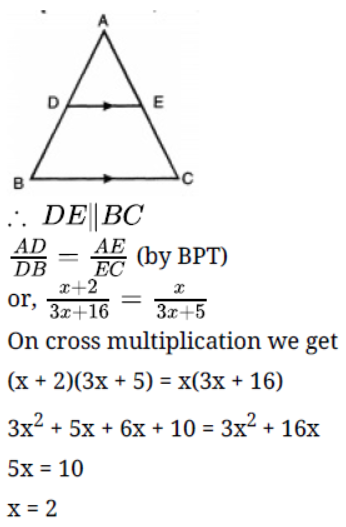
Question. In ΔABC , DE ll BC If AD = x + 2, DB = 3x + 16, AE = x and EC = 3x + 5, then find x.
Solution.

Question. In the fig PQ BC and AP: PB = 1:2. Find ar(ΔAPQ)/ar(ΔABC) .

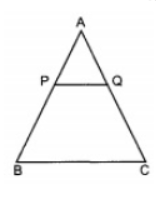
Solution. In ABC,
PQ ll BC
∴ AP/AB = AQ/AC
Now in ΔAPQ and ΔABC,

Since for similar triangles,the ratio of the areas is the square of the ratio of their corresponding sides. Therefore,

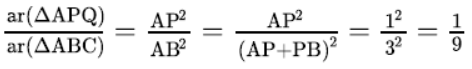
Question. In Fig. if EF ll DC ll AB . prove that AE/ED = BF/FC .

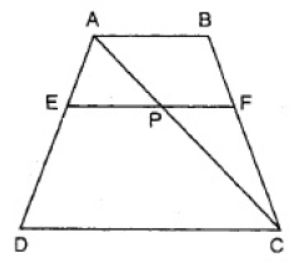
Solution. Given: According to the question,We have EF ll DC ll AB, in the given figure.
To prove: AE/ED = BE / FC

Construction: Produce DA and CB to meet at P(say).
Proof: In Δ PEF, we have

Question. If the altitude of two similar triangles are in the ratio 2 : 3, what is the ratio of their areas? 
Solution. We know that the ratio of areas of two similar triangles is equal to the square of the ratio of corresponding altitude.
Ratio of their areas = (ratio of their altitudes)²
= (2/3)2 = 4/9
= 4 : 9

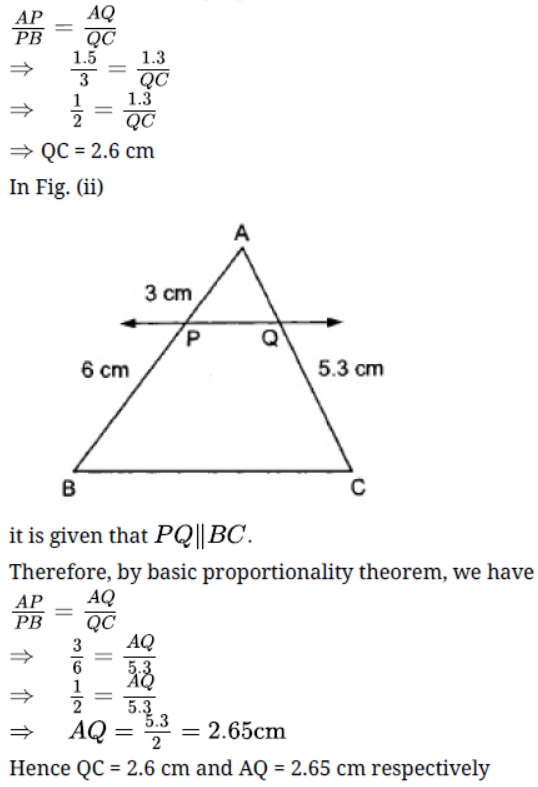
Question. In the given figure, DB ⊥ BC , DE ⊥ AB and AC ⊥ BC . Prove that BE/DE = AC/BC .

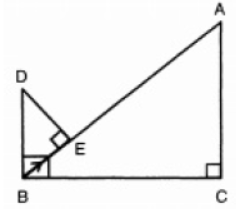
Solution. Given:

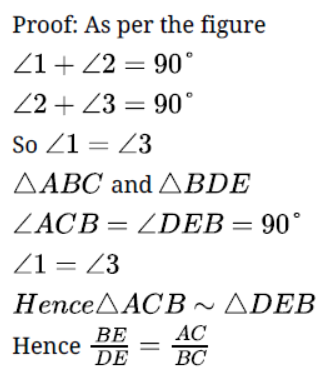
Question. In Fig. (i) and (ii), PQ ll BC . Find QC in (i) and AQ in (ii).

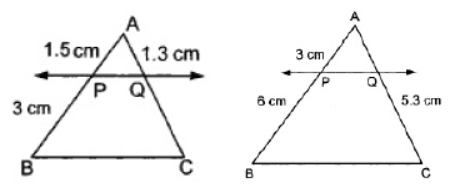
Solution. According to question

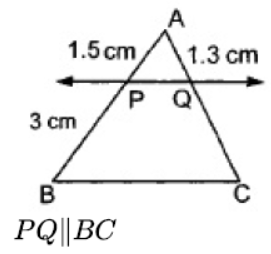
Therefore, by basic proportionality theorem, we have

Question. For going to a city B from city A, there is a route via city C such that AC ⊥ CB, AC = 2x km and CB = 2(x + 7) km. It is proposed to construct a 26 km highway which directly connects the two cities A and B. Find how much distance will be saved in reaching city B from city A after the construction of the highway.
Solution. A.T.Q.
Let AC = 2x km
CB = 2(x + 7) km

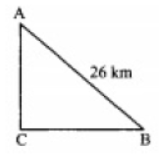
In right-angled Δ ACB, AB² = AC² + CB²
Highway is AB = 26 km
(26)² = (2x)² + (2(x + 7))²
⇒ 676 = 4x² + 4(x +7)²
⇒ 676/4= x² + x² + 49 + 14x
⇒ 169 = 2x² + 14x + 49
⇒ 2x² + 14x + 49 – 169 = 0
⇒ 2x² + 14x – 120 = 0
⇒ x² + 7x – 60 = 0
⇒ x² + 12x – 5x – 60 = 0
⇒ x(x + 12) – 5(x + 12) = 0
⇒ (x – 5)(x + 12) = 0
⇒ x = -12, 5
So, AC = 2x = 2(5) = 10 km
BC = 2(x + 7) = 2(5 + 7) = 24 km
Total distance for A to B via C = 10 + 24 = 34 km
A to B via highway = 26 km
Distance saved = 34 – 26 = 8 km.

Question. Find ∠P in the adjoining figure.

Solution.

Question. In two similar triangles ABC and PQR, if their corresponding altitudes AD and PS are in the ratio 4: 9, find the ratio of the areas of ΔABC and ΔPQR.
Solution. Theorem: ratio of areas of two similar triangles is equal to the ratio of the square of their corresponding altitudes.   
Here it is given that two triangles ABC and PQR are similar.

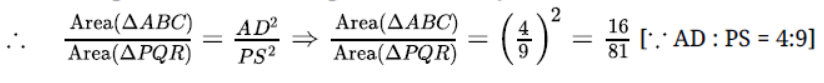
Question. In figure DE BC. If BD = x – 3, AB = 2x. CE = x – 2 and AC = 2x + 3. Find x.   

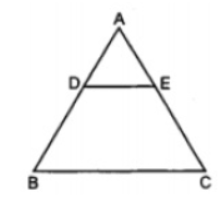
Solution. In ΔABC, DE || BC   
Therefore,by basic proportionality theorm,we have,

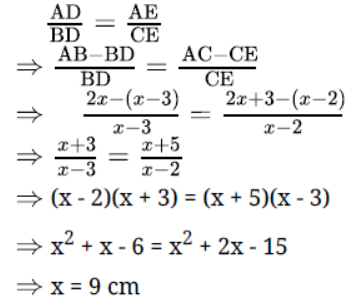
Question. D and E are points on the sides AB and AC respectively of a ΔABC . If AD = 7.2 cm, AE = 6.4 cm, AB = 12 cm and AC = 10 cm then determine whether DE || BC or not.   

Solution. Here, we use the converse proportionality theorm.   
Since D and E are points on the sides AB and AC respectively.

Hence, by the converse of Thales theorem DE is not Parallel to BC.

Question. In figure, DE || BC in Δ ABC such that BC = 8 cm, AB = 6 cm and DA = 1.5 cm. Find DE.   

Solution. Given DE || BC.

Question. If ratio of corresponding sides of two similar triangles is 5 : 6, then find ratio of their areas.
Solution. Let the triangles be ΔABC and ΔDEF   
Then the ratio of their area is =

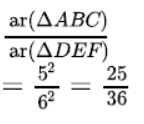
Question. Prove that the line segment joining the midpoints of any two sides of a triangle is parallel to the third side.
Solution.

According to question it is given that ABC is a triangle in which D and E are the midpoints of AB and AC respectively.
To Prove DE ll BC
Proof :- Since D and E are the midpoints of AB and AC respectively, we have AD = DB
and AE = EC.

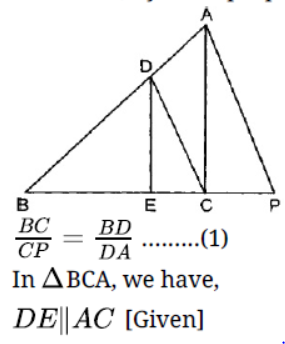
Question. In Fig. DE || AC and DC || AP. Prove that BE/EC = BC/CP.   

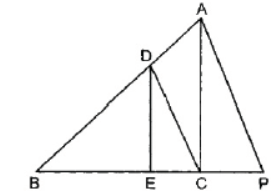
Solution. In ΔBPA, we have       
DC ll AP
Therefore, by basic proportionality theorem, we have ,

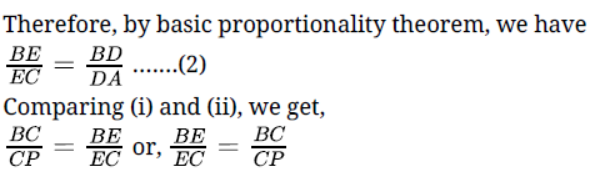
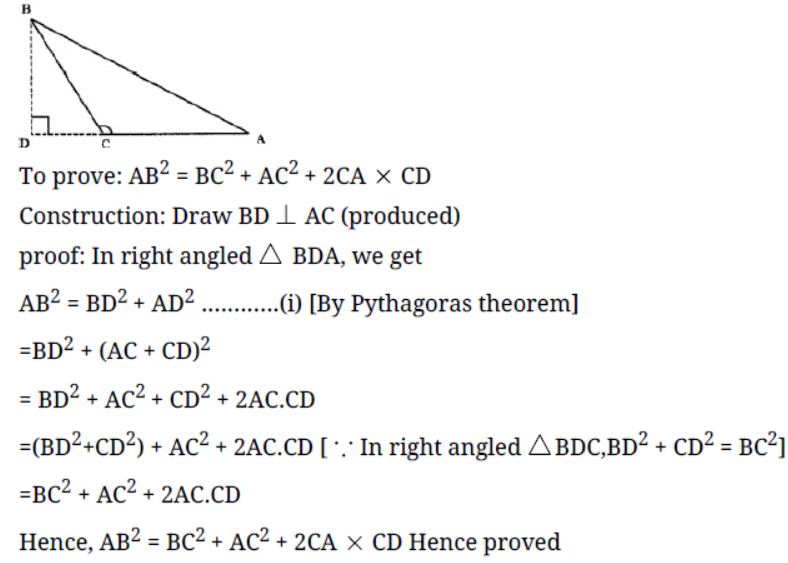
Question. In Δ ABC, C > 90o and side AC has produced to D such that segment BD is perpendicular to segment AD. Prove that AB² = BC² + AC² + 2CA x CD.
Solution. Given: ΔABC in which ΔACB > 90^o 

Question. In Fig. if AD⊥ BC and BD/DA = DA/DC , Prove that Δ ABC is a right triangle.

Solution. In Δ’s BDA and ADC, we have   

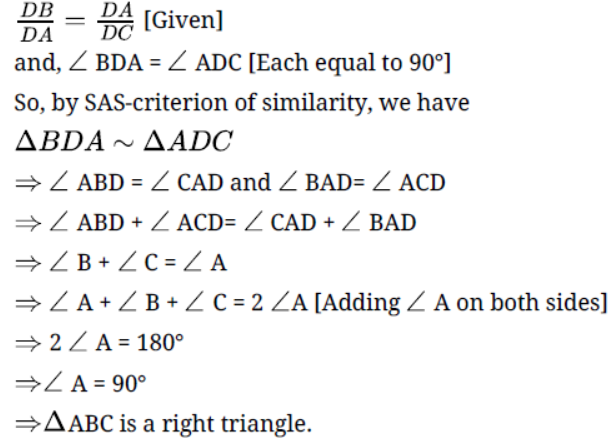
Question. In Δ ABC, AD is a median. Prove that AB² +AC² = 2 AD² + 2 DC².
Solution. 

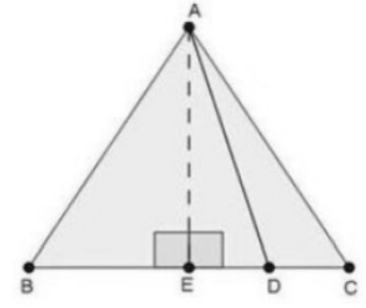
Construction:- Draw AE ⊥ BC     
Given, AD is a median
In ΔAED ,
By using pythagoras theorem, we get
DE² = AE² + AD²
AE² = AD² – DE² … (i)
In ΔAEB ,
By using pythagoras theorem, we get
AB² = AE² + BE²
⇒ AB² = AD² – DE² + (BD – DE)² [from (i)]
⇒ AB² = AD² – DE² + BD² + DE² – 2BD DE
⇒ AB² = AD² + CD² – 2CD x DE …(i) [BD = CD]
In  ΔAEC ,
By using pythagoras theorem, we get
AC² = AE² + EC²
⇒ AC² = AD² – DE² + (DE + DC)² [from (i)]
⇒ AC² = AD² – DE² + DE² + DC² + 2DE x DC
⇒ AC² = AD² + DC² + 2DE x DC …(ii)
Add equations (i) and (ii)
Therefore, AB² + AC² = 2AD² + 2CD²

Question. In the adjoining figure, ABC is a triangle in which AB = AC. If D and E are points on AB and AC respectively such that AD = AE, show that the points B, C, E and D are concyclic.

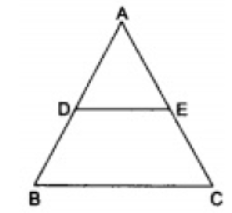
Solution. It is gIven that:
AD = AE ……………(i)
AB = AC ……………(ii)
Subtracting AD from both sides, we obtain
AB – AD = AC – AD
⇒ AB – AD = AC – AE (Since, AD = AE)
⇒ BD = EC ……………(iii)
Dividing equation (i) by equation (iii), we Obtain
AD/DB = AE/EC
Applying the converse of Thales’ theorem, we get DE ll BC .
⇒ ∠DEC + ∠ECB = 180° (Sum of interior angle on the same side of a Transversal line 180° .)
⇒ ∠DEC + ∠CBD = 180° (Since, AB = AC ⇒ ∠B = ∠C)
Hence, quadrilateral BCED is cyclic.
Therefore, B, C, E and D are concyclic points, which completes the proof.

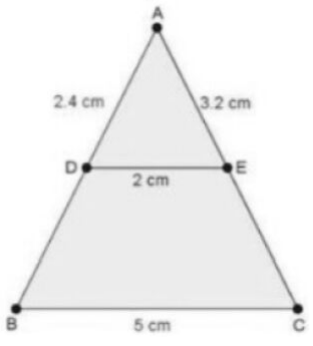
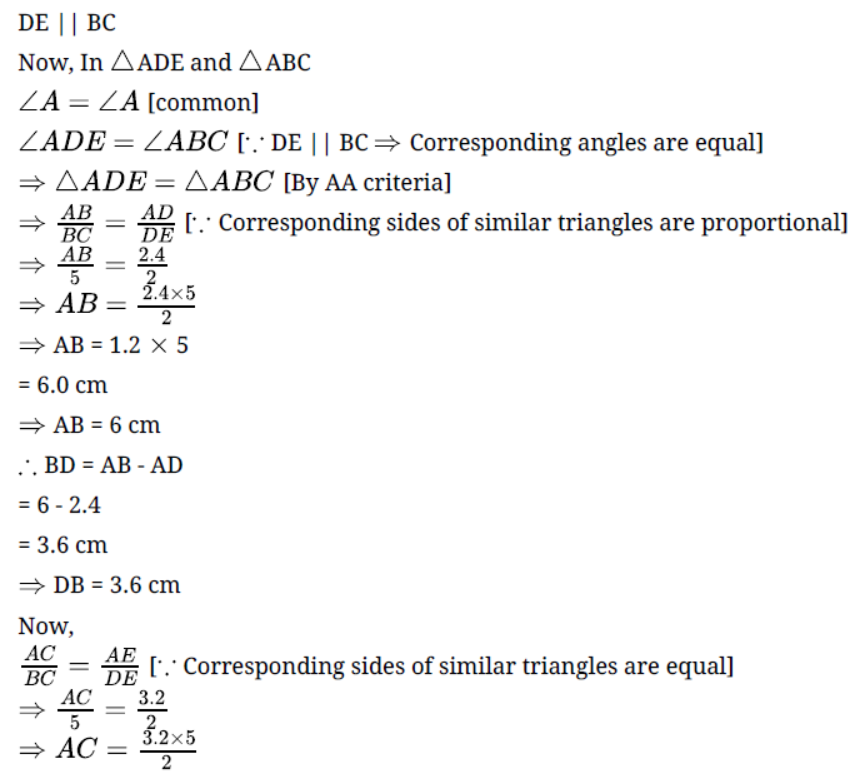
Question. In a Δ ABC, D and E are points on AB and AC respectively such that DE || BC. If AD = 2.4 cm, AE = 3.2 cm, DE = 2 cm and BC = 5 cm, find BD and CE.
Solution. We have,     

Question. Find the altitude of an equilateral triangle when each of its side is ‘a’ cm. 
Solution. 

Let the triangle be ABC.   
The altitude AD is also the median of equilateral ΔABC.so, BD = DC = a/2 . Let AD = h cm.
In right-angled ΔABD,
By Pythagoras theorem,we have

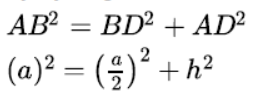
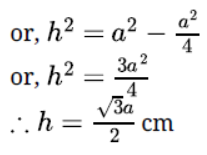
Question. In the given figure the line segment XY AC and XY divides triangular region ABC into two points equal in area, Determine AX/AB .

Solution. Since XY ll AC
∴ ∠BXY = ∠BAC
∠BYX = ∠BCA [Corresponding angles] 

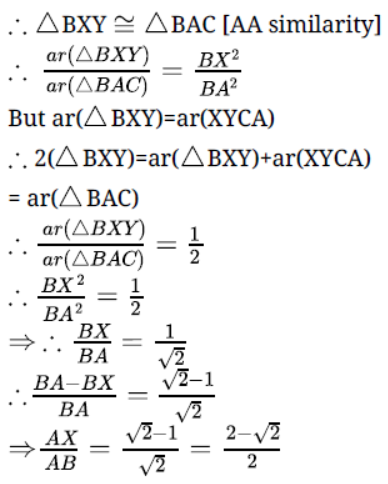
Question. Let s denote the semiperimeter of a triangle ABC in which BC = a, CA = b, AB = c. If a circle touches the sides BC, CA, AB at D, E, F, respectively, prove that BD = s-b.
Solution. According to the question ,
s = a + b + c/2 ⇒ 2s = a + b + c
B is an external point and BD and BF are tangents and from an external point the
tangents drawn to a circle are equal in length.
So, BD = BF; AF = AE; CD = CE … (i)   

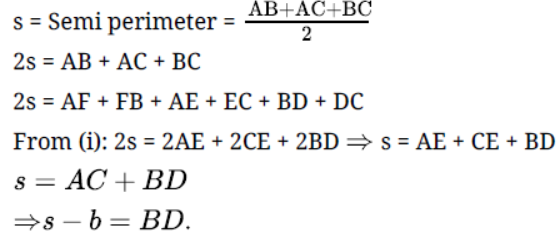
Question. From an airport, two aeroplanes start at the same time. If speed of first aeroplane due North is 500 km/h and that of other due East is 650 km/h then find the distance between the two aeroplanes after two hours. 
Solution. 

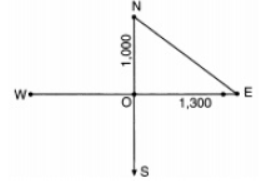
Distance covered by first aeroplane due North aftert two hours= ON = 500 x 2 = 1000 km
Distance covered by second aeroplane due East
after two hours OE = 650 X 2 = 1300 km
As per shown in the figure the distance between the aeroplane will be equal to NE,Now in ΔONE

Question. In the given figure, ΔABC ~ ΔDEF. If AB = 2DE and area of ΔABC is 56 sq. cm, find the area of ΔDEF.

Solution. ΔABC ~ ΔDEF
Since for similar triangles,the ratio of the areas is the square of the ratio of their corresponding sides.

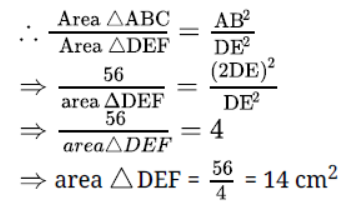
Question. In Fig. AD and BE are respectively perpendiculars to BC and AC. Show that ΔADC ∼ ΔBEC .

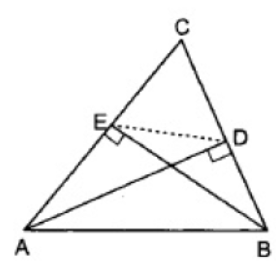
Solution. In Δ’s ADC and BEC, we have

Question. In ΔABC, P and Q are points on sides AB and AC respectively such that PQ ll BC. If AP = 4 cm, PB = 6 cm and PQ = 3 cm, determine BC.
Solutipon. Let BC = x cm

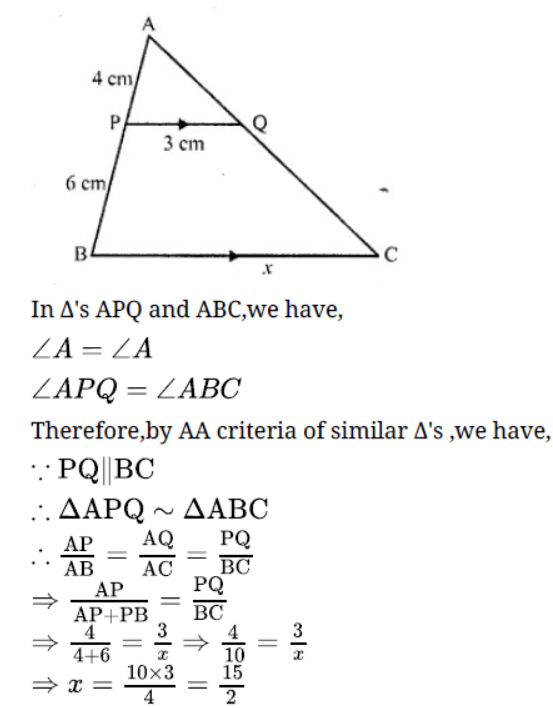
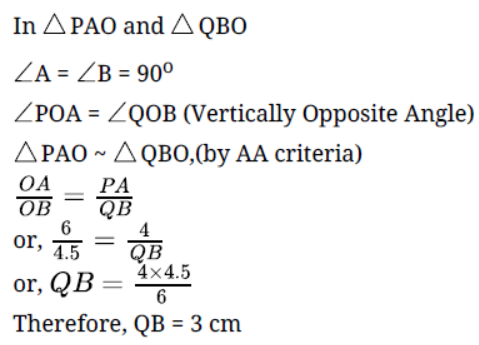
Question. In the given figure, if ∠A = 90o, ∠B = 90o, OB = 4.5 cm, OA = 6 cm and AP = 4 cm, then find QB.

Solution.

Question. In Fig. DEFG is a square and BAC = 90°. Prove that ΔAGF ∼ ΔDBG .

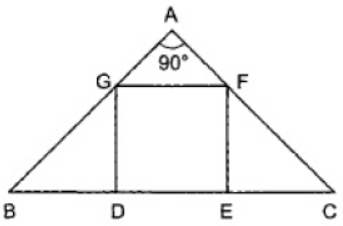
Solution. In ΔAGF and DBG, we have
∠GAF = ∠BDG [Each equal to 90°]
and, ∠AGF = ∠DBG [Corresponding angles]
ΔAGF ~ ΔDBG [By AA-criterion of similarity]

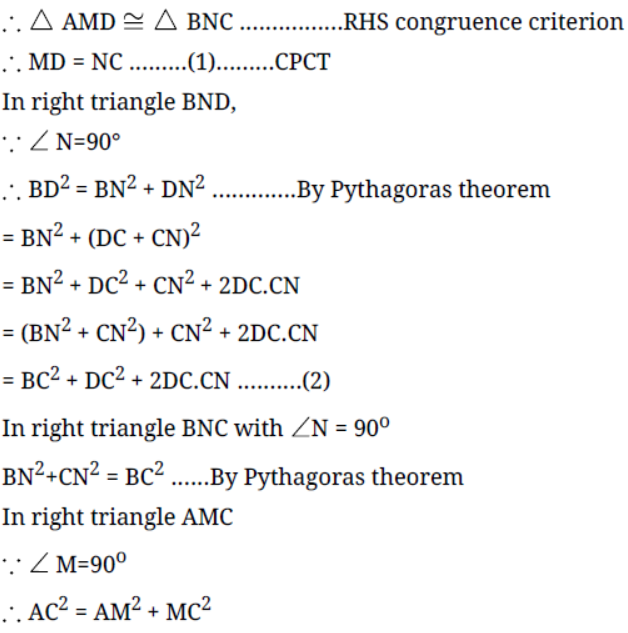
Question. Prove that the sum of the squares of the diagonals of a parallelogram is equal to the sum of the squares of its sides.
Solution. Given: ABCD is a parallelogram whose diagonals are AC and BD.
To prove: AB² + BC² + CD² + DA² = AC² + BD²
Construction: Draw AM ⊥ DC and BN ⊥ D(Produced)

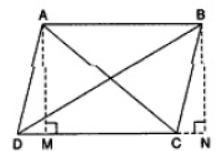
Proof: In right triangle AMD and BNC.
AD = BC …………..Opp.sides of a ||gm
AM = BN …………Both are altitudes of the same parallelogram to the same base

Question. ΔABD is a right triangle right-angled at A and AC ⊥ BD. Show that AB² =BC x BD .

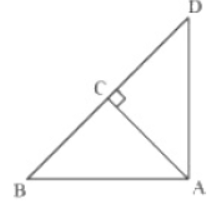
Solution. Given: ΔABD is a right triangle right-angled at A and AC ⊥ BD.
To Prove: AB² =BC x BD

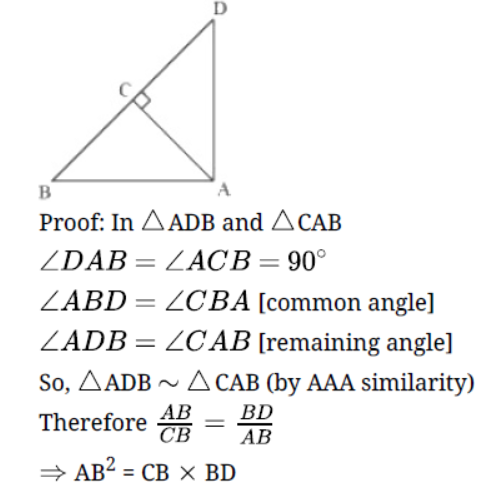
Question. In the given figure, ΔABC and ΔDBC are on the same base BC. If AD intersects BC at O, Prove that ar (ΔABC)/ar ΔDBC = AO/DO .

Solutipon. Given: ΔABC and ΔDBC are on the same base BC and AD intersects BC at O.

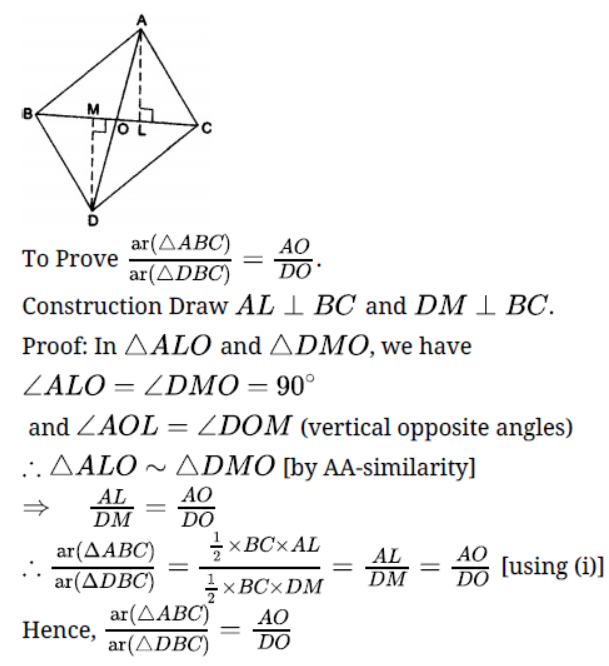
Question. In a quadrilateral ABCD, P,Q,R,S are the mid-points of the sides AB, BC, CD and DA respectively. Prove that PQRS is a parallelogram.
Solution. To Prove: PQRS is a parallelogram
Construction: Join AC
Proof: In ΔDAC

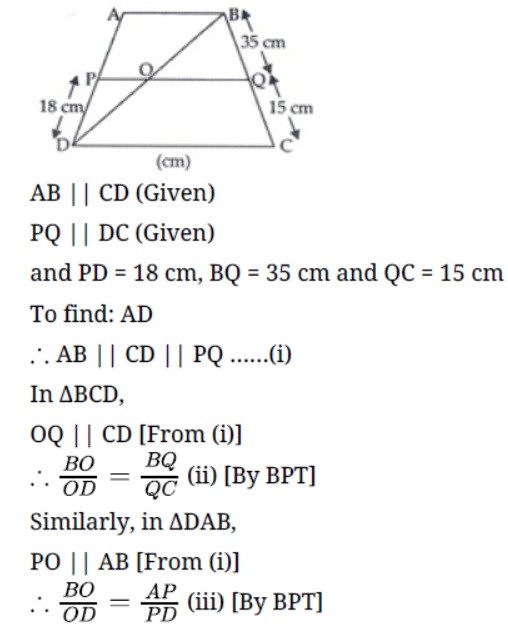
Question. ABCD is a trapezium in which AB || DC and P and Q are points on AD and BC, respectively such that PQ || DC. If PD = 18 cm, BQ = 35 cm and QC = 15 cm, find AD.
Solution. In trapezium ABCD

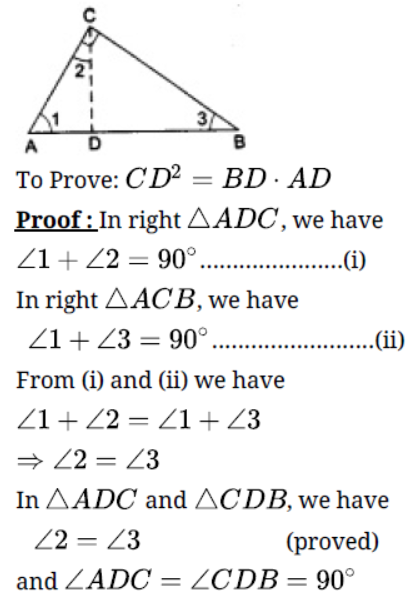
Question. In the given figure, ∠ACB = 90° and CD ⊥ AB . Prove that CD² = BD . AD .

Solution.

Question. If ΔABC ~ ΔDEF such that 2AB = DE and BC = 6 cm, find EF. 
Solution. Given that ΔABC ~ ΔDEF
We know that when two triangles are similar, then the ratios of the lengths of their corresponding sides are equal.

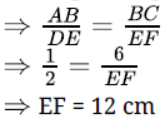
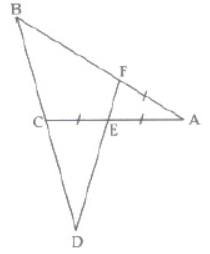
Question. In the given figure, line segment DF intersect the side AC of a triangle ΔABC at the point E such that E is the mid–point of CA and ∠AEF = ∠AFE. Prove that:
BD/CD = BF/CE . [Hint: Take point G on AB such that CG DF.]

Solution. To Prove: BD / CD = BE/CE

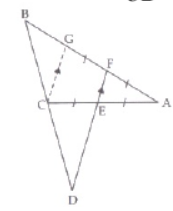
Construction: Draw CG ll EF.

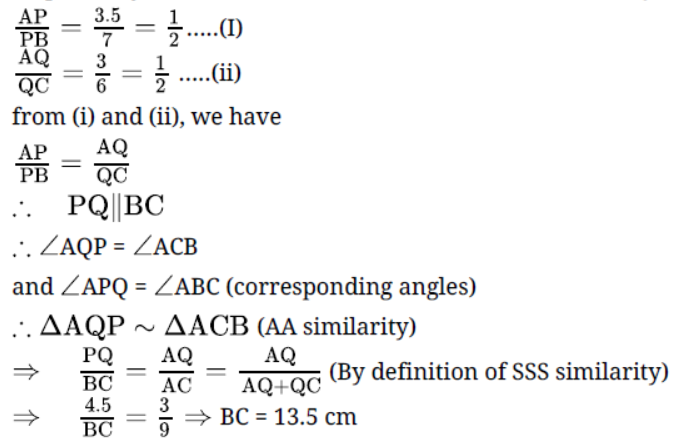
Question. In the fig., P and Q are points on the sides AB and AC respectively of ΔABC such that AP = 3.5 cm, PB = 7 cm, AQ = 3 cm and QC = 6 cm. Find BC.

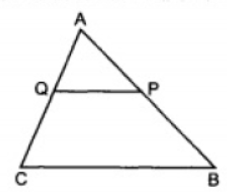
Solution. According to question it is given that P and Q are points on the sides AB and AC respectively of ΔABC such that AP = 3.5 cm, PB = 7 cm, AQ = 3 cm and QC = 6 cm.

Mathematics Triangles Worksheets for Class 10 as per CBSE NCERT pattern

Parents and students are welcome to download as many worksheets as they want as we have provided all free. As you can see we have covered all topics which are there in your Class 10 Mathematics Triangles book designed as per CBSE, NCERT and KVS syllabus and examination pattern. These test papers have been used in various schools and have helped students to practice and improve their grades in school and have also helped them to appear in other school level exams. You can take printout of these chapter wise test sheets having questions relating to each topic and practice them daily so that you can thoroughly understand each concept and get better marks. As Mathematics Triangles for Class 10 is a very scoring subject, if you download and do these questions and answers on daily basis, this will help you to become master in this subject.

Benefits of Free Class 10 Triangles Worksheet

1. You can improve understanding of your concepts if you solve NCERT Class 10 Mathematics Triangles Worksheet,
2. These CBSE Class 10 Mathematics Triangles worksheets can help you to understand the pattern of questions expected in Mathematics Triangles exams.
3. All worksheets for Mathematics Triangles Class 10 for NCERT have been organized in a manner to allow easy download in PDF format
4. Parents will be easily able to understand the worksheets and give them to kids to solve
5. Will help you to quickly revise all chapters of Class 10 Mathematics Triangles textbook
6. CBSE Class 10 Mathematics Triangles Workbook will surely help to improve knowledge of this subject

These Printable practice worksheets are available for free download for Class 10 Mathematics Triangles. As the teachers have done extensive research for all topics and have then made these worksheets for you so that you can use them for your benefit and have also provided to you for each chapter in your ebook. The Chapter wise question bank and revision worksheets can be accessed free and anywhere. Go ahead and click on the links above to download free CBSE Class 10 Mathematics Triangles Worksheets PDF.