Please refer to Complex Numbers and Quadratic Equations MCQ Questions Class 11 Mathematics below. These MCQ questions for Class 11 Mathematics with answers have been designed as per the latest NCERT, CBSE books and syllabus issued for the current academic year. These objective questions for Complex Numbers and Quadratic Equations will help you to prepare for the exams and get more marks.
Complex Numbers and Quadratic Equations MCQ Questions Class 11 Mathematics
Please see solved MCQ Questions for Complex Numbers and Quadratic Equations in Class 11 Mathematics. All questions and answers have been prepared by expert faculty of standard 11 based on latest examination guidelines.
MCQ Questions Class 11 Mathematics Complex Numbers and Quadratic Equations
Question: The point of intersection of the curves arg ( z – 3 i) =3π/4 and arg (2z+1- 2i )= π/4,(where i = √-1) is
(a)1/4(3+9i)
(b)1/4(3-9i)
(c)1/2(3+2i
(d) No point
Answer
D
Question:

Answer
A
Question: The complex number z satisfies the condition

Answer
C
Question: If |z – iRe ( z)|=|z – Im ( z)| (where i = √-1), then z lies on
(a) Re (z) = 2
(b) Im (z) = 2
(c) Re (z) + Im (z) = 2
(d) None of the above
Answer
A
Question: If |z1| =|z2 |, arg ( z1 / z2 ) = π, then z1+ z2 is equal to
(a) 0
(b) purely imaginary
(c) purely real
(d) None of these
Answer
A
Question: If ω is a complex cube root of unity, then the value of
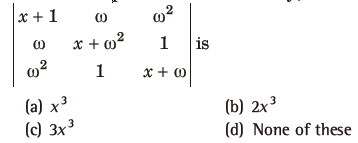
Answer
D
Question: If z1 and z2 be complex numbers such that z1≠ z2 and| z1 | |z2 |. If z1 has positive real part and z2 has negative imaginary part, then [( z1+ z2 )/( z1 -z2)] may be
(a) purely imaginary
(b) real and positive
(c) real and negative
(d) None of the above
Answer
A
Question: The trigonometric form of z = (1 – i cot 8)3(where i = √-1) is
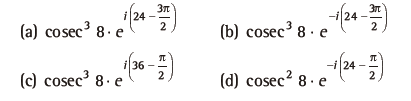
Answer
A
Question: If |z1|= |z2| =1 and amp z1+ ampz2= = 0, then
(a) z1z2 = 1
(b) z1 + z2 = 0
(c) z1 =¯z2
(d) None of these
Answer
(a,c)
Question: If |z1|< √2 – 1, then |z2+2z cos α| is
(a) less than 1
(b) 2 + 1
(c) 2 – 1
(d) None of these
Answer
A
Question:
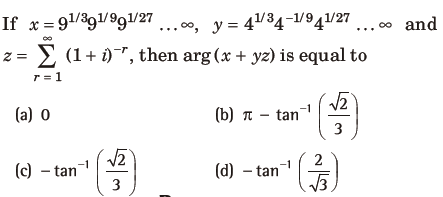
Answer
C
Question: If A(z1), B(z2) and C(z3) are the vertices of the ΔABC such that ( z1– z2 )/( z3 -z2 ) = (1/√2 – (i/1/ √2 , then Δ ABC is
(a) equilateral
(b) right angled
(c) isosceles
(d) obtuse angled
Answer
C
Question:

Answer
(a,b,c)
Question: The real value of q for which the expression
1+i cos θ/1-2i cos θ is a r eal num ber, is

Answer
(a,b,c)
Question:

(a) 3 √ 3
(b) √3
(c) 3
(d) 1/3√3
Answer
C
Question: If |z1|= 15 and |z2-3-4|5,then
(a) | z1 – z2 |min = 5
(b) | z1 – z2 |min = 10
(c) | z1 – z2 |max = 20
(d) | z1 – z2 |max = 25
Answer
(a,d)
Consider the complex numbers z1and z2 satisfying the relation
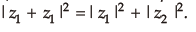
Question: Complex number z1¯z2
(a) purely real
(b) purely imaginary
(c) zero
(d) None of these
Answer
B
Question: Complex number z1¯z2 is
(a) purely real
(b) purely imaginary
(c) zero
(d) None of the above
Answer
B
Question: One of the possible argument of complex number
i(z1/z2)
(a) π/2
(b) – π/2
(c) 0
(d) None of these
Answer
C
Assertion and Reason
Each of these questions contains two
statements : Statement I (Assertion) and Statement II (Reason). Each of these questions also has four
alter native choices, only one of which is the correct answer. You have to select one of the codes (a), (b),(c) and (d) given be low.
(a) Statement I is true, Statement II is true; Statement II is a correct explanation for Statement I.
(b) Statement I is true, Statement II is true; Statement II is not a correct explanation for Statement I.
(c) Statement I is true; Statement II is false.
(d) Statement I is false; Statement II is true.
Question:
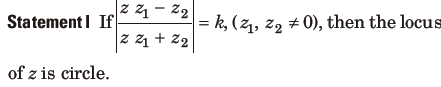
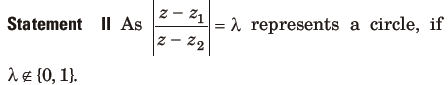
Answer
D
Question:
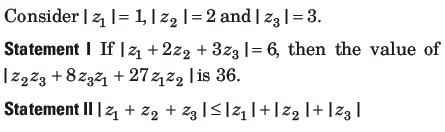
Answer
C
Question: Consider z1 and z2 are two complex numbers.
Statement I Such that |z1| |z2 |+ |z1– z2 |, then Im (z1/z2)=0
Statement II arg ( z) = 0⇒ z is purely real.
Answer
A
Question: Statement I If cos (1 – i) = a + ib, where a, b∈R and
i = √-1, then α =1/2(e+1/e) cos 1,b=1/2(e-1/e)sin 1.
Statement II e iθ = cos θ+i sin θ
Answer
A
Question:

Answer
D
Question: State ment I If arg (z1 z2) 2π then both z1and z2 are purely real (z1 and z2 have principal arguments).
State ment II Principal argument of complex number and between -π and π.
Answer
A
Question:Statement I The product of all values of (cos α+i sin α )3/5 is cos 3a +i sin 3a.
State ment II The product of fifth roots of unity is 1.
Answer
B
Question:

Answer
A
Question: Statement I
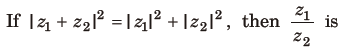
is purely imaginary.
State ment II If z is purely imaginary, then z + ¯z = 0.

Answer
C
Question: If z is a complex number of unit modulus and argument q, then arg(1+z/1+z) is equal to
(a) -θ
(b)π/2-θ
(c) q
(d) θ – q
Answer
C
Question: The number of complex numbers z such that
|z – 1|=|z + 1|=|z – i| is equal to
(a) 0
(b) 1
(c) 2
(d) ∞
Answer
B
Question: If z≠1 and z2/z-1 is real, then the point represented by the complex number z lies
(a) either on the real axis or on a circle passing through the origin
(b) on a circle with centre at the origin
(c) either on the real axis or on a circle not passing through the origin
(d) on the imaginary axis
Answer
A
Question:

(a) √3 + 1
(b) 5 + 1
(c) 2
(d)2+√2
Answer
B
Question: If |z + 4|≤ 3, then the max i mum value of |z + 1| is
(a) 4
(b) 10
(c) 6
(d) 0
Answer
C
Question:

(a) 54
(b) 6
(c) 12
(d) 18
Answer
C
Question: The conjugate of a complex number is 1/i – 1 . Then,that complex number is
(a)1/i – 1
(b) -1/-1
(c)1/i + 1
(d) -1/i+ 1
Answer
D
Question: If z1 and z2 are two non-zero com plex num bers such that |z1+ z2 | =| z1| +|z2| ,then arg ( z1 ) -arg ( z2 ) equal to
(a)- π/ 2
(b) 0
(c) -π
(d)π/2
Answer
B
Question: If ω=z/z-i/3 and |ω|= 1, then z lies on
(a) a parabola
(b) a straight line
(c) a circle
(d) an ellipse
Answer
B
Question: If(1+i/1+i)x=1, then
(a) x = 4n, where n is any positive integer
(b) x = 2n, where n is any positive integer
(c) x = 4n + 1, where n is any positive integer
(d) x = 2n + 1, where n is any positive integer
Answer
A
Question: If the cube roots of unity are 1,ω and ω2 , then the roots of the equation (x – 1)3 + 8 = 0, are
(a) -1,1 + 2ω,1 + 2ω2
(b) -1, 1 – 2ω,1- 2 ω2
(c) -1, -1, -1
(d) -1 -1 + 2ω-1-2ω2
Answer
B
Question:
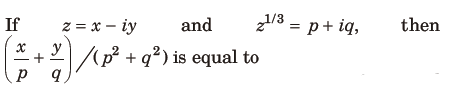
(a) 1
(b) -1
(c) 2
(d) -2
Answer
D
Question: If |z2-1|= |z|2+1,then z lies on
(a) the real axis
(b) the imaginary axis
(c) a circle
(d) an ellipse
Answer
B
Question: Let z1 and z2 be two roots of the equation z2+ az+ b= 0,z being com plex. Further, assume that the origin z1 and z2 form an equilateral triangle.
Then,
(a) a2 =b
(b) a2 = 2b
(c) a2 = 3b
(d) a2 = 4b
Answer
C
Question:
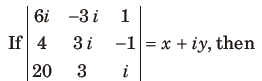
(a) x = 3, y = 1
(b) x = 1, y = 3
(c) x = 0, y = 3
(d) x = 0, y = 0
Answer
D
Question:

(a) 1
(b) -1
(c) i
(d) – i
Answer
D
Question: Let z and w be com plex num bers such that ¯z + i¯w = 0 and arg ( zω) = π. Then, arg (z) is equal to
(a)π /4
(b) π /2
(c) 3π /4
(d) 5π /2
Answer
C
46. If ω is an imaginary cube root of unity, then
(1 ) + ω – ω2)7 is equal to
(a) 128 w
(b) -128 w
(c) 128 w2
(d) -128 w2
Answer
D
Question: If w(≠ 1) is a cube root of unity and (1+ω )7 = A + Bω .
Then, (A, B) is equal to
(a) (1, 1)
(b) (1, 0)
(c) (-1, 1)
(d) (0, 1)
Answer
A
Question. If z=7-i/3-4i then z14 = ?
a. 27
b. 27 i
c. 214 i
d. – 27i
Answer
D
Question. Inverse of a point a with respect to the circle |z – c| = R (a and c are complex numbers, centre C and radius R) is the point c+R2/a̅ –c̅
a. c + R2/a̅ –c̅
b. c–R2/a̅ –c̅
c. c +R/c̅ -a̅
d. None of these
Answer
A
Question. If z1 = 2+5i , z2 = 3–i then projection of z2 on z2 is
a. 1/10
b. 1 / 10
c. −7 /10
d. None of these
Answer
B
Question. If complex numbers z1,z2 and z3 represent the vertices A, B and C respectively of an isosceles triangle ABC of which ∠C is right angle, then correct statement is
a. z12 + z22 + z32 = z1z2z3
b. (z3 – z1)2 = z3 – z2
c. (z1 – z2)2 = (z1 – z3)(z3 – z2)
d. (z1 – z2)2 = 2(z1 – z3)(z3 – z2)
Answer
D
Question. If zi = a + ib and z2 = c + ib are complex numbers such that |z1|=| z2|=1 and 1 2 Re(z1z2) = 0, then the pair of complex numbers w1 = a + ic and w2 = b + id satisfies?
a. |w1| = 1
b. |w2| = 1
c. 1 2 Re | w w |= 0
d. None of these
Answer
A,B,C
Question. Let z1 and z2 be complex numbers such that z1 ≠ z2 and |z1|=|z2|, If z1 has positive real part and z2 has negative imaginary part, then z1+z2/z1–z2 may be:
a. zero
b. real and positive
c. 0
d. 0
Answer
A,D
Question. The imaginary part of tan-1 (5i/3) is:
a. 0
b. ∞
c. log 2
d. log 4
Answer
C
Question. Let ω be a complex cube root of unity with ω ≠1 and P = [pij] be a n×n matrix with . Pij=ωi+j Then, P2 ≠ 0 when n is equal to:
a. 57
b. 55
c. 58
d. 56
Answer
C,D
Question. If z1,z2, z3, z4 are the four complex numbers represented by the vertices of a quadrilateral taken in order such that z1–z4=z2 –z3and amp (z4–z1/z2–z1) = π/2 , then the quadrilateral is:
a. rhombus
b. square
c. rectangle
d. cyclic quadrilateral
Answer
C,D
Question. If z1,z2,z3,z4 are roots of the equation a0z4 + a1z3 + a2z2 + a3z + a4 = 0
where a0, a1, a2, a3, and a4 are real, then:
a. z̅1,z̅2,z̅3,z̅4, are also roots of the equation
b. z̅1 is equal to at least one of z̅1,z̅2,z̅3,z̅4
c. –z̅1,–z̅2,–z̅3,–z̅4 are also roots of the equation d. none of the above.
d. none of the above
Answer
A,B
Assertion and Reason
Note: Read the Assertion (A) and Reason (R) carefully to mark the correct option out of the options given below:
a. If both assertion and reason are true and the reason is the correct explanation of the assertion.
b. If both assertion and reason are true but reason is not the correct explanation of the assertion.
c. If assertion is true but reason is false.
d. If the assertion and reason both are false.
e. If assertion is false but reason is true.
Question. Assertion: The maximum value of
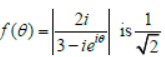
Reason: The minimum value of
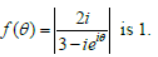
Answer
D
Question. Assertion: If z is a root of the equation z7 + 2x + 3 = 0, then 1 ≤ | z | < 3 / 2.
Reason: If z lies in the annular region 1< | z | ≤ 3/ 2, then z satisfies the 1/z-1 + 1/z-ω + 1/z-ω2 = 1 where ω ≠1 is a cube root of unity.
Answer
C
Question. Assertion: If ω≠1 is a cube root of unity, then A2 = O,

Reason: If ω≠1 is a cube root of unity, then
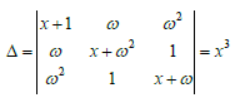
Answer
B
Question. Assertion: If z1, z2 z3 are such that |z1| + |z2|=|z3|=1, then maximum value of |z2 − z3|2 + | z3 − z1 | + | z1 − z2 | is 9.
Reason: If z1, z2 z3 are such that |z1|=|z2|=|z3| =1 hen Re(z2z3 +z1z2 ) ≥ 3/2.
Answer
A
Question. Assertion: If z2 − z +1 = 0 and n is a natural number, then
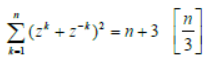
denotes the greatest integer ≤ x.
Reason: If ω ≠ 1 is a cube root of unity, then

Answer
A
Question. If z1 and z2 are complex numbers, such that
|15z1 −13z2|2 + |13z1 +15z2 |2 = λ |z1|2 +|z2|2), then the value of √λ√λ√λ√λ…..∞ must be equal to:
Answer
394
