Worksheets For Class 10 Mathematics Arithmetic Progression

Arithmetic Progression Class 10 Worksheet have been designed as per the latest pattern for CBSE, NCERT and KVS for Grade 10. Students are always suggested to solve printable worksheets for Mathematics Arithmetic Progression Grade 10 as they can be really helpful to clear their concepts and improve problem solving skills. We at worksheetsbag.com have provided here free PDF worksheets for students in standard 10 so that you can easily take print of these test sheets and use them daily for practice. All worksheets are easy to download and have been designed by teachers of Class 10 for benefit of students and is available for free download.

Mathematics Arithmetic Progression Worksheets for Class 10

We have provided chapter-wise worksheets for class 10 Mathematics Arithmetic Progression which the students can download in Pdf format for free. This is the best collection of Mathematics Arithmetic Progression standard 10th worksheets with important questions and answers for each grade 10th Mathematics Arithmetic Progression chapter so that the students are able to properly practice and gain more marks in Class 10 Mathematics Arithmetic Progression class tests and exams.

Chapter-wise Class 10 Mathematics Arithmetic Progression Worksheets Pdf Download

Question. If the sum of first n even natural numbers is equal to k times the sum of first n odd natural numbers, then k =   
(A) 1/n
(B) n-1/n
(C) n+1 /2n
(D) n+1 /n

Question. If n AMs are inserted between 2 & 38, the sum of the resulting series obtained is 200. The value of n (total number of terms) is   
(A) 8
(B) 10
(C) 9
(D) 11

Question. Find t5 and t6 of the arithmetic progression 0, 1/4, 1/2, 3/4,……. respectively   
(A) 1, 5/4 
(B) 5/4, 1
(C) 1, 7/4
(D) 7/4, 1

Question. If tn = 6n + 5, then tn + 1 =       
(A) 6n –1
(B) 6n+11
(C) 6n + 6
(D) 6n – 5

Question. Which term of the arithmetic progression 21, 42, 63, 84, ……. is 420?       
(B) 20
(C) 21
(D) 22

Question. Find the 15 term of the arithmetic progression 10, 4, –2,……   
(A) –72
(B) –74
(C) –76
(D) –78

Question. Find the smallest positive term of the series 25,22(3/4) ,20(1/2) ,18(1/4) …………..?   
(A) 9th
(B) 10th
(C) 11th
(D) 12th

Question. The sum of first four terms of an A.P. is 56. The sum of last four terms is 112. If its first term is 11, find number of terms?   
(A) 8
(B) 9
(C) 10
(D) 11

Question. If the kth term of the arithmetic progression 25, 50, 75, 100,…….. is 1000, then k is ________.   
(A) 20
(B) 30
(C) 40
(D) 50

Question. The sum of the first 20 terms of an arithmetic progression whose first term is 5 and common difference is 4, is   
(A) 820
(B) 830
(C) 850
(D) 860

Question. Find the 15th term of the series 243, 81, 27,…….. 
(A) 1/314
(B) 1/38
(C) (1/3)9
(D) (1/3)10

Question. In a right triangle, the lengths of the sides are in arithmetic progression. If the lengths of the sides of the triangle are integers, which of the following could be the length of the shortest side?   
(A) 1225
(B) 1700
(C) 1275
(D) 1150

Question. If S₁ = 3,7,11,15,…….. upto 125 terms and S₂ 4,7,10,13,16…….. upto 125 terms, then how many terms are there in S1 that are there in S2?    
(A) 29
(B) 30
(C) 31
(D) 32

Question. The sum of n terms of two A.P’s are in ratio 5n+ 4:9n+6 find ratio of their 18th terms?   
(A) 179:321
(B) 180:322
(C) 170:320
(D) 171:329

Question. If log2 (5X2^x+1) , log4 (2^1-x +1 ) and 1 are in A.P. find x?   
(A) log₂⁵
(B) 1-log₂⁵
(C) log₅² 
(D) 1-log₅² 

Question. Find the sum of all natural numbers and lying between 100 and 200 which leave a remainder of 2 when divided by 5 in each case.     
(A) 2990
(B) 2847
(C) 2936
(D) none of these

Question. If the first, second and last term of an A.P. are a, b and 2a respectively, its sum is       
(A) ab/2(b-a)
(B) ab/(b-a)
(C) 3ab /2(b-a)
(D) none of these

Question. If S₁ is the sum of an arithmetic progression of ‘n’ odd number of terms and S₂ the sum of the terms of the series in odd places, then S₁ /S₂ =     
(A) 2n/n+1
(B) n/n+1
(C) n+1/2n
(D) n+1/n

Question. The sum of the first 51 terms of the arithmetic progression whose 2nd term is 2 and 4th tem is 8, is   
(A) 3774
(B) 3477
(C) 7548
(D) 7458

Question. Three alternate terms of an arithmetic progression are x + y,x – y and 2x + 3y, then x =
(A) -y
(B) -2y
(C) -4y
(D) -6y

VERY SHORT ANSWER TYPE QUESTIONS

Question. Write the missing terms of the A.P. –9, , –19, –24,

Question. Which term of the A.P. 19, 18 , 1/5 , 17, 2/5 ,….. is the first negative term? 

Question. For what value of k, the numbers k + 2, 4k + 4 and 9k + 4 are three consecutive terms of an A.P.

Question. Write the first three terms of an A.P. whose nth term is –3n + 5.

Question. Find the number of terms of A.P. 7, 13, 19, ….., 301.

Question. Write the A.P. whose nth term is 4n – 7.

Question. The 17^th term of an A.P. exceeds its 10th term by 7. Find the common difference.

Question. How many 2-digit numbers are divisible by 3?

Question. Find a, such that –15, a, 35 are in A.P.
Solution. 

Question. Find the sum of first 50 natural numbers.

Question. Find the following sum : 1 + 3 + 5 + ….. 20 terms.

Question. If the sum of first n terms of an A.P. is 2n2 + 5n, write the sum of its first 5 terms.

Question. Which term of the A.P. 2, 6, 10, …. is 210?

Question. Is 67 a term of the A.P. 7, 10, 13, ……?

Question. Find the sum to n terms of the A.P. whose rth term is 5r + 1.

Question. Find the sum of first 15 terms of an AP, whose nth term is 9–5n.

Question. Given that the first term of an A.P. is 2 and its common difference is 4, find the sum of its first 40 terms.

Question. Find the sum of the odd numbers between 0 and 50.

Question. Find the 10th term of an A.P. –3, -1/2 , 2,…. 

Question. Write the 15^th term of an A.P. whose first term is 7 and the common difference is –3.

CBSE Class 10 Mathematics Arithmetic Progression Worksheet Set A

CBSE Class 10 Mathematics Arithmetic Progression Worksheet Set B

CBSE Class 10 Mathematics Arithmetic Progression Worksheet Set C

CBSE Class 10 Mathematics Arithmetic Progression Worksheet Set D

CBSE Class 10 Mathematics Arithmetic Progression Worksheet Set E

CBSE Class 10 Mathematics Arithmetic Progression Worksheet Set F

CBSE Class 10 Mathematics Arithmetic Progression Worksheet Set G

CBSE Class 10 Mathematics Arithmetic Progression Worksheet Set H

CBSE Class 10 Mathematics Arithmetic Progression Worksheet Set I

CBSE Class 10 Mathematics Arithmetic Progression Worksheet Set J

CBSE Class 10 Mathematics Arithmetic Progression Worksheet Set K

CBSE Class 10 Mathematics Arithmetic Progression Worksheet Set L

CBSE Class 10 Mathematics Arithmetic Progression Worksheet Set M

CBSE Class 10 Mathematics Arithmetic Progression Worksheet Set N

CBSE Class 10 Mathematics Arithmetic Progression Worksheet Set O

CBSE Class 10 Mathematics Arithmetic Progression Worksheet Set P

CBSE Class 10 Mathematics Arithmetic Progression Worksheet Set Q

CBSE Class 10 Mathematics Arithmetic Progression Worksheet Set R

CBSE Class 10 Mathematics Arithmetic Progression Worksheets Set A

CBSE Class 10 Mathematics Arithmetic Progression Worksheets Set B

CBSE Class 10 Mathematics Arithmetic Progression Worksheets Set C

CBSE Class 10 Mathematics Arithmetic Progression Worksheets Set D

PRACTICE EXERCISE

Question. For what value of n, the n^th terms of the two A.P.’s are same? 3, 10, 17, …… and 63, 65, 67, …..
Solution. n = 13

Question. Find the three numbers in A.P. such that their sum is 24 and the sum of their squares is 194.
Solution. 7, 8 and 9

Question. If the 10^th term of an A.P. is 52 and 17^th term is 20 more than the 13^th term, find the A.P.
Solution. 7, 12, 17, 22…….

Question. If the sum of the first 6 terms of an A.P. is 36 and the sum of the first 21 terms is 441, find the first term and the common difference and hence find the sum of n terms of this A.P.
Solution. a = 1, d = 2; Sn = n²

Question. Find the number of integers between 200 and 500 which are divisible by 7.
Solution. 43

Question. How many terms of the sequence –35, –28, –21, …… should be taken so that their sum is zero?
Solution. 11

Question. How many terms of the A.P. 63, 60, 57,….. must be taken so that their sum is 693? 
Solution. 21 or 22

Question. How many numbers of three digits are exactly divisible by 11?
Solution. 81

Question. The angles of a triangle are in A.P. If the greatest angle equals the sum of the other two, find the angles.
Solution. 30°, 60°, 90°

Question. Three numbers are in A.P. If the sum of these numbers is 27 and the product is 648, find the numbers.
Solution. 6, 9, 12

Question. A sum of Rs. 1000 is invested at 8% simple interest per annum. Calculate the interest at the end of 1, 2, 3, ……years. Is the sequence of interest an A.P.? Find the interest at the end of 30 years.
Solution. Rs. 80, Rs. 160, Rs. 240, and so on. Yes; Rs. 2400

Question. Two A.P.’s have the same common difference. The difference between their 100th terms is 111222333. What is the difference between their millionth terms?
Solution. 111222333

Question. Find the sum of first n natural numbers.
Solution. n(n + 1)/2

Question. Two cars start together in the same direction from the same place. The first goes with a uniform speed of 10 km/hr. The second goes with a speed of 8 km/hr in the first hour and increases the speed by 1/2 km/hr in each succeeding hour. After how many hours will the second car overtake the first if both cars go nonstop?
Solution. 9 hours

Question. The ages of the students in a class are in A.P. whose common difference is 4 months. If the youngest student is 8 years old and the sum of the ages of all the students is 168 years, find the number of students in the class.
Solution. 16

Question. Find the sum of first 30 even natural numbers.
Solution. 930

Question. Find the sum of first 25 odd natural numbers.
Solution. 625

Question. Find the value of k so that k – 3, 4k – 11 and 3k – 7 are three consecutive terms of an A.P.
Solution. k = 3

Question. Find the value of x so that 3x + 2, 7x – 1 and 6x + 6 are three consecutive terms of an A.P.
Solution. x = 2

Question. The 4^th term of an A.P. is three times the first and the 7^th term exceeds twice the third term by 1. Find the first term and the common difference.
Solution. a = 3, d = 2

Question. The 9^th term of an A.P. is equal to 7 times the 2^nd term and 12^th term exceeds 5 times the 3rd term by 2. Find the first term and the common difference.
Solution. a = 1, d = 6

Question. Find the sum of n terms of an A.P. whose nth term is given by an = 5 – 6n.
Solution. 2n –3n²

Question45. In an A.P., an = 116, a = 2 and d = 6. Find Sn.
Solution. 1180

Question. Find the A.P. whose third term is 16 and the seventh term exceeds its fif^th term by 12.
Solution. 4, 10, 16, ……

Question. The n^th term of an A.P. is given by tn = 4n – 5. Find the sum of the first 25 terms of the A.P.
Solution. 1175

Question. between 250 and 1000 which are exactly divisible by 3.
Solution. 156375

Question. between 50 and 500 which are divisible by 7.
Solution. 17696

Question. between 100 and 1000 which are multiples of 5.
Solution. 98450

Question. How many terms of an A.P. 1, 4, 7, ….. are needed to give the sum 1335?
Solution. 30

Question. The sum of the third and the seventh terms of an A.P. is 6 and their product is 8. Find the sum of first ten terms of the A.P.
Solution. 32.5 or 27.5

Question. How many terms of an A.P -6 , 11/2 , -5 ….. are needed to give the sum –25? Explain the double answer.
Solution. 5 or 20

Question. For what value of n is the n^th term of the following A.P.’s the same? 1, 7, 13, 19, ….. and 69, 68, 67…………… 
Solution. No value of n

Question. Find the middle term of an A.P. with 17 terms whose 5^th term is 23 and the common difference is –2.
Solution. 15

Question. For what value of n, the n^th terms of the two A.P.’s are same? 2, –3, –8, –13, …… and –26, –27, –28, –29, …….
Solution. n = 8

Question. Which term of the A.P. 3, 10, 17, …. will be 84 more than its 13^th term? 
Solution. 25^th

Question. Find the 20^th term from the end of the A.P. 3, 8, 13, ….., 253.
Solution. 158

Question. Find the sum of first 20 terms of an A.P., in which 3rd term is 7 and 7^th term is two more than thrice of its 3^rd term.
Solution. 740

Question. Find the 8^th term from the end of the A.P. 7, 10, 13, ….. , 184. 
Solution. 163

Question. between 50 and 500 which are divisible by 3 and 5.
Solution. 8325

Question. The sums of n-terms of two A.P.’s are in the ratio 7n + 1 : 4n + 27. Find the ratio of their 11^th terms.
Solution. 4 : 3

Question. The 10^th term of an A.P. is 29 and and sum of the first 20 terms is 610. Find the sum of the first 30 terms.
Solution. 1365

Question. (a) For an A.P., find Sn if given a_n = 3 – 5n.
(b) For an A.P., find an if given S_n = 2n² + 5n.
Solution. (a) n/2 (1 –5n) (b) 4n + 3

Question. The sum of the first 15 terms of an A.P. is 105 and the sum of next 15 terms is 780. Find the first three terms of the A.P.
Solution. –14, –11, –8

Question. Find the A.P. whose n^th term is given by : (a) 9 – 5n (b) –n + 6 (c) 2n + 7
Solution. (a) 4, – 1, –6, –11, ….. (b) 5, 4, 3, 2, ……. (c) 9, 11, 13, 15, ……

Question. Find the 8^th term of an A.P. whose 15th term is 47 and the common difference is 4.
Solution. 19

Question. The 10^th term of an A.P. is 52 and 16^th term is 82. Find the 32nd term and the general term.
Solution. a₃₂ = 162, a_n = 5n + 2

Question. In an A.P., the sum of the first n terms is 3n²/2 + 13/n , n. Find its 25^th term.
Solution. 80

Question. The sum of the first 9 terms of an A.P. is 171 and that of the first 24 terms is 996, find the first term and the common difference.
Solution. a = 7, d = 3

Question. A man saved Rs. 32 during the first year, Rs. 36 in the second year and in this way be increases his savings by Rs. 4 every year. Find in what time his savings will be Rs. 200.
Solution. 5 years.

Question. If the sum of the first 20 terms of an A.P. is 400 and the sum of the first 40 terms is 1600, find the sum of its first 10 terms.
Solution. 100

Question. Find the common difference of an A.P. whose first term is 1 and the sum of the first four terms is one-third the sum of the next four terms.
Solution. 2

Question. A person borrows Rs. 4500 and promises to pay back (without any interest) in 30 instalments each of value Rs. 10 more than last (preceding one). Find the first and the last instalments.
Solution. Rs. 5, Rs. 295

Question. The 7^th term of an A.P. is – 4 and its 13^th term is –16. Find the A.P.
Solution. 8, 6, 4, …..

Question. The sum of the 4th and 8th terms of an A.P. is 24 and the sum of the 6th and 10th terms is 44. Find the sum of first 20 terms of the A.P.
Solution. 690

Question. Find the sum of first 25 terms of an A.P. whose nth term is given by an = 2 – 3n.
Solution. –925

Question. The sum of the third and the seventh terms of an A.P. is 6 and their product is 8. Find the sum of first sixteen terms of the A.P.
Solution. 20 or 76

Question. Find the number of integers between 50 and 500 which are divisible by 7.
Solution. 64

Question. (a) Find the 12^th term from the end of the A.P. 3, 8, 13, ….. , 253.
(b) How many three digit numbers are divisible by 7?
Solution. (a) 198 (b) 128

Question. Find the sum of first 24 term of AP 5,8,11,14………..
Solution. 948

Question. Find the middle term of A.P 6,13,20,………..216
Solution. 111

Question. Find whether 0 is a term of the A.P: 40,37,34,31………….
Solution. no

Question. Write the value of x for which x+2,2x,2x+3 are three consecutive terms of an A.P
Solution. 5

Question. The 6t hterm of an A.P is -10 and its 10thterm is -26.Determine the15thterm of an A.P
Solution. -46

Question. Write the next term of an A.P. √2 , √18
Solution. 5√2

Question. Find the sum of three digits numbers which are divisible by 11
Solution. 6,12

Question. The 8thterm of an A.P is 0 prove that its38t h term is triple its18th term.
Solution. -800

Question. Which term of the A.P 12,7,2,-3……is -98
Solution. 23rdterm

Question. Write fourth term of an AP if its nth term is 3n+2.
Solution. 14

Question. If 4/5 ,a,2 three consecutive term of an A.P then find ’a’.
Solution. 7/5

Question. The sum of three numbers in A.P is 21and their product is 231 find the numbers.
Solution. 2475

Question. Determine the 10thterm from the end of the A.P:4,9,14……..254
Solution. 209

Question. Find the sum of 25^th term of an AP which nth term is given by tn=(7-3n)
Solution. 44550

Question. Find the sum of all two digit odd positive numbers
Solution. 2, 26

Question. Find A.P which fifth terms are 5 and common difference is –3.
Solution. 17

Question. The nth term of an A.P is 3n+5 find its common difference.
Solution. 3

Question. Salman Khan buys a shop for Rs. 1, 20,000. He pays half of the amount in cash and agrees to pay the balance in 12 annual instalments of Rs. 5000 each. If the rate of interest is 12% and he pays with the instalment the interest due on the unpaid amount, find the total cost of the shop.
Solution. Rs. 1,66,800

Question. Find the term of A.P. 9, 12, 15, 18, …. which is 39 more than its 36th term.
Solution. 49^th

Question. If the sum of 7 terms of an A.P. is 49 and that of 17 terms is 289, find the sum of n terms.
Solution. n²

Question. Pranshi saved Rs. 5 in the first week of the year and then increased her weekly savings by Rs. 1.75 each week. In what week will her weekly savings be Rs. 20.75?
Solution. 10^th week

Question. Find the sum of first 15 multiples of 8.
Solution. 0

Question. If Sn denotes the sum of first n terms of an AP, prove that S₁₂ = 3(S₈ – S₄)
Solution. We know that sum of n terms of an AP,
S_n =n/2[2a + ( n – 1) d]
S₄ =4/2 [2a + (4 – 1)d]
= 2 [2a +3d] = 4a + 6d
S₈ =8/2[2a + (8 – 1)d]
= 4 [2a +7d] = 8a + 28d
(S8 – S4) = (8a + 28d) – (4a +6d)
= 8a + 28d – 4a – 6d
= 4a + 22d
S₁₂ =12/2 [2a + (12 – 1)d]
= 6 [2a +11d] = 12a + 66d
= 3 [4a + 22d]
S₁₂ = 3 [S8 – S4] [using equation (i)]
Hence proved.

Question. If the sum of the first 6 terms of an AP is 36 and that of the first 16 terms is 256, find the sum of the first 10 terms.
Solution. It is given that
S₆ = 36 and S₁₆ = 256
Let a be the first term and d be the common difference of an AP.
We know that sum of n terms of an AP,
S_n =n/2[2a + (n – 1)d]
Now S6 = 36 (Given)
⇒ 6/2[2a + (6 – 1)d] = 36
⇒ 3 [2a + (6 – 1)d = 36
⇒ 2a + 5d = 12
Also, S₁₆ = 256
⇒16/2[2a + (16 – 1)d] = 256
⇒ 8[2a + 15d] = 256
⇒ 2a + 15d = 32 …(ii)
Subtracting equation (i) from equation (ii), we get
(2a + 15d) – (2a + 5d) = 32 – 12
⇒ 2a + 15 – 2a – 5d = 20
⇒ 10d = 20
⇒ d = 2
Putting the value of d in equation (i), we get
2a + 5d = 12
2a + 5(2) = 12
⇒ 2a + 10 = 12
⇒ 2a = 2 ⇒ a = 1
We have to find sum of first 10 terms, S₁₀ = ?
S₁₀ =10/2[2a + (10 – 1)d]
= 5 [2(1) + 9(2)]
= 5 [2 + 18]
= 5 × 20
S₁₀ = 100
Hence, the required sum of the first 10 terms is 100.

Question. The sum of the first n terms of an AP whose first term is 8 and the common difference is 20 is equal to the sum of first 2n terms of another AP whose first term is -30 and the common difference is 8. Find n.
Solution. It is given that
first term of first AP, a = 8
and common difference of first AP, d = 20
Let n be the number of terms in first AP.
We know that sum of first n terms of an AP,
S_n =n/2[2a + (n – 1)d]
=n/2[2 × 8 + (n – 1)20]
=n/2[16 + 20n – 20]
=n/2[20n – 4] = n[10n – 2]
⇒ S_n = n[10n – 2] …(i)
Now, first term of second AP (a’) = –30
Common difference of secod (d’) = 8
∴ Sum of first 2n terms of second AP
S_2n =2n/2 [2a’ + (2n – 1)d’]
= n[2(–30) + (2n –1)8]
= n[–60 + 16n – 8]
S_2n = n[16n – 68] …(ii)
By given condition,
Sum of first n terms of first AP
= sum of first 2_n terms of second AP
⇒ Sn = S_2n
Using equation (i) and equation (ii), we get
⇒ n(10n – 2) = n(16n – 68)
⇒ 10n – 2 – 16n + 68 = 0
⇒ –6n + 66 = 0
⇒ –6(n – 11) = 0
⇒ n = 11
Hence, the required value of n is 11.

Question. If mth term of an A.P. is 1/ n and nth term is 1/m , find the sum of its first mn terms.
Solution. Let, the first term of an A. P. be ‘a’ and its common difference be ‘d’.
Now, am = a + (m – 1)d
⇒ 1/n = a + (m – 1)d …(i) (given)
and a_n = a + (n – 1)d
⇒ 1/m = a + (n – 1)d …(ii) (given)
On subtracting eduation (ii) from (i), we get.

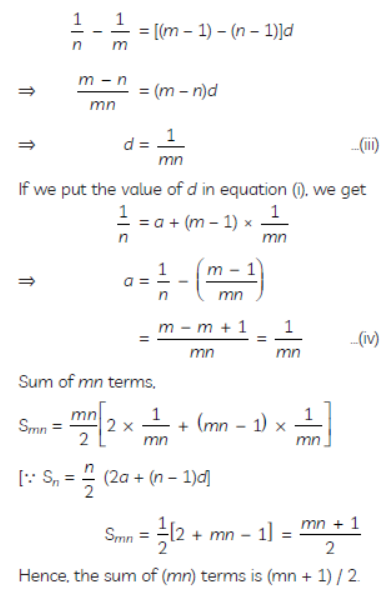
Question. Find the sum of n terms of the series

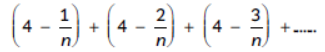
Solution. Given series is

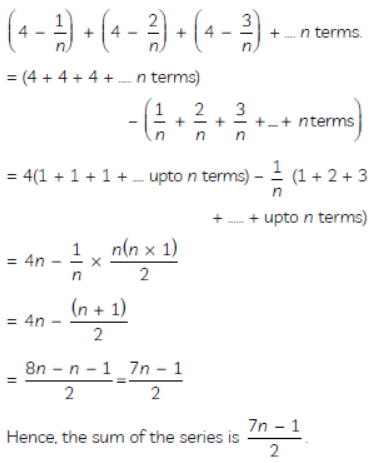
Question. For what value of n are the nth terms of two A.P.’s 63, 65, 67, …. and 3, 10, 17, ….. equal ?
Ans. Given, APs are 63, 65, 67……. and 3, 10, 17……..
For first A.P., first term, a = 63 and common
difference, d = 2
Then, its nth term = 63 + (n – 1) × 2 …(i)
For second A.P., first term, a’ = 3 and common
difference, d’ = 7
Then, its nth term = 3 + (n − 1) × 7
According to the question:
63 + (n − 1) × 2 = 3 + (n − 1) × 7
(n − 10 × 5 = 60
n − 1 = 12
n = 13
The 13^th term of both given APs are equal.

Question. The first term of an AP is -5 and the last term is 45. If the sum of the terms of the AP is 120, then find the number of terms and the common difference. 
Solution. Let a be the first term, d be the common difference and n be the number of terms.
It is given that
first term, a = –5
Last term, L = 45
We know that, if the last term of an AP is
known, then the sum of n terms of an AP is
Sn =n /2 (a +l )
120 =n/2(-5 + 45)
120 × 2 = 40 × n
⇒ n = 6
L = a + (n – 1)d
⇒ 45 = –5 + (6 – 1)d
⇒ 50 = 5d ⇒ d = 10
Hence, number of terms = 6
and common difference = 10

Question. Find the sum of the first 40 positive integers which give a remainder 1 when divided by 6.
Solution. The numbers which when divided by 6 gives 1 as remainder are :
7, 13, 19, 25, 31, 37, …
They form an A.P., as their common difference
is the same, d = 6
Here: first term, a = 7, common difference, d = 6
Sum of first 40 positive integers:
S₄₀ =n/2[2a + (n – 1)d]
=40/2[2 × 7 + (40 – 1) × 6]
= 20[14 + 39 × 6]
= 20 × 248
= 4,960
Hence, the sum of the first 40 positive integers is 4,960.

Question. Divide 56 in four parts in A.P such that the ratio of the product of their extremes (1st and 4th) to the product of means (2nd and 3rd) is 5 : 6.
Solution. Let the four parts of the A.P. are (a – 3d), (a – d), (a + d), (a + 3d)
Now, a – 3d + a – d + a + d + a + 3d = 56
⇒ 4a = 56
⇒ a = 14
According to question,
(a−3d)(a+3d)/(a-d)(a+d) = 5/6
⇒ (14 – 3d)(14 + 3d) / (14 – d) (14 + d) = 5/6 [Putting a = 14]
⇒ 196 – 9d2 / 196 – d² = 5/6
⇒ 1176 – 54d² = 980 – 5d²
⇒ 49d² = 196
⇒ d² = 4 ⇒ d = ±2
when,
a = 14 and d = 2
The 4 parts are (a – 3d), (a – d), (a + d), (a + 3d), i.e., 8, 12, 16, 20.
when, a = 14 and d = –2
The 4 parts are 20, 16, 12 and 8.

Question. If the sum of first m terms of an AP is the same as the sum of its first n terms, show that the sum of its first (m + n) terms is zero.
Solution. Sm = Sn
⇒ = m/2[2a + (n − 1)d]
= n/2[2a + (n − 1)d]
⇒ 2a(m − n) + d(m² − m − n² + n) = 0
⇒ (m − n)[2a + (m + n − 1)d] = 0
or Sm + n = 0

Question. How many terms of an A.P. 9, 17, 25,… must be taken to give a sum of 636?
Solution.

Question. Among the natural numbers 1 to 49, find a number x, such that the sum of numbers preceding it is equal to sum of numbers succeeding it.
Solution. Let, the number be x.
1, 2, 3, 4, …, x – 1, x, x + 1, … 49
1 + 2 + 3 + 4 + … + x – 1 = x + 1 + … + 49
S_{x – 1} = S₅₀ – S_x
⇒ ( x -1 )x /2 = 49 x 50/2 − (x+ 1)x/2
⇒ x² – x = 2450 – x² – x
⇒ x² = 1225
⇒ x = ±35 (–35 is not between 1 to 49 therefore rejected)
x = 35.

Question. The 14^th term of an AP is twice its 8th term. If its 6th term is -8, then find the sum of its first 20 terms.
Solution. a₁₄ = 2a₈
⇒ a + 13d = 2(a + 7d) ⇒ a = –d
a₆ = –8
⇒ a + 5d = –8
solving to get a = 2, d = –2
S₂₀ = 10(2a + 19d)
= 10(4 – 38)
= –340

QQuestionuestion. The digits of a positive number of three digits are in A.P. and their sum is 15. The number obtained by reversing the digits is 594 less than the original number. Find the number.
Solution. Let the required numbers in A.P. are (a – d), a, (a + d) respectively.
Now, a – d + a + a + d = 15
3a = 15 ⇒ a = 5
According to question, number is
100(a – d) + 10a + a + d = 111a – 99d
Number on reversing the digits is
100(a + d) + 10a + a – d = 111a + 99d
Now, as per given condition in question,
(111a – 99d) – (111a + 99d) = 594
⇒ –198d = 594 ⇒ d = –3
So, digits of number are [5 – (–3), 5, (5 + (–3)]
= 8, 5, 2
Required number is 111 × (5) – 99 × (– 3)
= 555 + 297 = 852
The number is 852.

Question. The digits of a positive number of three digits are in A.P. and their sum is 15. The number obtained by reversing the digits is 594 less than the original number. Find the number.
Solution. 

Question. Find the sum of first 24 terms of an A.P. whose n^th term is given by a_n = 3 + 2_n.
Solution. Given, nth term of an A.P., an = 3 + 2_n
First term of A.P., a₁ = 3 + 2 × 1 = 5
Second term of A.P., a₂ = 3 + 2 × 2 = 7
third term of A.P., a₃ = 3 + 2 × 3 = 9
and 24th term of A.P., a₂₄ = 3 + 2 × 24 = 51
common difference of A.P., d = a₂ – a₁ = a₃ – a₂
= 7 – 5 = 9 – 7 = 2
Sum of 24 terms, S24 = n /2 [2a+(n – 1)d]
Here, a = 5, n = 24, d = 2
S₂₄ =24/2[2 x 5 + (24 – 1) x2]
= 12 [10 + 46]
= 12 × 56 = 672
Hence the sum of first 24 terms of an A.P. is 676.

Question. Determine the A.P. whose third term is 16 and the 7^th term exceeds the 5^th term by 12. 
Solution. Let, the first term of A.P. be ‘a’ and its common difference be ‘d’
Given, a₃ = 16
i.e. a + (3 – 1)d = 16 [∵ an = a + (n – 1)d]
⇒ a + 2d = 19 …(i)
And a7 = a₅ + 12
⇒ a + (7 – 1)d = a + (5 – 1)d + 12
⇒ a + 6d = a + 4d + 12
⇒ 2d = 12
⇒ d = 2
If we put the value of ‘d’ in eduation (i), we get;
a + 2 × 2 = 16
⇒ a = 16 – 4 = 12
First term of AP = 12
Second term of AP = 12 + 2 = 14
Third term of AP = 14 + 2 = 16 and so on.
Hence, the required A.P is 12, 14, 16, 18, ….

Question. The 26^th, 11^th and the last term of an AP are 0, 3 and -1/5 respectively. Find the common difference and the number of terms.
Solution. It is given that:
26^th term of AP, a26 = 0
11^th term of AP, a11 = 3
Last term, L =-1/5
Let the AP contain n term, last term (L) is the n^th term.
Let first term be a and common difference be d of an AP.
We know that
an = a + (n – 1)d
Also, a₂₆ = 0 [Given]
⇒ a₂₆ = a + (26 – 1)d
⇒ 0 = a + 25d
⇒ a + 25d = 0
Also, a11 = 3
⇒ a + (11 – 1) d = 3
⇒ a + 10d = 3
Last term L = -1/5
a + (n – 1) d = -1/5
Subtracting equation (ii) from equation (i), we get
⇒ (a + 25d) – (a + 10d) = 0 – 3
⇒ a + 25d – a – 10d = 0 – 3
⇒ 15d = –3
⇒ d = -3/15 = -1/15
Putting the value of d in equation (i)
⇒ a + 25(-1/5) = 0
⇒ a – 5 = 0
⇒ a = 5
Putting the value of a and d in equation (iii)
⇒ a + (n – 1) d = -1/5
⇒ 5 + (n – 1) (-1/5) =-1/5
⇒ 25 – (n – 1) = –1
⇒ 25 + 1 = (n – 1)
⇒ n = 27
Hence, the common difference =-1/5 and number of terms = 27.

Question. If the sum of the first 7 terms of an A.P. is 49 and that of its first 17 terms is 288, find the sum of first n terms of the A.P. 
Solution. Let the first term of an A.P be ‘a’ and its common difference be ‘d’.
given: S₇ = 49
and S₁₇ = 289
Then, S₇ = 7/2[2a + (7 – 1) × d]
[∵ S_n = n/2[2a + (n -1 )d]
⇒ 49 = 72[2a + 6d]
⇒ 7 = a + 3d …(i)
and S₁₇ = 17/2[2a + (17 – 1) × d]
⇒ 289 = 172× [2a + 16 × d]
⇒ 17 = a + 8d …(ii)
On subtracting equation (i) from equation (ii), we get:
a + 8d = 17 89
If we put the value of ‘d’ in equation (i), we get
a + 3 × (2) = 7
⇒ a = 7 – 6 = 1
Sum of the first ‘n’ terms,
S_n =n²[2a + (n – 1)d]
=n²[2 + (n – 1) × 2]
=n²[2 + 2n – 2]
= n²
Hence, the sum of the first ‘n’ terms is n².

Question. The sum of four consecutive number in AP is 32 and the ratio of the product of the first and last terms to the product of two middle term is 7 : 15. Find the numbers.
Solution. Let ‘a’ be the first term and ‘d’ be the common
difference to the AP. then,
a – 3d, a – d, a + d, a + 3d,
are four consecutive terms of the AP.
As per the question,
(a – 3d) + (a – d) + (a + d) + (a + 3d) = 32
⇒ 4a = 32, or a = 8 …(i)
and (a – 3d)(a+3d)/(a – d)(a + d) = 7/15
⇒ a2 – 9d² / a² – d² = 7/15
⇒ 15a² – 135d² = 7a² – 7d²
⇒ 8a2 = 128 d²
⇒ sing (i), we have:
8 × 82 = 128 d²
⇒ d² = 4, or d = ± 2
Thus, the four numbers are 2, 6, 10 and 14

Question. Solve : 1 + 4 + 7 + 10 + …. + x = 287.
Solution. In the given AP, a = 1 and d = 3.
Let AP contains ‘n’ terms. Then, an = x
⇒ a + (n – 1)d = x
⇒ 1 + 3(n – 1) = x
⇒ n = x + 2/3
Further, Sn =n/2[first term + last term]
⇒ 287 = x + 2/3 x 2 [1 + x]
⇒ (x + 1) (x + 2) = 1722
or x² + 3x – 1720 = 0
⇒ x² + 43x – 40x – 1720 = 0
⇒ x(x + 43) – 40(x + 43) = 0
⇒ (x + 43) (x – 40) = 0
⇒ x – 40 = 0 (∵ x + 43 ≠ 0)
⇒ x = 40.
Thus, x = 40.

Question. The sum of the first 5 terms of an AP and the sum of the first 7 terms of the same AP is 167. If the sum of the first 10 terms of this AP is 235, find the sum of its first 20 terms.
Solution. Let a be the first term, d be the common difference and n be the number of terms of an AP.
We know that
S_n =n/2[2a + (n – 1)d]
Sum of first five terms, S5
S₅ =5/2[2a + (5 – 1)d] =5/2[2a + 4d]
S₅ = 5[a + 2d]
S₅ = 5a + 10d …(i)
Sum of first seven terms, S7
S₇ =7/2[2a + (7 – 1)d]
= 7/2[2a + 6d]
S₇ = 7[a + 3d]
S₇ = 7a + 21d …(ii)
Now, by given condition
S₅ + S7 = 167
⇒ 5a + 10d + 7a + 21d = 167
[using equation (i) & equation (ii)]
⇒ 12a + 31d = 167 …(iii)
Also, it is given that sum of first 10 terms of this AP is 235.
⇒ S₁₀ = 235
⇒10/2[2a + (10 – 1)d] = 235
⇒ 5[2a + 9d] = 235
⇒ 2a + 9d = 235/5 = 47
⇒ 2a + 9d = 47 …(iv)
Multiplying equation (iv) by 6 and subtracting
it from equation (iii), we get
(12a + 31d) – (12a + 54d) = 167 – 282
⇒ 23d = 115 ⇒ d = 5
Putting the value of d in equation (4), we get
2a + 9d = 47
⇒ 2a + 9(5) = 47
⇒ 2a + 45 = 47
⇒ 2a = 2 ⇒ a = 1
Sum of first 20 terms of this AP,
S₂₀ =20/2[2a + (20 – 1)d]
= 10[2(1) + 19(5)]
= 10[2 + 95]
= 10 × 97
= 970
Hence, the required sum of first 20 terms is 970.

Question. Find the:
(A) sum of those integers between 1 and 500 which are multiples of 2 as well as of 5. 
(B) sum of those integers from 1 to 500 which are multiples of 2 as well as of 5.
(C) sum of those integers from 1 to 500 which are multiples of 2 or 5.
[Hint (iii): These numbers will be: multiple of
2 + multiple of 5 – multiples of 2 as well as of 5]
Solution. (A) Multiples of 2 and 5 will be multiples of
LCM of 2 and 5. LCM of ( 2, 5) = 10
Multiples of 2 as well as 5 between 1 and
500 is 10, 20, 30, 40, …490
this forms an AP with first term, a= 10
Common difference, d = 20 – 10 = 10
Last term, L = 490
We know that sum of n terms between 1 and 500 is
S_n = n/2[a + L]
Also, L = a + (n – 1) d
⇒ 490 = 10 + (n – 1)10
⇒ 480 = (n – 1)10
⇒ (n – 1) = 48
⇒ n = 49
Putting this value in equation (i), we get
S₄₉ =49/2[10 + 490]
=49/2× 500 = 49/2× 250= 12250
⇒ S₄₉ = 12250
(B) Here, multiples of 2 as well as 5 from 1 to
500 are 10, 20, 30, …500
Here, first term, a = 10
common difference, d = 20 – 10 = 10
Last term, L = 500
We know that an = a + (n – 1)d
L = a + (n – 1)d
[Where, n is total no. of terms]
500 = 10 + (n – 1)d
490 = (n – 1)10
⇒ (n – 1) = 49 ⇒ n = 50
Also we know that Sn =n/2(a + L)
⇒ S₅₀ = 50/2(10 + 500)
= 25 × 510 = 12750
Hence, S50 = 12750
(C) Multiples of 2 or 5 = Multiples of 2 +
multiples of 5 – [Multiples of 2 and 5] …(i)
Multiples of 2 [2, 4, 6, …500]
Multiples of 5 [5, 10, 15, …500]
Multiples 2 and of 5 [10, 20, 30, …500]
1st list of multiples of 2 [2, 4, 6, …500]
Here, first term, a1 = 2
and common difference d₁ = 2
Let number of terms be n₁
Then, last term, L = a + (n₁ – 1)d
500 = 2 + (n₁ – 1)(2)
498 = (n₁ – 1)2
⇒ (n₁ – 1) = 249 ⇒ n₁ = 250
Sum of [2, 4, 6, … 500]
S_n,=n₁/2[a + l]
= 250/2[2 + 500]
S_n, = 225 × 502 = 62750
2nd list of multiples of 5 [5, 10, 15, …500]
Here, first term, a’ = 5,
common difference, d’ = 5
Last term, L’ = 500
Let n² be the number of terms of second
list. We know that an = a + (n – 1)d
L’ = a’ + (n₂ – 1) d’
500 = 5 + (n₂ – 1)5
⇒ 495 = (n2 – 1)5
⇒ n₂ – 1 = 99
⇒ n₂ = 100
Sum of 2nd List,
S_n =n/2(a + L)
S_n2 =n₂/2(5 + 500)
= 100/2×505= 25250
3rd list of multiples of 2 as well as 5
[10, 20, 30, …500 ]
Here, first term, a’’ = 10
Common difference, d’’ = 10
Last term , L’’ = 500
Let n3 be the number of terms, then
L’’ = a’’ + (n3 – 1) d’’
500 = 10 + (n3 – 1)(10)
490 = (n3 – 1)10
⇒ n₃ – 1 = 49
⇒ n₃ = 50
Sum of 3rd List,
Sn³ = n₃/2(10 + 500)
= 50/2×510 =12750
⇒ Sum of multiples of 2 or 5
= Sn₁+ Sn₂ – Sn₃
= 62750 + 25250 – 12750
= 88000 – 12750 = 75250

Question. Find the sum of the following series :
5 + (– 41) + 9 + (– 39) + 13 + (– 37) + 17 + …. + (– 5) + 81 + (– 3)
Solution. Given: series is:
5 + (– 41) + 9 + (– 39) + 13 + (– 37) + 17 +
(– 5) + 81 + (– 3)
= [5 + 9 + 13 + … + 81) + [(– 41) + (– 39) +
(– 37) + (– 5) + (– 3)]
Here are the two series:
1. 5 + 9 + 13 + …… + 81
2. (– 41) + (– 39) + (– 37) + …. + (– 3)
For the first series :
first term a = 5, common difference, d = 9 – 5
= 13 – 9 = 4
last term, l(an) = 81
Then, an = a + (n – 1)d [where ‘n’ is the number of terms
81 = 5 + (n – 1) × 4
⇒ (n – 1) = 76/4 = 19
⇒ n = 20
Sum of the first series, S20
S₂₀= 20/2[2 × 5 + (20 − 1) × 4 ]
= 10(10 + 19 × 4)
= 10(10 + 76)
= 860
For the second series :
first term, a′ = – 41,
common difference, d′ = (– 39) – (– 41)
= (– 37) – (– 39)
= 2
last term, l′(an) = – 3
Then, an′ = a′ + (n′ – 1)d′
[where n′ are the number of terms]
⇒ – 3 = – 41 + (n′ – 1) × 2
⇒ (n′ – 1) = 19
⇒ n′ = 20
Sum of the second series, S′20 =n'[2d’ + (n’ – 1)d’]
=20/2[2 × (− 4 1) + (19) × 2]
= 10[– 82 + 38]
= 10 × (– 44)
= – 440
Total sum = S₂₀ + S_′20
= 860 – 440 = 420
Hence, the total sum of series is 420.

Question. An AP consists of 37 terms. The sum of the 3 middle most terms is 225 and the sum of the last 3 is 429. Find the AP.
Solution. It is given that
Total number of terms, n = 37
Since n is odd, therefore
middle most term =(n + 1)/2 th term = 19th term 3 middle most terms = 18th, 19th and 20th term by given condition
a₁₈ + a₁₉ +a₂₀ = 225
We know that an = a + (n – 1) d
⇒ (a + 17d) + (a + 18d) + (a + 19d) = 225
⇒ 3a + 54d = 225
⇒ a + 18d = 75 …(i)
Also, it is given that sum of last 3 terms = 429
⇒ a₃₅ + a₃₆ + a₃₇ = 429
⇒ (a + 34d) + (a + 35d) + (a + 36d) = 429
⇒ 3a + 105d = 429
⇒ a + 35d = 143 …(ii)
Subtracting equation (i) from equation (ii), we get
(a + 35d) – (a + 18d) = 143 – 75
a + 35d – a – 18d = 68
⇒ 17d = 68 ⇒ d = 4
Putting value of d in equation (i), we get
a + 18(4) = 75
⇒ a + 72 = 75
⇒ a = 3, d = 4
Required AP is
a, a + d, a + 2d, a + 3d, …
3, 3 + 4, 3 + 2(4), 3 + 3(4), …
3, 7, 11, 15, …

Question. If the sum of the first ‘p’ terms of an A.P. is ‘q’ and sum of the first ‘q’ terms is ‘p’; then show that the sum of the first (p + q) terms is {(– (p + q)}.
Solution. Consider an A.P., whose first term be ‘a’ and common difference be ‘d’.
Given, Sp terms = q
Sq terms = p
To prove : Sp + q = – (p + q)
Proof : SP = p/2[2a + (p – 1)d] = q
or 2a + (p – 1)d =2q/p
and sq = q/2[2a + (q – 1)d] = p
or 2a + (q – 1)d = 2p/q
On subtracting equation (ii) from (i), we get
[(p – 1) – (q – 1)]d = 2q /2 -2p/p

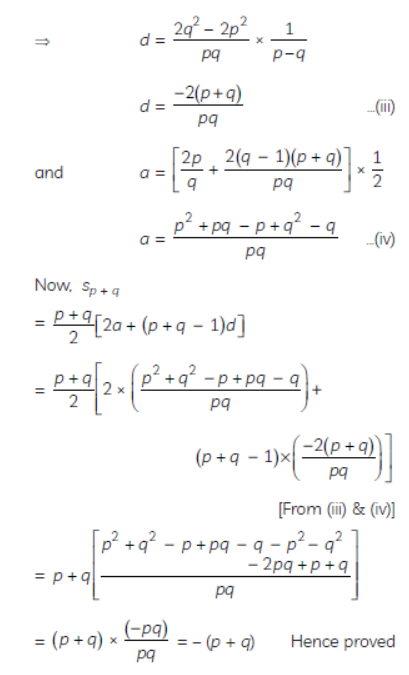
Question. Which term of the Arithmetic Progression – 7, – 12, – 17, – 22, … will be –82? Is –100 any term of the A.P.? Give reason for your answer.
Solution. The first term of the A.P., a = – 7
Common difference,
d = (– 12) – (– 7) = (–12 + 7)
= – 5
Let the nth term of A.P. be – 82.
Then, an = – 82
a + (n – 1) d = – 82
⇒ – 7 + (n – 1) (– 5) = – 82
⇒ – 7 – 5n + 5 = – 82
⇒ – 5n = – 80
⇒ n = 16
Let, mth term of the given A.P. be – 100
am = – 100
a + (m – 1) d = – 100
⇒ – 7 + (m – 1) (– 5) = – 100
⇒ (m – 1) 5 = 93
⇒ m =93/5+ 1=98/5∉ N
Therefore, – 100 is not any term of the given A.P.
Hence, – 82 is the 16th term of the A.P. and – 100 is not a term of the given A.P.

Question. How many terms of the arithmetic progression 45, 39, 33, … must be taken so that their sum is 180? Explain the double answer.
Solution. Given: The arithmetic progression is 45, 39, 33, ……
Here, a = 45
d = 39 – 45 = 33 – 39 = ……..
= – 6
and the sum the of nth term, Sn = 180
Sn =n/2[2a + (n – 1)d]
where, n is the number of terms.
180 =n/2[2 × 45 + (n – 1) (– 6)]
⇒ 360 = 96n – 6n2
⇒ 6n2 – 96n + 360 = 0
⇒ 6[n2 − 16n + 60] = 0
⇒ 6(n2 − 10n − 􀇩n + 60] = 0
⇒ 6(n(n(n − 10) − 6(n − 10)] = 0
⇒ 6[(n – 6) (n – 10)] = 0
⇒ n = 6, 10
Sum of a7, a8, a9 and a10 terms = 0
Hence, on either adding 6 terms or 10 terms we get a total of 180.

Question. Show that the sum of an AP whose 1st term is a, the 2nd turn is b and the last term c, is equal to
(a + c) (b + c -2a)/2(b – a)
Solution. It is given that
First term, a1 = a
Second term, a2 = b
Last term, L = c
Common difference = b – a
AP is a, b, …c
Let n be the number of terms of the given AP.
We know that an = a + (n – 1)d
⇒ L = a + (n – 1)(b – a)
⇒ c = a + (n – 1)(b – a)
⇒ (c – a) = (n – 1)(b – a)
⇒ (n – 1) = (c – )/(b – a)
⇒ n = c – a /b – a + 1 (c – a) +(b – a)/b – a
= c + b -2a/b – a
Now, we know that sum of an AP

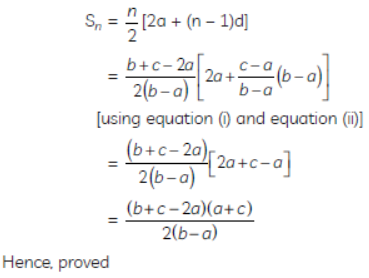
Question. If the sum of the first four terms of an AP is 40 and that of the first 14 terms is 280, find the sum of its first ‘n’ terms.
Solution. Let the first term of the A.P. be ‘a’ and common difference be ‘d’.
Then, sum of ‘n’ terms, Sn =n/2[2a + (n – 1)d]
where, ‘n’ is the number of terms.
According to the Question:
Given, S4 = 40
⇒ 4/2[2a + (4 – 1) × d)] = 40
⇒ 2a + 3d = 20
and S₁₄ = 280
⇒ 14/2 [2a + 13d] = 280
⇒ 2a + 13d = 40
On subtracting equation (i) from equation (ii), we get:

Put the value of ‘d’ in equation (i),
⇒ a =20 – 3 x 2/2 =7
Sum of n terms = n/2[2 × 7 + (n – 1) × 2]
= n/2[14 + 2 (n – 1)]
= n[7 + n – 1]
= n(n + 6) or n² + 6n
Hence, the sum of first ‘n’ terms is n(n + 6) or n² + 6n.

Question. Find the sum of the integers between 100 and 200 that are:
(A) divisible by 9
(B) not divisible by 9
Solution. (A) Integers between 100 and 200 that are divisible by 9 are 108, 117, 126, …198
Let n be the number of terms between 100 and 200
Here, first term, a = 108
Common difference, d = 117 – 108 = 9
Last term, L = 198
We know that an = a + (n – 1)d
L = a + (n – 1)d
⇒ 198 = 108 + (n – 1)9
⇒ 198 – 108 = (n – 1)9
⇒ 90 = (n – 1)9
⇒ (n – 1) = 10 ⇒ n = 11
Sum of n terms, Sn =n/2[2a + (n – 1)d]
∴ Sum of 11 terms between 100 and 200 which is divisible by 9 is
S_n =n/2[2a + (n – 1)d]
⇒ S₁₁ =11/2[2(108) + (11 – 1)(9)]
=11/2[2(108) + 10 × 9]
= 11 [108 + 5 × 9]
= 11 [108 + 45]
= 11× 153 = 1683
Hence, the required sum of integers is 1683.
(B) Sum of integers between 100 and 200 which is not divisible by 9 = sum of total no. between 100 and 200 –
sum of no. between 100 and 200 which are divisible by 9 …(i)
From part (i), we know that sum of no.
between 100 and 200 divisible by 9 = 1683
Total numbers between 100 and 200 is
101, 102, 103, … 199.
Here, first term, a = 101
Common difference, d = 102 – 101 = 1
Last term, L = 199
Let n be total no. of terms.
We know that an = a + (n – 1)d
⇒ L = a + (n – 1)d
199 = 101 + (n – 1)(1)
199 – 101 = (n – 1)
n = 99
∴ Sum of 99 terms between 100 and 200
S_n =n/2[2a + (n – 1)d]
S₉₉ =99/2[2(101) + (99 – 1)(1)]
=99/2[202 + 98
=99/2× 300
S₉₉ = 99 × 150 = 14850
Putting this value in equation (i), we get
sum of no. between 100 and 200 not divisible by 9
= 14850 – 1683
= 13167
Hence, the required sum is 13167.

Question. The sum of the 4^th and the 8^th terms of an AP is 24 and the sum of the 6th and the 10^th terms is 44. Find the sum of the first 10 terms of the AP.
Solution. Let the first term of A.P. be ‘a’and its common
difference be ‘d‘.
Given, a4 + a8 = 24
⇒ a + 3d + a + 7d = 24 [∵an = a + (n – 1)d]
⇒ 2a + 10d = 24
or a + 5d = 12 …(i)
and a6 + a10 = 44 (given)
⇒ a + 5d + a + 9d = 44
⇒ 2a + 14d = 44
or a + 7d = 22 …(ii)
on subtracting eduation (i) from (ii), we get

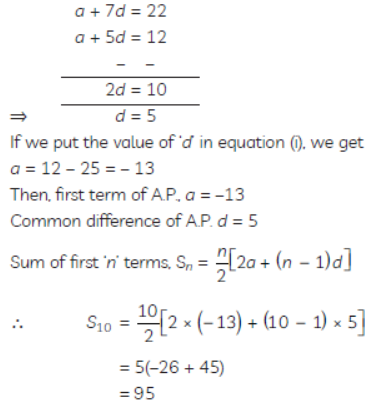
Question. If the ratio of the 11th term of an AP to its 18th term is 2 : 3, find the ratio of the sum of the first five terms to the sum of its first 10 terms. 
Solution. Let, the first term of A.P. be ‘a’ and its common
difference be ‘d’.
Then, 11th term of A.P., a11 = a + 10d
18th term of A.P., a18 = a + 17d
a +10d /a + 17d 2/3
⇒ 3(a + 10d) = 2(a + 7d)
⇒ 3a + 30d = 2a + 34d
⇒ a = 4d …(i)
Now, sum of first five terms,
S5 = n/2[2a + (n – 1)d]
=5/2[2 × 4d + 4 × d] [from (i)]
=5/2× 12d = 30d
Sum of first 10 terms,
S10 = n/2[2a + (n – 1)d]
=10/2[2 × 4d + 9 × d]
= 5(8d + 9d)
= 85d
S5/S10 = 30d/85d = 6/17
Hence, the required ratio is 6 : 17.

Question. A thief runs with a uniform speed of 100 m/ minute. After one minute a policeman after the thief to catch him. He goes with a speed of 100 m/minute in the first minute and increases his speed by 10 m/minute every succeeding minute. After how many minutes the policeman will catch the thief? 
Solution. Let, the total time to catch the thief be ‘n’ minutes.
speed of thief = 100 m/min.
Total distance covered by the thief = (100 n)
Here, the speed of the policeman in the first minute is 100 m/min, in the 2nd minute is 110 m/min, in the 3rd minute is 120 m/min and so on.
Then, the speed forms an A.P. with a constant
increasing speed of 10 m/min. thus, the series is :
100, 110, 120, 130
As the policeman starts after a minute (n – 1) Total distance covered by the policeman
= 100 + 110 + 120 + … (n – 1) terms
100n = n – 1/2 [200 + (n – 2) × 10
[∴sn = n/2 [2a + (n- 1)]d]
⇒ 200n = n – 1[200 + 10n – 20]
⇒ 200n = (n – 1) (180 + 10n)
⇒ 200n = 180n – 180 + 10n2 – 10n
⇒ 10n² – 30n – 180 = 0
⇒ n² – 3n – 18 = 0
⇒ (n – 6) (n + 3) = 0
⇒ n = 6 [∵ n = – 3, is not possible]
Hence, the police’man takes (n – 1) = 5 minutes to catch the theif.

Question. If the ratio of the sum of the first n terms of two A.Ps is (7n + 1) : (4n + 27), then find the ratio of their 9^th terms.
Solution.

Question. The ratio of the sums of first m and first n terms of an A.P. is m² : n². Show that the ratio of its mth and nth terms is (2m – 1):(2n – 1).
Solution. Let, ‘a’ be the first term an A.P. and ‘d’ be the common difference.
Let, Sm and Sn be the sum of the first ‘m’ and first ‘n’ of terms of the A.P. respectively.

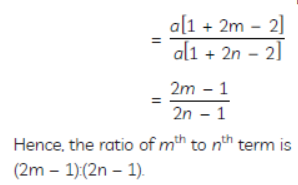
Question. Solve the equation –4 + (–1) + 2 + … + x = 437.
Solution. The given equation is –4 + (–1) + 2 + … + x = 437
The given equation is A.P.
with first term, a = –4
and common difference,
d = (–1) – (–4) = –1 + 4 = 3
Last term, L = x
We know that an = a + (n – 1)d
⇒ L = x = a + (n – 1)(d)
⇒ x = –4 + (n – 1)(3)
⇒ x = –4 + 3n – 3
⇒ n = x + 7/3
Also, we know that
S_n =n/2[2a + (n – 1)d]

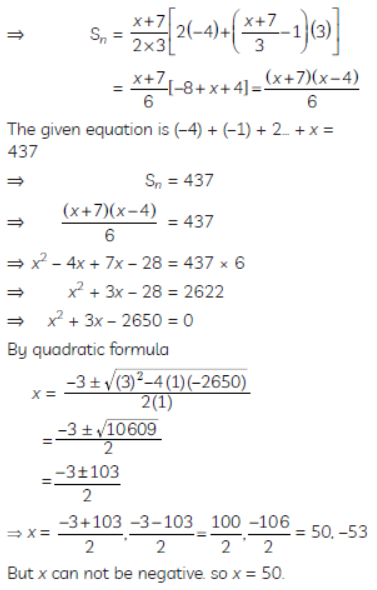
Mathematics Arithmetic Progression Worksheets for Class 10 as per CBSE NCERT pattern

Parents and students are welcome to download as many worksheets as they want as we have provided all free. As you can see we have covered all topics which are there in your Class 10 Mathematics Arithmetic Progression book designed as per CBSE, NCERT and KVS syllabus and examination pattern. These test papers have been used in various schools and have helped students to practice and improve their grades in school and have also helped them to appear in other school level exams. You can take printout of these chapter wise test sheets having questions relating to each topic and practice them daily so that you can thoroughly understand each concept and get better marks. As Mathematics Arithmetic Progression for Class 10 is a very scoring subject, if you download and do these questions and answers on daily basis, this will help you to become master in this subject.

Benefits of Free Arithmetic Progression Class 10 Worksheet

1. You can improve understanding of your concepts if you solve NCERT Class 10 Mathematics Arithmetic Progression Worksheet,
2. These CBSE Class 10 Mathematics Arithmetic Progression worksheets can help you to understand the pattern of questions expected in Mathematics Arithmetic Progression exams.
3. All worksheets for Mathematics Arithmetic Progression Class 10 for NCERT have been organized in a manner to allow easy download in PDF format
4. Parents will be easily able to understand the worksheets and give them to kids to solve
5. Will help you to quickly revise all chapters of Class 10 Mathematics Arithmetic Progression textbook
6. CBSE Class 10 Mathematics Arithmetic Progression Workbook will surely help to improve knowledge of this subject

These Printable practice worksheets are available for free download for Class 10 Mathematics Arithmetic Progression. As the teachers have done extensive research for all topics and have then made these worksheets for you so that you can use them for your benefit and have also provided to you for each chapter in your ebook. The Chapter wise question bank and revision worksheets can be accessed free and anywhere. Go ahead and click on the links above to download free CBSE Class 10 Mathematics Arithmetic Progression Worksheets PDF.