Polynomials Class 10 Worksheet have been designed as per the latest pattern for CBSE, NCERT and KVS for Grade 10. Students are always suggested to solve printable worksheets for Mathematics Polynomials Grade 10 as they can be really helpful to clear their concepts and improve problem solving skills. We at worksheetsbag.com have provided here free PDF worksheets for students in standard 10 so that you can easily take print of these test sheets and use them daily for practice. All worksheets are easy to download and have been designed by teachers of Class 10 for benefit of students and is available for free download.
Mathematics Polynomials Worksheets for Class 10
We have provided chapter-wise worksheets for class 10 Mathematics Polynomials which the students can download in Pdf format for free. This is the best collection of Mathematics Polynomials standard 10th worksheets with important questions and answers for each grade 10th Mathematics Polynomials chapter so that the students are able to properly practice and gain more marks in Class 10 Mathematics Polynomials class tests and exams.
Chapter-wise Class 10 Mathematics Polynomials Worksheets Pdf Download
PRACTICE EXERCISE
Question. Draw the graph of each of the following polynomials and if possible, read the zero(s) from the graph :
(a) x2 – 2x + 9 (b) –2x2 + 4x
(c) x2 + 2x – 3 (d) x2 – 8x + 16
(e) x3 (f) x3 – 2x2
Solution. (a) No zero (b) 0 and 2 (c) –3 and 1 (d) 4 (e) 0 (f) 0 and 2
Question. Draw the graph of the polynomial x2 – 3x – 10. Read off the zeroes of the polynomial from the graph. Also show the axis of symmetry on it.
Solution. zeroes 5 and –2 ; Axis of symmetry; x = 3/2
Question. Find the zeroes of each of the following quadratic polynomial. Also, in each case, verify the relationship between the zeroes and its coefficients :
(a) x2 + 8x + 12 (b) x2 + 3x – 4
(c) x2 – 7x + 10 (d) y2 – 4
(e) 4u2 – 1 (f) 3t2 – 5
Solution. (a) –2 and –6 (b) 1 and –4 (c) 2 and 5 (d) ± 2 (e) ± 1/2 (f) ±√5/3
Question. On dividing x3 – 3x2 + x + 2 by a polynomial g(x), the quotient and remainder are (x – 2) and (–2 x + 4) respectively. Find g(x).
Solution. x2 – x + 1
Question. Find a quadratic polynomial whose sum and product of the zeroes are 3 and –5 respectively.
Solution. x2 – 3x – 5
Question. If the zeroes of the polynomial x3 – 3x2 + x + 1 are a – b, a, a + b, find a and b.
Solution. a = 1, b = ± √2
Question. Divide p(x) = 9x4 – 4x2 + 4 by q(x) = 3x2 + x – 1. Also, find quotient and remainder.
Solution. quotient = 3x2 – x, remainder = – x + 4
Question. Find zeroes of f (x)= x2 + 5x – 14. Also, verify the relationship between zeroes and its coefficients.
Solution. 2 and – 7
Question. Find all the zeroes of the polynomial f(x) = 2x4 – 3x3 – 3x2 + 6x – 2, if its two zeroes are – √2 and √2 .
Solution. – √2 , √2 , 1 , 1/2
Question. The graph of y = p(x) are given below. find the number of zeroes of p(x).
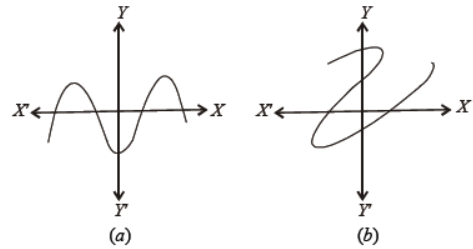
Solution. (a) 4 (b) 2
Question. Find a quadratic polynomial each with the given numbers as the sum and the product of its zeroes respectively :
(a) 3 and 4 (b) –2 and 3/ 2
(c) – 3/2 and 0 (d) – √2 and √3
Solution. (a) x2 – 3x + 4 (b) x2 + 2x + 3/2 (c) x2 + 3/2 x (d) x2 + √2x + √3
Question. If the polynomial x4 – 6x3 + 16x2 – 26x + 10 – a is divided by another polynomial x2 – 2x + k, the remainder comes out to be x + a. Find k and a.
Solution. k = 5, a = – 5
Question. Obtain all the zeroes of the polynomial 2x4 – 2x3 – 7x2 + 3x + 6 ; if two of its zeroes are ± √3/2 .
Solution. 2, –1
Question. Find a cubic polynomial with the sum, sum of product of its zeroes taken two at a time and the product of its zeroes respectively as given below :
(a) –4, 7 and 0 (b) 5, –2 and – 24
(c) – 2, – 8/3 and 0
Solution. (a) x3 + 4x2 – 7 (b) x3 – 5x2 – 2x + 24 (c) 3x3 – 6x + 8
Question. Without drawing actual graph, find the zeroes of the polynomials if any. Give reason.
(a) x2 – 2x– 8 (b) –x2 – 2x + 3
(c) x2 + x + 1 (d) x2 – 1
(e) x2 + 4x + 4 (f) –4x2+ 4x – 1
Solution. (a) –2 and 4 (b) –3 and 1 (c) no zero (d) 1 and –1 (e) –2 and –2 (f) 1/2 and 1/2
Question. The graphs of y = p(x) are given below, for some polynomials p(x). Find the number of zeroes of p(x), in each case.

Solution. (a) 1 (b) 1 (c) 2 (d) 2 (e) 1 (f) 1
Question. Write a cubic polynomial with zeroes : – 2, 1, 4 and hence find :
(a) sum of its zeroes (b) sum of its zeroes taking any two at a time
(c) product of three zeroes
Solution. x3 – 3x2 – 6x + 8; (a) 3 (b) –6 (c) –8
Question. The graph of y = p(x) are given below, for some polynomial p(x). Find the zeroes of the corresponding polynomial.
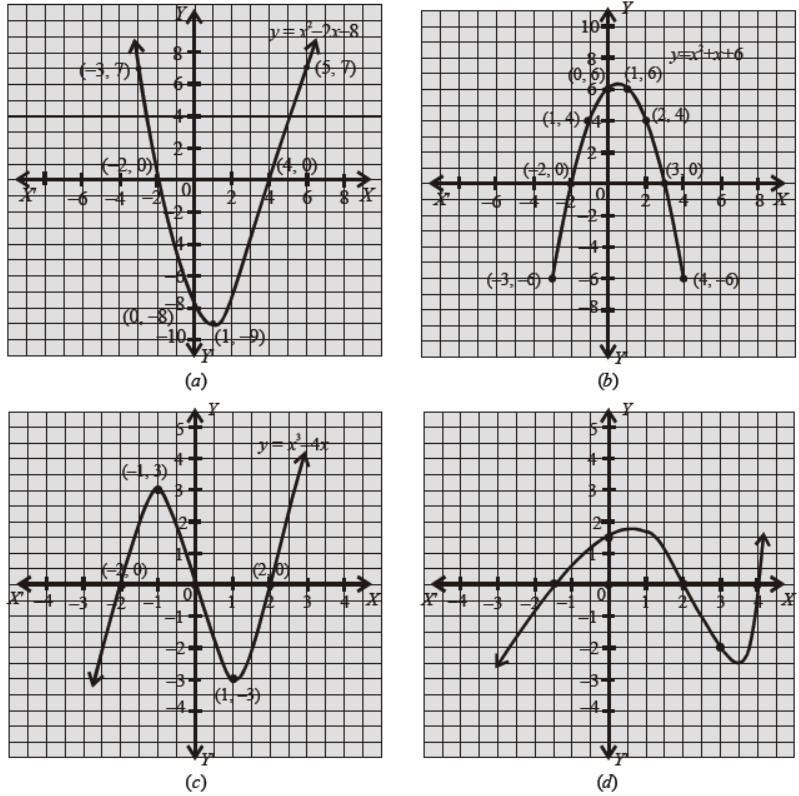
Solution. (a) –2, 4 (b) –2, 3 (c) –2, 0, 2 (d) –1.5, 2, 4
Question. Check whether the first polynomial is a factor of the second polynomial by applying the division algorithm :
(a) x + 8; x3 + 15 x2 + 56x (b) x – 2; x4 – 3x3 + 3x – 9
(c) x2 – 2; x3 – 3x2 + 5x – 3 (d) – 5y2 – 4y + 2; 15y4 + 2y3 – 39y2 – 16y + 10
(e) 2x2 + 3x + 4 ; 2x4 + 7x3 + 10x2 + 14x + 8 (f) x3 – 3x + 1; x5 – 4x3 + x2 + 3x + 1
Solution. (a) yes (b) yes (c) no (d) yes (e) no (f) no
Question. What must be added to the polynomial p(x) = x4 + 2x3 – 2x2 + x – 1 so that the resulting polynomial is exactly divisible by x2 + 2x – 3?
Solution. x – 2
Question. Find the value of a and b so that 1, –2 are the zeroes of the polynomial x3 + 10x2 + ax + b.
Solution. a = 7, b = – 18
Question. On dividing x3 – 3x2 + 5x – 3 by a polynomial g(x), the quotient and remainder are x – 3 and 7x – 9 respectively. Find g(x).
Solution. x2 – 2
Question. Obtain all the zeroes of the polynomial f(x) = x4 – 3x3 – x2 + 9x – 6; if two of its zeroes are √3 and – √3 .
Solution. – √3, √3, 1, 2
Question. On dividing x4 – 5x + 6 by a polynomial g(x), the quotient and remainder are –x2 – 2 and –5x + 10 respectively. Find g(x).
Solution. – x2 + 2
Question. Apply the division algorithm to find the quotient and the remainder on division of p(x) by g(x) as given below :
(a) p(x) = –5x2 + 14x3 + 9x – 1, g(x) = –1 + 2x (b) p(x) = 6x3 + 11x2 – 39x – 65, g(x) = x2 + x – 1
(c) p(x) = x4 – 5x + 6, g(x) = 2 – x2 (d) p(x)= 3x3 + x2+ 2x + 5, g(x) = 1 + 2x + x2
(e) p(x) = 4x3 – 27x + 4x2 + 16, g(x) = 2x – 3 (f) p(x) = 6x3 + 19x2 + 18x – 5, g(x) = 3x + 5
(g) p(x) = –2x4 – 12x3 – 22x2 – 17x + 4, g(x) = x2 – 3x + 4
(h) p(x) = 6x5 + 4x4 – 3x3 + x + 1, g(x) = 3x2 – x + 1
Solution. (a) quotient = 7x2 + x + 5, remainder = 4
(b) quotient = 6x + 5, remainder = – 38x – 60
(c) quotient = – x2 – 2, remainder = – 5x + 10
(d) quotient = 3x – 5, remainder = 9x + 10
(e) quotient = 2x2 + 5x – 6, remainder = – 2
(f) quotient = 2x2 + 3x + 1, remainder = – 10
(g) quotient = – 2x2 – 9x – 49, remainder = 130 x + 200
(h) quotient = 2x3 + 2x2 – x – 1, remainder = x + 2
Question. What must be subtracted from 8x4 + 14x3 – 2x2 + 7x – 8 so that the resulting polynomial is exactly divisible by 4x2 + 3x – 2?
Solution. 14 x – 10
Question. If the polynomial f (x) = x 4 – 6x3 +16x2 – 25x +10 is divided by another polynomial x2 – 2x + k, the remainder comes out to be x + a. Find k and a.
Sol. By di vi sion al go rithm, we have
Dividend = Divisor ´ Quotient + Remainder
⇒ Dividend – Remainder = Divisor x Quotient
⇒ Dividend – Remainder is always divisible by the divisor.
When f (x) = x 4 – 6x3 +16x2 – 25x +10 is divided by x 2 – 2x + k the remainder comes out to be x + a .
∴ f (x) – (x + a) = x4 – 6x3 +16x2 – 25x +10 -(x + a)
= x 4 – 6x3 +16x2 – 25x +10 – x – a
= x 4 – 6x3 +16x2 – 26x +10 – a
is exactly divisible byx2 – 2x + k.
Let us now divide x 4 – 6x3 +16x2 – 26x +10 – a byx2 – 2x + k.

For f (x) – (x + a) = x4 – 6x3 +16x 2 – 26x +10 – a to be exactly divisible by x2 – 2x + k, we must have
(-10 + 2k) x + (10 – a – 8k + k2) = 0 for all x
⇒ -10 + 2k = 0 and 10 – a – 8k + k2 = 0
⇒ k = 5 and 10 – a – 40 + 25 = 0
⇒ k = 5 and a = – 5.
Answer
(i) Zeroes are – √2 – 1/2, (ii) g(x) = -4x2 -3x +6
Question. What must be subtracted from p(x) = 8x 4 +14x 3 – 2x 2 + 7x – 8 so that the resulting polynomial is exactly divisible by g(x) = 4x 2 + 3x – 2?
Sol. Let y be sub tracted from polynomial p (x)
∴ p(x) = 8x4 +14x3 – 2x2 +7x – 8 – y
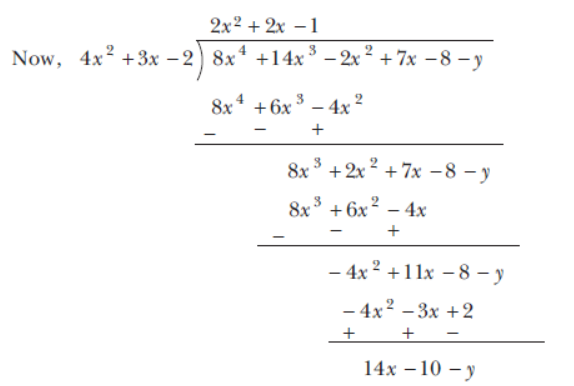
Q Remainder should be 0.
∴ 14x -10 – y = 0
or 14x -10 = y or y =14x -10
∴ (14x -10) should be subtracted from p(x) so that it will be exactly divisible by g(x).
Question. (i) If the remainder on division of x 3 -2x2 + kx +5 by x -2 is 11, find the quotient and the value of k. Hence, find the zeroes of the cubic polynomial x3 -2x2 + kx -6.
(ii) Given that the zeroes of the cubic polynomial x 3 -6x2 +3x +10 are of the form a, a + b, a +2b for some real numbers a and b, find the values of a and b as well as the zeroes of the given polynomial.
Answer
(i) k = 3, Quotient = x2 +3, 2 is the zero of x 3 -2x2 +3x-6
(ii) When a = 5, b = -3 and when a = -1, b = 3, zeroes are –1, 2, 5
Question State whether the following statements are true or false. Justify your answer.
(i) If the zeroes of a quadratic polynomial ax 2 + bx + c are both positive, then a, b and c all have the same sign.
(ii) The quotient and remainder on division of 2x2 +3x + 4by x 3 +1 are 0 and 2x2 +3x + 4 respectively.
Answer
(i) False (ii) True
Question. Divide the polynomial f(x) = x4 – 3x2 + 4x + 5 by the polynomial 1 – x + x2 and verify the division algorithm.
Solution. On writing the dividend and the divisor in the standard form, we get :
Dividend p(x) = x4 – 3x2 + 4x + 5 and divisor g(x) = x2 – x + 1.
Now,
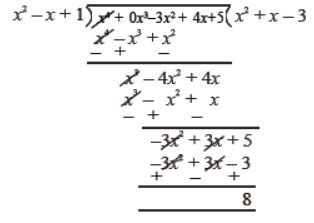
Clearly, quotient = x2 + x – 3 and remainder = 8.
Question. Find the zeroes of the quadratic polynomial x2 – 2x – 8, and verify the relationship between the zeroes and their coefficients.
Solution. Let f(x) = x2 – 2x – 8
The zeroes of f(x) are given by f(x) = 0.
⇒ x2 – 2x – 8 = 0
⇒ x2 – 4x + 2x – 8 = 0
⇒ x (x – 4) + 2 (x – 4) = 0
⇒ (x + 2) (x – 4) = 0
⇒ x = – 2 or x = 4 Ans.
Question. Obtain all the zeroes of the polynomial f (x) = 3x4 + 6x3 – 2x2 – 10x – 5, if two of its zeroes are

Solution.
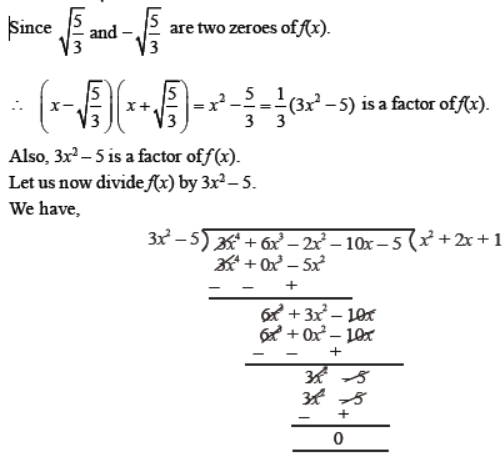
Question. Find a quadratic polynomial whose sum and product of the zeroes is 1/4 and –1 respectively .
Solution. We know that a quadratic polynomial when the sum and product of its zeroes are given by f(x) = k {x2 – (sum of the zeroes) x + Product of the zeroes}, where k is a constant.
∴ Required quadratic polynomial f(x) is given by f (x) = k (x2 – 1/4 , x-1)
OR
Let the required quadratic polynomial be ax2 + bx + c.
Since, sum of zeroes = -b/a ⇒ 1/4 = -b/a
and, product of zeroes = c/a ⇒ -1 = c/a ⇒ -4/4 = c/a
If a = 4; then b = 1 and c = – 4.
∴ Required quadratic polynomial = ax2 + bx + c
= 4x2 – x – 4
Question. Draw the graph of the polynomial f(x) = x2 – 2x – 3. Obtain the vertex of this parabola. Also, read the zeroes of the polynomial, if possible from the graph.
Solution. Let y = f(x) = x2 – 2x – 3.
The following table gives the values of y for various values of x.

After plotting the points in the table on a graph paper, draw a free-hand continuous curve (parabola) through all the points plotted.

Vertex of Parabola : On comparing the polynomial x2 – 2x – 3 with ax2 + bx + c, we get a = 1,
b = –2, c = – 3. The vertex of the parabola has the co-ordinates (-b/2a , – D/4a) , where D = b2 – 4ac.
Now, D = b2 – 4ac = (–2)2 – 4 (1) (–3)= 4 + 12 = 16
∴ Co-ordinate of vertex is [-(-2)/2(1) ,-16/4(1)] = (1,4)
Zeroes of f(x) = x2 – 2x – 3
Since the parabola f(x) = x2 – 2x – 3 cuts the x-axis at (–1, 0) and (3, 0).
∴ zeroes of f(x) are –1 and 3.
Mathematics Polynomials Worksheets for Class 10 as per CBSE NCERT pattern
Parents and students are welcome to download as many worksheets as they want as we have provided all free. As you can see we have covered all topics which are there in your Class 10 Mathematics Polynomials book designed as per CBSE, NCERT and KVS syllabus and examination pattern. These test papers have been used in various schools and have helped students to practice and improve their grades in school and have also helped them to appear in other school level exams. You can take printout of these chapter wise test sheets having questions relating to each topic and practice them daily so that you can thoroughly understand each concept and get better marks. As Mathematics Polynomials for Class 10 is a very scoring subject, if you download and do these questions and answers on daily basis, this will help you to become master in this subject.
Benefits of Free Polynomials Class 10 Worksheet
- You can improve understanding of your concepts if you solve NCERT Class 10 Mathematics Polynomials Worksheet,
- These CBSE Class 10 Mathematics Polynomials worksheets can help you to understand the pattern of questions expected in Mathematics Polynomials exams.
- All worksheets for Mathematics Polynomials Class 10 for NCERT have been organized in a manner to allow easy download in PDF format
- Parents will be easily able to understand the worksheets and give them to kids to solve
- Will help you to quickly revise all chapters of Class 10 Mathematics Polynomials textbook
- CBSE Class 10 Mathematics Polynomials Workbook will surely help to improve knowledge of this subject
These Printable practice worksheets are available for free download for Class 10 Mathematics Polynomials. As the teachers have done extensive research for all topics and have then made these worksheets for you so that you can use them for your benefit and have also provided to you for each chapter in your ebook. The Chapter wise question bank and revision worksheets can be accessed free and anywhere. Go ahead and click on the links above to download free CBSE Class 10 Mathematics Polynomials Worksheets PDF.

You can download free worksheets for Class 10 Mathematics Polynomials from https://worksheetsbag.com
You can get free PDF downloadable worksheets for Grade 10 Mathematics Polynomials from our website which has been developed by teachers after doing extensive research in each topic.
On our website we have provided worksheets for all subjects in Grade 10, all topic wise test sheets have been provided in a logical manner so that you can scroll through the topics and download the worksheet that you want.
You can easily get question banks, topic wise notes and questions and other useful study material from https://worksheetsbag.com without any charge
Yes all test papers for Mathematics Polynomials Class 10 are available for free, no charge has been put so that the students can benefit from it. And offcourse all is available for download in PDF format and with a single click you can download all worksheets.
https://worksheetsbag.com is the best portal to download all worksheets for all classes without any charges.